High School: Geometry

More Specific Topics in High School: Geometry

Popular Tutorials in High School: Geometry

What are Similar Figures?

Looking at two figures that are the same shape and have the same angle measurements? You have similar figures! Learn all about it in this tutorial!
What are Corresponding Parts in Similar Figures?

Identifying corresponding parts in similar figures isn't so bad, but you have to know what you're looking for. This tutorial does a great job of explaining the corresponding parts of similar figures! Take a look!
How Do You Write an Equation of a Line in Slope-Intercept Form If You Have One Point and a Parallel Line?

Want to find the slope-intercept form of a line when you're given a point on that line and another line parallel to that line? Remember, parallel lines have the same slope. If you can find the slope of that parallel line, you'll have the slope of your line! In this tutorial, you'll see how to find the slope of your line and use that slope, along with the given point, to write an equation for the line in slope-intercept form. Take a look!
How Do You Write an Equation of a Line in Slope-Intercept Form If You Have One Point and a Perpendicular Line?

Want to find the slope-intercept form of a line when you're given a point on that line and another line perpendicular to that line? Remember, perpendicular lines have slopes that are opposite reciprocals of each other. In this tutorial, you'll see how to find the slope using the slope of the perpendicular line. Then, use this slope and the given point to write an equation for the line in slope-intercept form. Check it out!
How Do You Find the Slope of a Line If You Have a Perpendicular Line?

Perpendicular lines have slopes that are opposite reciprocals of each other. To find the slope of a line that is perpendicular to a given equation, find the opposite reciprocal of that slope. Check out this tutorial to learn how!
How Do You Know if Two Lines are Parallel?

Parallel lines are lines that will go on and on forever without ever intersecting. This is because they have the same slope! If you have two linear equations that have the same slope but different y-intercepts, then those lines are parallel to one another!
How Do You Know if Two Lines Are Perpendicular?

Perpendicular lines intersect at right angles to one another. To figure out if two equations are perpendicular, take a look at their slopes. The slopes of perpendicular lines are opposite reciprocals of each other. Their product is -1! Watch this tutorial and see how to determine if two equations are perpendicular.
How Do You Find the Distance Between Two Points?

Trying to find the distance between two points? Use the distance formula! Want to see how it's done? Check out this tutorial!
What is the Pythagorean Theorem?

The Pythagorean theorem is a very popular theorem that shows a special relationship between the sides of a right triangle. In this tutorial, you'll get introduced to the Pythagorean theorem and see how it's used to solve for a missing length on a right triangle!
How Do You Use Matrices to Translate a Figure?

Matrices can help you solve all sorts of problems! In this tutorial, you'll see how to use a translation matrix to translate a figure on the coordinate plane.
How Do You Write an Equation for an Ellipse Centered at the Origin Given a Vertex and a Co-Vertex?
What are Conic Sections?
What is a Parabola?

A parabola is much more than just the graph of a quadratic. This tutorial shows you various definitions of a parabola and even shows you horizontal parabolas! Take a look!
What is an Ellipse?

An ellipse is a specific shape with some very neat pieces! In this tutorial, you'll learn multiple definitions of an ellipse and see all its different parts.
What is the Standard Form of the Equation of a Horizontal Ellipse?
What is a Hyperbola?

This tutorial explains how hyperbolas are created. It also shows you the different parts of hyperbolas and defines them multiple ways. Follow along with this tutorial to learn all about hyperbolas!
What is the Sine Ratio?

The sine ratio is a handy ratio when you're dealing with right triangles! In this tutorial, you'll learn what the sine ratio is and how to use it to find angle measurements in a right triangle.
What is the Tangent Ratio?

There are several different ratios you can make from the sides of a right triangle. One of them is the tangent ratio. Watch this tutorial to add the tangent ratio to your right triangle tool box!
What is the Secant Ratio?
What is the Law of Sines?

Want to find a missing side or angle measurement of a triangle? The Law of Sines can help! Follow along to learn about this handy proportion.
What is the Formula for the Area of a Triangle Using Sines?

You can use sine to help you find the area of a triangle! All you need is two sides and an angle measurement! This tutorial helps you find this formula.
What is the Law of Cosines?
What are the Trigonometric Cofunction Identities?
How Do You Bisect a Segment by Constructing?
How Do You Construct a Line Parallel to Another Line Through a Given Point?

A compass is a very handy tool! In this tutorial, you'll see how to use a compass and straightedge to construct parallel lines!
What is the Corresponding Angles Postulate?

When a transversal intersects parallel lines, the corresponding angles created have a special relationship. The corresponding angles postulate looks at that relationship! Follow along with this tutorial to learn about this postulate.
What is the Converse of the Corresponding Angles Postulate?

The corresponding angles postulate states that when a transversal intersects parallel lines, the corresponding angles are congruent. What if you go the other way and start with corresponding angles that are congruent? Is the converse of this postulate true? This tutorial explores exactly that!
How Do You Determine if Triangles on the Coordinate Plane are Congruent?

There are many ways to show that two triangles are congruent. This tutorial shows you how to use a triangle congruence postulate to show that two triangles on the coordinate plane are congruent to each other!
How Do You Use a Congruence Postulate to Prove Triangles are Congruent?

When proving two triangles are congruent, you use information and postulates you already know to create a logical trail from what you know to what you want to show. This tutorial shows an example of using a congruence postulate to show two triangles are congruent!
How Do You Show that Corresponding Parts of Congruent Triangles are Congruent?

If you're given information about two triangles and asked to prove parts of the triangles are congruent, see if you can show the two triangles are congruent. If they are, then you know that the corresponding parts are congruent! Follow along with this tutorial to see an example.
How Do You Prove that Two Overlapping Triangles are Congruent?

Proofs are an important part of geometry. This tutorial shows you how to use given information to prove that two overlapping triangles are congruent!
How Do You Identify Common Parts in Overlapping Triangles?

It might seem like a challenge to make sense of figures with overlapping triangles, but it's not so difficult! This video gives some commonsense advice for identifying common angles and common sides in these figures.
How Do You Construct an Equilateral Triangle?

This tutorial shows you how to construct an equilateral triangle using a ruler and compass.
What is the Side-Angle-Side Postulate for Triangle Congruence?

The Side-Angle-Side postulate is just one of many postulates you can use to show two triangles are congruent. This tutorial introduces you to the SAS postulate and shows you how to use it!
What is the Angle-Side-Angle Postulate for Triangle Congruence?

What is the Angle-Side-Angle postulate? This postulate is just one of many postulates you can use to prove two triangles are congruent! This tutorial explains the ASA postulate.
What is CPCTC?

The term CPCTC can come up a lot when you're dealing with congruent triangles, but what does it mean? This tutorial gives a great explanation and shows you how to use it in an example!
What is the Hypotenuse-Leg Congruence Theorem?

There's a special theorem that helps you quickly figure out if two right triangles are congruent. This tutorial introduces you to that theorem and shows you how to use it!
How Can You Tell if a Point is on the Perpendicular Bisector of a Line Segment?

If you want to determine if a point is on the perpendicular bisector of a line segment, the Perpendicular Bisector Theorem and its converse might come in handy. This tutorial gives a great example of how to tell if a given point is a perpendicular bisector of a segment!
How Do You Use the Centroid to Find Segment Lengths in a Triangle?

When you're given the centroid of a triangle and a few measurements of that triangle, you can use that information to find missing measurements in the triangle! This tutorial shows you how it's done.
How Do You Use the Hinge Theorem to Compare Side Lengths in Two Triangles?

The Hinge Theorem helps you compare side measurements of two triangles when you have two sets of congruent sides. Follow along with this tutorial to see this theorem used to find the relationship between the sides of two triangles.
How Do You Construct a Perpendicular Bisector?

This tutorial shows you how to construct a perpendicular bisector using only a compass and a straightedge!
How Do You Write an Indirect Proof?

This tutorial looks at using an indirect proof to show that an equilateral triangle cannot have a right angle.
How Do You Put the Sides of a Triangle in Order According to Size if You Know Two Angles of the Triangle?

Trying to figure out which side of a trianlge is the shortest? How about the longest? All you need to get your answer are the angle measurements of the triangle! How does it work? Watch this tutorial to find out!
What is the Circumcenter of a Triangle?

This tutorial explains the ins and outs of the circumcenter of a triangle. You'll see how to build up from the Perpendicular Bisector Theorem to find the circumcenter of a triangle.
What is the Incenter of a Triangle?

The incenter of a triangle deals with the angle bisectors of a triangle. This tutorial shows you how to find the incenter of a triangle by first finding the angle bisectors.
What is the Hinge Theorem?

What's the Hinge Theorem all about? Watch this tutorial to find out!
What is a Perpendicular Bisector?

Looking at the term 'perpendicular bisector', you can bet it has something to do with perpendicular lines! This tutorial talks all about perpendicular bisectors and shows you exactly what the term means.
What is the Triangle Midsegment Theorem?

The triangle midsegment theorem looks at the relationship between a midsegment of a triangle and the triangle's third side. Follow along with this tutorial to learn about the triangle midsegment theorem.
What is a Median of a Triangle?

A median of a triangle is a special line segment that connects two pieces of a triangle. This tutorial introduces you to the median of a triangle and shows you how many medians each triangle has!
How Do You Find Values for Variables in the Angles of a Quadrilateral To Make it a Parallelogram?

When you're given a quadrilateral with some of the interior angles defined with variables, you can find what values those variables need to have to make that quadrilateral a parallelogram. Follow along with this tutorial to learn what steps to take to get the answer!
How Do You Use the Diagonals of a Rectangle to Find the Value of a Variable?

When you make diagonals inside a rectangle, those diagonals are congruent. With this information, you can find the value of a variable that's part of a measurement of a diagonal. This tutorial shows you the steps.
How Do You Use Variables to Name Coordinates for a Figure Placed on the Coordinate Plane?

This tutorial shows you how to find the vertices of a rhombus that's graphed on the coordinate plane!
How Do You Write a Coordinate Proof?

In this tutorial, you'll see how to write a coordinate proof to prove that two triangles are congruent. Check it out!
How Do You Find the Value for a Variable in the Angles of a Quadrilateral To Make it a Rhombus?

A parallelogram needs to have certain qualities in order to be a rhombus. In this tutorial, you'll use your knowledge of these shapes in order to find the value for a variable that will make a given parallelogram a rhombus.
How Do You Construct a Square?

Did you know you don't need a ruler to construct a square? All you need is a compass and a straightedge! Watch this tutorial to see how it's done!
How Do You Use the Triangle Proportionality Theorem to Find Missing Lengths in a Diagram?

This tutorial shows you how the Triangle Proportionality Theorem can be used to find a missing length in a diagram. Take a look!
How Do You Graph a Glide Reflection?

When you graph a composition of two transformations, you have to be very careful to perform all the steps in the right order! Watch this tutorial to see how to graph a glide reflection.
How Do You Identify a Congruence Transformation?

Want to figure out whether two figures are congruent? There's a mathematically precise way to do this! Watch this tutorial on congruence transformations to learn more.
How Do You Identify a Similarity Transformation?

How do you know if one figure is similar to another? If you can find a similarity transformation that maps one figure to the other, then the figures are similar! Learn more about spotting similarity transformations with this tutorial.
What is a Congruence Transformation, or Isometry?

Not all transformations are created equal! Congruence transformations, or isometries, have a special property that distinguishes them from other transformations. This tutorial will show you what makes them special!
How Do You Determine Whether Two Chords are Equidistant From the Center of a Circle?

When you want to know if two chords are the same distance away from the center of the circle, there's a quick way to get the answer. In this tutorial, you'll learn how to find that answer and figure out which chords are equidistant from the center.
How Do You Find The Measure of an Inscribed Angle When You Know the Measure of the Intercepted Arc?

When you're given the measurement of the intercepted arc, you can find the measure of the inscribed angle with a few short steps! Follow along with this tutorial to learn how to find an inscribed angle when you know the intercepted arc!
How Do You Use Intersecting Chords to Find Arc Measures in a Circle?

This tutorial shows you how to use your knowledge of intersecting arcs in a circle to find a missing arc measurement. Take a look!
How Do You Find the Measure of an Angle Created by Intersecting Chords in a Circle?

This tutorial shows you how to use your knowledge of intersecting chords in a circle to find a missing angle measurement.
How Do You Determine Whether a Line is Tangent to a Circle?

In this tutorial, you'll see how the Converse of the Pythagorean Theorem is used to figure out if a line is tangent to a circle.
How Do You Find Missing Measures of Angles in Quadrilaterals Inscribed in Circles?

When a quadrilateral is inscribed in a circle, you can find the angle measurements of the quadrilateral in just a few quick steps! Follow along with this tutorial to learn what to do!
What is a Tangent Line to a Circle?

What does it mean when a line is tangent to a circle? In this tutorial, you'll learn what a line needs to do to be a tangent line to a circle.
How Do You Use Similar Figures to Find the Area of a Polygon?

When you're working with similar figures, knowing the scale factor can help you find all sorts of pieces including side measurements and area. This tutorial shows you how to use the scale factor to help find the area of one of the figures.
How do you Figure Out if Two Figures are Similar?

Follow along with this tutorial to see an example of determining if two given figures are similar.
How Do You Find the Perimeter of a Rectangle in the Coordinate Plane?

Finding the perimeter of a rectangle in the coordinate plane is easier than you might think! This tutorial shows you how to use the coordinate plane to find the perimeter of a rectangle.
How Do You Find the Area of a Rectangle in the Coordinate Plane?

If you need to find the area of a rectangle on the coordinate plane, all you need is the length and width of that rectangle! This tutorial shows you what to do to find the area of a rectangle when it's graphed on the coordinate plane.
How Do You Find the Area of an Irregular Figure in the Coordinate Plane?

Finding the area of a composite figure in the coordinate plane is easier than you might think! Follow along with this tutorial to see the process step-by-step.
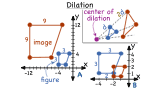
What is a Dilation?

When someone's eyes dilate, their pupils get bigger or smaller, but they always stay the same shape. Dilation in math is very similar. When you dilate a figure, you change the size of the figure without changing its shape. This tutorial introduces you to dilation. Take a look!
How Do You Find the Slope of a Line If You Have a Parallel Line?

Looking at a graph of parallel lines? Got the equation of one of the lines? Want to find the slope of the other line? No problem! Just remember that parallel lines have the same slope! Use the given equation to find the slope of the first line and since the lines are parallel, that's the slope of the second line! To see an example, check out this tutorial.
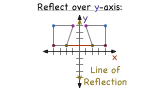
How Do You Use a Graph to Reflect a Figure Over the Y-Axis?

Reflecting a figure over the y-axis can be a little tricky, unless you have a plan. In this tutorial, see how to use the graph of a figure to perform the reflection. Check it out!
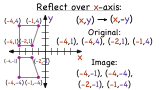
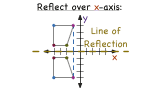
How Do You Use Coordinates to Reflect a Figure Over the X-Axis?

Want to see how to reflect a figure over the x-axis? Then this tutorial was made for you! In this tutorial, you'll see how to use coordinates from the original figure to reflect the figure over the x-axis. Take a look!
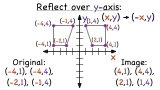
How Do You Use Coordinates to Reflect a Figure Over the Y-Axis?

Want to see how to reflect a figure over the y-axis? Then this tutorial was made for you! In this tutorial, you'll see how to use coordinates from the original figure to reflect the figure over the y-axis. Take a look!
How Do You Use a Graph to Reflect a Figure Over the X-Axis?

Reflecting a figure over the x-axis can be a little tricky, unless you have a plan. In this tutorial, see how to use the graph of a figure to perform the reflection. Check it out!
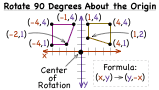
How Do You Rotate a Figure 90 Degrees Around the Origin?

Rotating a figure about the origin can be a little tricky, but this tutorial can help! This tutorial shows you how to rotate coordinates from the original figure about the origin. Then, simply connect the points to create the new figure. See this process in action by watching this tutorial!
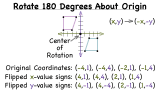
How Do You Rotate a Figure 180 Degrees Around the Origin?

Rotating a figure about the origin can be a little tricky, but this tutorial can help! This tutorial shows you how to rotate coordinates from the original figure about the origin. Then, simply connect the points to create the new figure. See this process in action by watching this tutorial!
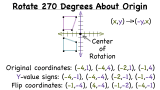
How Do You Rotate a Figure 270 Degrees Clockwise Around the Origin?

Rotating a figure about the origin can be a little tricky, but this tutorial can help! This tutorial shows you how to rotate coordinates from the original figure about the origin. Then, simply connect the points to create the new figure. See this process in action by watching this tutorial!
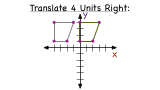
How Do You Use a Graph to Translate a Figure Horizontally?

Translating a figure on the coordinate plane is easier than you might think! In this tutorial, see how to use the graph of the original figure to perform the translation. Take a look!
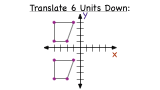
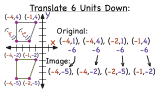
How Do You Use a Graph to Translate a Figure Vertically?

Translating a figure on the coordinate plane is easier than you might think! In this tutorial, see how to use the graph of the original figure to perform the translation. Take a look!
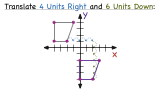
How Do You Use a Graph to Translate a Figure Diagonally?

Performing multiple translations on the graph of a figure is easier than you might think! In this tutorial, see how to use the graph of the original figure to perform each translation in order to get the graph of the new figure. Check it out!
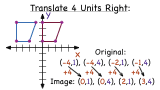
How Do You Use Coordinates to Translate a Figure Horizontally?

Translating a figure on the coordinate plane is easier than you might think! This tutorial shows you how to translate coordinates from the original figure. Then, simply connect the points to create your new figure. This tutorial shows you how!
How Do You Use Coordinates to Translate a Figure Vertically?

Translating a figure on the coordinate plane is easier than you might think! This tutorial shows you how to translate coordinates from the original figure. Then, simply connect the points to create your new figure. This tutorial shows you how!
What is a Parallelogram?

A parallelogram is a special type of quadrilateral with some special properties. In this tutorial, take a look at parallelograms and learn what kinds of quadrilaterals can also be called parallelograms!
What is a Point?

A point is a fundamental building block of math. Without points, you couldn't make lines, planes, angles, or polygons. That also means that graphing would be impossible. Needless to say, learning about points is very important! That makes this tutorial a must see!
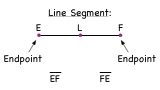
What is a Line Segment?

A math term can really tell you a lot about the thing it's describing. Take the term line segment. A line segment is just part of a line! In this tutorial, learn about line segments, how to name them, and what the midpoint of a line segment is!
What is an Angle?

Angles are a fundamental building block for creating all sorts of shapes! In this tutorial, learn about how an angle is formed, how to name an angle, and how an angle is measured. Take a look!
What are Vertical Angles?

Vertical angles have a very special quality. They are always congruent to one another! Check out this tutorial to learn about and see how to identify vertical angles!
What are Parallel Lines?

Lines that are parallel have a very special quality. Without this quality, these lines are not parallel. In this tutorial, take a look at parallel lines and see how they are different from any other kind of lines!
What are Perpendicular Lines?

Perpendicular lines have a special property. The angles formed by perpendicular lines will always be the same. Check out this tutorial to learn about perpendicular lines and see a cool trick involving these special lines!
What is a Transversal?

Ever heard of a transversal? It's not as confusing as the term sounds. This tutorial will introduce you to transversals and show you the neat things that happen when a transversal meets two parallel lines. Take a look!
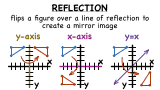
What is a Reflection?

When you look in the mirror, you see your reflection. In math, you can create mirror images of figures by reflecting them over a given line. This tutorial introduces you to reflections and shows you some examples of reflections. Take a look!
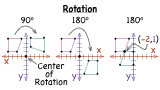
What is a Rotation?

Ever turned a door handle? You were performing a rotation! In math, rotations are just the same! Check out this tutorial to learn about rotations.
What is a Translation?

Ever slide something across a table? If so, then you have performed a translation! In this tutorial, learn the definition of translation and see some really neat examples. Take a look!
What is a Transformation?

Transformations can be really fun! They allow you to change or move a figure. In this tutorial, learn about all the different kinds of transformations!
What is an Image?

Just about everything in math has a name! Did you know that when you're dealing with transformations, the new figure you get is called an image? Check out this tutorial and learn about this math term!
What is the Triangle Sum Theorem?

When you're dealing with triangles, the Triangle Sum theorem can be very useful in finding interior angle measurements. In this tutorial, learn how to find this helpful theorem!
How Do You Find the Sine of an Angle in a Right Triangle?

A trigonometric ratio is a ratio between two sides of a right triangle. The sine ratio is just one of these ratios. In this tutorial, you'll see how to find the sine of a particular angle in a right triangle. Take a look!
How Do You Find the Cosine of an Angle in a Right Triangle?

A trigonometric ratio is a ratio between two sides of a right triangle. The cosine ratio is just one of these ratios. In this tutorial, you'll see how to find the cosine of a particular angle in a right triangle. Take a look!
How Do You Find the Tangent of an Angle in a Right Triangle?

A trigonometric ratio is a ratio between two sides of a right triangle. The tangent ratio is just one of these ratios. In this tutorial, you'll see how to find the tangent of a particular angle in a right triangle. Take a look!
How Do You Find a Missing Side of a Right Triangle Using Sine?

The sine ratio is not only used to identify a ratio between two sides of a right triangle, but it can also be used to find a missing side length. This tutorial shows you how to use the sine ratio to find that missing measurement!
How Do You Find a Missing Side of a Right Triangle Using Cosine?

The cosine ratio is not only used to identify a ratio between two sides of a right triangle, but it can also be used to find a missing side length. This tutorial shows you how to use the cosine ratio to find that missing measurement!
How Do You Find a Missing Side of a Right Triangle Using Tangent?

The tangent ratio is not only used to identify a ratio between two sides of a right triangle, but it can also be used to find a missing side length. This tutorial shows you how to use the tangent ratio to find that missing measurement!
How Do You Find the Area of a Sector of a Circle?

Trying to find the area of a sector of a circle? Then check out this tutorial! You'll see how to use given information and the formula for the area of a sector to find the answer. Take a look!
How Do You Find the Volume of a Cylinder?

The volume of a cylinder is the amount of space that will fit inside it. You can use the formula for the volume of a cylinder to find that amount! In this tutorial, see how to use that formula and the radius and height of the cylinder to find the volume. Check it out!
How Do You Find the Height of a Cylinder if You Know the Volume?

Want to find the height of a cylinder? Already know the volume of the cylinder and radius of the base? Then, you can use the formula for the volume of a cylinder to find the height! Check out this tutorial to see how!
How Do You Find the Volume of a Cone?

To find the volume of a cone, you need to plug in the measurement for the height of the cone and the radius of the base into the formula for the volume of a cone. Then simplify to get your answer. This tutorial shows you the entire process step-by-step!
How Do You Find the Volume of a Sphere?

Want to find the volume of a sphere? If you know the radius of the sphere, you can simply plug that value into the formula for the volume of a sphere and simplify! This tutorial shows you how!
How Do You Find the Volume of a Triangular Pyramid?

If you want to find the volume of a triangular pyramid, you'll need to know the length and height of the base and the height of the pyramid. Once you have those values, you can plug them into the formula for the volume of a triangular pyramid and simplify. Check out this tutorial to see this process!
How Do You Find the Volume of a Rectangular Pyramid?

To find the volume of a rectangular pyramid, you need to know the length and width of the base and the height of the pyramid. Then, take those values, plug them into the formula for the volume of a rectangular pyramid, and simplify to get your answer! Watch this tutorial to see how it's done!
What is a Circle?

Circles are a fundamental part of math! In this tutorial, you'll be introduced to circles and see the different parts of a circle such as the diameter, radius, and chord. Check out this tutorial to learn about circles!
What is a Sector and Central Angle?

Just about everything in math has a name! Did you know that a fraction of the area of a circle is known as a sector? This tutorial introduces you to the term sector and gives you examples of sectors. Take a look!
What is Circumference?

The circumference of a circle is the distance around that circle. But what is the formula to find the circumference? In this tutorial, you'll learn the formulas for the circumference of a circle. Take a look!
What is the Formula for the Area of a Circle?

Did you know that you can figure out the formula for the area of a circle by first turning the circle into a parallelogram? It seems a little weird, but it really works! Watch this tutorial to see how it's done!
What is the Formula for the Area of a Sector of a Circle?

A sector is just a fraction of the area of a circle. Did you know that there's a formula to help you find the area of a sector? In this tutorial, you'll learn how to find that formula! Take a look!
What is the Formula for the Volume of a Cylinder?

Did you know that you can use the formula for the area of a circle to find the formula for the volume of a cylinder? In this tutorial, you'll see how to do just that! Watch this tutorial to learn about the formula for the volume of a cylinder.
What is the Formula for the Volume of a Pyramid?

Looking for the formula for the volume of a pyramid? Then check out this tutorial! You'll learn about the formula for the volume of a pyramid and see how to use the formula in an example. Take a look!
What is the Formula for the Volume of a Cone?

Looking for the formula for the volume of a cone? Then check out this tutorial! You'll learn about the formula for the volume of a cone and see how to use the formula in an example. Take a look!
What is the Formula for the Volume of a Sphere?

Trying to find the formula for the volume of a sphere? Then check out this tutorial! You'll see how to use a cylinder with the same dimensions to find the formula for the volume of a sphere. Take a look!