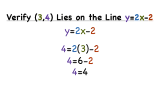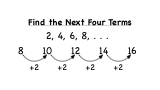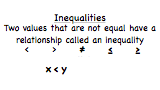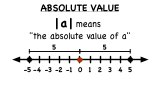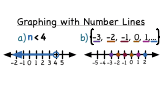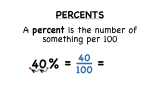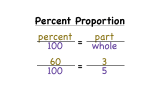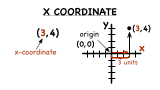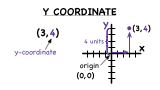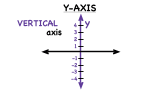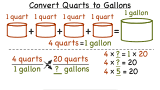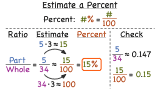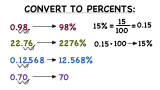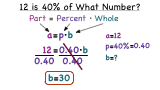More Specific Topics in
Popular Tutorials in
-
How Do You Find the Absolute Value of Positive and Negative Numbers?
Finding the absolute value of a number is a breeze when you use a number line! Remember, absolute value is the distance from zero on the number line. This tutorial shows you how to use a number line to find absolute value.
-
How Do You Convert a Rate To A Unit Rate?
When you talk about the speed of a car, you usually say something in miles per hour. For example, you say, 'I drove 40 miles per hour.' Normally, you don't say, 'I drove 120 miles per 3 hours.' Figure out how to convert a rate like 120 miles per 3 hours to the unit rate of 40 miles per hour by watching this tutorial.
-
What are Similar Figures?
Looking at two figures that are the same shape and have the same angle measurements? You have similar figures! Learn all about it in this tutorial!
-
What are Rates and Unit Rates?
Can you do 100 sit-ups in 2 minutes? That's a rate! Driving a car going 40 miles per hour? That's a unit rate! Watch this tutorial to learn about rate and unit rate (and the difference!).
-
What is a Scale Drawing?
Without a blueprint, it would be really hard to construct a building. Without a road map, you'd be lost! Scale drawings make it easy to see large things, like buildings and roads, on paper. Even a GPS uses scale drawings! Check out this tutorial to learn all about scale drawings.
-
What are Corresponding Parts in Similar Figures?
Identifying corresponding parts in similar figures isn't so bad, but you have to know what you're looking for. This tutorial does a great job of explaining the corresponding parts of similar figures! Take a look!
-
How Do You Write the Prime Factorization of a Number Using Exponents?
When you're writing the prime factorization of a number, you may be able to use exponents to quickly show all the factors. This tutorial shows you how to find the prime factorization of a number and use exponents to write the answer!
-
What's a Function?
Function rules are like instructions on how to change input values into their respective output values. In this tutorial, see how to write a function rule for a given relation. Check it out!
-
How Do You Check if a Point is on a Line If You Have an Equation?
Wondering if a point is part of the equation of a line? Got the equation of the line but no graph? No problem! Just take that point and plug it into the equation and simplify. If you end up with a true statement, the point is indeed part of the equation. If you end up with a false statement, then that point is not part of the equation. See this process first-hand in this tutorial!
-
How Do You Find the Next Terms in an Arithmetic Sequence?
To find the next few terms in an arithmetic sequence, you first need to find the common difference, the constant amount of change between numbers in an arithmetic sequence. Once you know the common difference, you can use it to find those next terms! This tutorial takes you through that process, so be sure to check it out!
-
What are the Endpoints of a Line Segment?
A math term can really tell you a lot about the thing it's describing. Take the term 'endpoints'. The endpoints of a line segment are just the 'points' located at the 'ends' of the line segment! That's an informative name! Watch this tutorial to learn about endpoints of a line segment.
-
What's a Sequence?
Got a set of numbers? Are they in a particular order? If so, then you have a sequence! Take a look at sequences by watching this tutorial.
-
What Does the Slope of a Line Mean?
You can't learn about linear equations without learning about slope. The slope of a line is the steepness of the line. There are many ways to think about slope. Slope is the rise over the run, the change in 'y' over the change in 'x', or the gradient of a line. Check out this tutorial to learn about slope!
-
What are Multiplicative Inverses?
Multiplicative inverses. That's a mouthful! Really, this term just refers to numbers that when multiplied together equal 1. These numbers are also called reciprocals of each other! Learn about multiplicative inverses by watching this tutorial.
-
How Do You Use a Tree Diagram to Count the Number of Outcomes in a Sample Space?
When you're trying to figure out all the possibilities from different options, it can be helpful to make a tree diagram. In this tutorial, you'll see how to use a tree diagram to figure out how many different outfits can be created from the possible shirts, bottoms, and shoes given. Check it out!
-
How Do You Use the Fundamental Counting Principle to Count the Number of Outcomes in a Sample Space?
When you're trying to figure out all the possibilities from different options, it can be helpful to draw a picture. In this tutorial, you'll see how to use a picture to figure out how many different outcomes can be created from the possibilities given. Check it out!
-
How Do You Find the Probability of a Simple Event?
Working with probabilities? Check out this tutorial! You'll see how to calculate the probability of picking a certain marble out of a bag.
-
How Do You Find the Probability of Independent Events?
Calculating probabilities? Take a look at this tutorial and see how to figure out the probability of independently drawing certain cards from a deck!
-
How Do You Find the Mean of a Data Set?
Mean is just another name for average. To find the mean of a data set, add all the values together and divide by the number of values in the set. The result is your mean! To see an example of finding the mean, watch this tutorial!
-
How Do You Find the Median of a Data Set?
The median of a data set is the number that is the middle value of the set. It's easy to find the median if you first put the numbers in order from least to greatest. In this tutorial, see how to find the median of a data set, and see what to do if there are two middle values!
-
How Do You Find the Mode of a Data Set?
The mode of a data set is the number that occurs most frequently in the set. To easily find the mode, put the numbers in order from least to greatest and count how many times each number occurs. The number that occurs the most is the mode! Follow along with this tutorial and see how to find the mode of a set of data.
-
How Do You Find the Range of a Data Set?
The range of a data set is the difference between the largest number and the smallest number. In this tutorial, you'll see how to find the range of a set of data. Check it out!
-
How Do You Make a Histogram?
If you want to see data from a frequency table in a more visual way, try creating a histogram to show off that data! This tutorial shows you what to do!
-
How Do You Make a Box-and-Whisker Plot?
A box-and-whisker plot can help you get a better picture of what your data looks like visually. This tutorial shows you the step-by-step process for making a box-and-whisker plot!
-
How Do You Make a Frequency Table?
Frequency tables help you more easily see what occurs most often in a set of data. This tutorial shows you how to create a fequency table for a given set of data.
-
How Do You Find a Number of Permutations Using the Fundamental Counting Principle?
The Fundamental Counting Principle (FCP) can be used to find a number of permutations. Follow along with this tutorial to see how to use the FCP to find the number ways you can rearrange the letters in the word NUMBER.
-
How Do You Find the Probability of the Complement of an Event?
Probability can help you solve all sorts of everyday problems! This tutorial shows you how to find the probability of the complement of an event using gummy worms!
-
How Do You Compare the Mean and Median of Data Sets Using a Line Plot
The mean and median can help you better understand a data set. Check out this real world example involving finding and comparing the mean and median of multiple data sets.
-
What is an Outcome?
When you're conducting an experiment, the outcome is a very important part. The outcome of an experiment is any possible result of the experiment. Learn about outcomes by watching this tutorial!
-
What is a Sample Space?
In an experiment, it's good to know your sample space. The sample space is the set of all possible outcomes of an experiment. Watch this tutorial to get a look at the sample space of an experiment!
-
What is the Fundamental Counting Principle?
The Fundamental Counting Principle is a way to figure out the total number of ways different events can occur. In this tutorial, you'll be introduced to this principle and see how to use it in an example. Take a look!
-
What is Probability?
Probability can help you solve all sorts of everyday problems, but first you need to know what probability is! Follow along with this tutorial to learn about probability!
-
What is the Median of a Data Set?
The median is one of many measures of central tendency. Check out this tutorial to learn what the median is and how you can find it!
-
What is the Mode of a Data Set?
Finding the mode of a set of data can help you understand the data better. This tutorial introduces you to mode and shows you how to find this helpful measure of central tendency!
-
What is the Mean of a Data Set?
When you think of the mean of a data set, think of the word average. 'Mean' and 'average' are the same thing when you're talking about a set of data! This tutorial introduces you to mean and shows you how to find it!
-
What is a Stem-And-Leaf Plot?
Stem-and-leaf plots can be really helpful in visually interpreting data. This tutorial introduces you to stem-and-leaf plots, shows you how to use this special type of graph, and explains some of the popular uses. Take a look!
-
What is a Histogram?
Looking for info on histograms? Check this out:
-
What is a Frequency Table?
Frequency tables tell you how often something occurs in a set of data. This tutorial introduces you to frequency tables and shows you some of the ways they can be used to interpret data!
-
What is the Interquartile Range?
Being able to disect a set of data and better understand it is a key part of mastering statistics. This tutorial teaches you about one of these disection tools called the interquartile range.
-
What is Experimental Probability?
Do real life situations always work out the way your mathematical models tell you they should? No! This tutorial describes how experimental probability differs from theoretical probability.
-
What is the Complement of an Event?
When you learn about probablilities, the complement of an event is a must-know term! This tutorial introduces you the complement of an event.
-
What are the Commutative Properties of Addition and Multiplication?
The commutative property is a fundamental building block of math, but it only works for addition and multiplication. This tutorial defines the commutative property and provides examples of how to use it.
-
What are the Identity Properties of Addition and Multiplication?
Let's identify an identity! Addition and subtraction have a different identity than multiplication and division. Learn about each of these identities with this tutorial!
-
How Do You Translate a Phrase with One Variable and Number Into a Mathematical Expression?
Need some practice translating phrases into mathematical expressions? Then this tutorial is for you! You'll get practice translating statements involving addition, subtraction, multiplication, or division into mathematical expressions.
-
What are Natural and Whole Numbers?
The natural numbers are also called the counting numbers. The whole numbers are almost exactly as the natural numbers the same except for one small difference! Watch this tutorial and learn the difference between natural and whole numbers.
-
What is a Variable?
You can't do algebra without working with variables, but variables can be confusing. If you've ever wondered what variables are, then this tutorial is for you!
-
What Are Some Words You Can Use To Write Word Problems?
Knowing the mathematical meaning of words allows you to decipher word problems and gives you the power to write your own word problems, too! Take a look at these words and learn their mathematical translations.
-
What is the Distributive Property?
The distributive property is a very deep math principle that helps make math work. It's the rule that lets you expand parentheses, and so it's really critical to understand if you want to get good at simplifying expressions. So check out the tutorial and let us know what you think!
-
What are the Associative Properties of Addition and Multiplication?
The associative property is one of those fundamental properties of math that make math work. You probably take this property for granted because it's so ingrained, but it's important to see how the guts of math work, so check out the tutorial and make sure you're solid on your fundamentals!
-
What's the Order of Operations?
Check out this tutorial where you'll see exactly what order you need to follow when you simplify expressions. You'll also see what happens when you don't follow these rules, and you'll find out why order of operations is so important!
-
What's a Coefficient?
There is a bunch of vocabulary that you just need to know when it comes to algebra, and coefficient is one of the key words that you have to feel 100% comfortable with. Check out the tutorial and let us know if you want to learn more about coefficients!
-
How Do You Do Long Division?
We know that calculators are everywhere, but that doesn't mean that long division isn't important! Sometimes you won't be allowed to use a calculator, and when those times occur, you'll be thankful that you watched this video!
-
What's a Dividend and What's a Divisor?
When you do division problems, you need to know the vocabulary that people use to describe what number is being divided, and what number is doing the dividing. This tutorial will help you keep that vocabulary straight!
-
How Do You Do Long Division with Decimals?
We know that calculators are everywhere, but that doesn't mean that long division isn't important! Sometimes you won't be allowed to use a calculator, and when those times occur, you'll be thankful that you watched this video!
-
What's an Inequality?
Inequalities come up all the time when you're working algebra problems. In this tutorial you'll learn what an inequality is, and you'll see all the common inequality symbols that you're likely to see :)
-
How Do You Turn a Mixed Fraction Into an Improper Fraction?
Working with mixed fractions in equations can be tough, but things get easier if you convert them into improper fractions first. Once you learn this skill, you'll find yourself using it all the time, so take look at how to convert a mixed fraction to an improper fraction.
-
How Do You Multiply and Divide Numbers with Different Signs?
A positive times a positive is a positive. A negative times a negative is a positive. What about a negative times a positive? This tutorial gives you the answer by showing you how multiply (and divide!) with mixed signs.
-
How Do You Find a Common Denominator and a Least Common Denominator?
This tutorial gives you some practice finding a common denominator and the least common denominator of three fractions. There's only one least common denominator, but there are many common denominators. This tutorial gives you one. Can you find another?
-
How Do You Multiply Fractions?
Working with fractions can be intimidating, but if you arm yourself with the right tools, you'll find that working with fractions is no harder than working with basic numbers. In this tutorial you'll see the process for multiplying 3 very simple fractions. Enjoy!
-
How Do You Add Fractions with Different Denominators?
Trying to add fractions with unlike denominators? You're going to need a common denominator first! Follow along with this tutorial and see what you need to do to add these fractions together.
-
How Do You Add a Negative Number to a Positive Number?
How do you combine a positive and a negative number? This tutorial shows you how. You even get to see it explained with a number line!
-
What's a Mixed Number?
Fractions come in all sorts of flavors, and in this tutorial you'll learn how to recognize mixed numbers.
-
What's an Improper Fraction?
Fractions come in all sorts of flavors, and in this tutorial you'll learn how to recognize improper fractions.
-
How Do You Multiply a Whole Number by a Fraction?
Multiplying a whole number and a fraction can be confusing, but this tutorial helps to sort things out. Check it out!
-
What Does Absolute Value Mean?
In this tutorial you'll see how you can think of absolute value in a very intuitive way. Let us know if you have any questions about it!
-
How Do You Figure Out the Sign of a Product or Quotient?
Multiplying and dividing numbers takes a good amount of thinking, and it's easy to make a mistake. But you can make sure that you're on the right track if you check whether the answer should be positive or negative. In this tutorial you'll see exactly how to tell if your answer will be positive or negative, even if you don't know the exact value of the answer. That way you'll always be able to check your answers!
-
What's a Prime Number?
Prime numbers aren't too hard to define, but they still puzzle professional mathematicians. Believe it or not, all over the word computers are chugging away, trying to find the next biggest prime! Bigger and bigger prime numbers help keep your credit card info safe through really cool encryption techniques. So prime numbers really matter every day, and you can learn how they are defined in this tutorial.
-
How Do You Add Three Fractions with the Same Denominator?
While adding fractions can be hard, adding fractions with the same denominator is just as easy as adding numbers. That's why when you add fractions you first get all of them to have the same denominator, and then add them up. In this tutorial you get to see just how easy it is to add up fractions once they have the same denominator!
-
How Do You Subtract a Positive Number from a Negative Number?
Subtracting a positive from a negative? Just remember: subtracting a positive is the same as adding a negative. See how it works in this tutorial!
-
How Do You Subtract a Negative Number from a Positive Number?
Subtracting a negative from a positive? Just remember: subtracting a negative is the same as adding a positive. See how it works in this tutorial!
-
How Do You Graph an Inequality or an Infinite Set on a Number Line?
Number lines are really useful in visualizing an inequality or a set. In this tutorial, you'll see how to graph both. Take a look!
-
How Do You Write an Inequality from a Number Line Graph?
Writing inequalities from a graph on a number line isn't so bad if you know what to do. Watch this tutorial to learn how!
-
What's an Integer?
There are lots of different kinds of numbers that you'll come across in algebra, and a lot of these kinds of numbers are related to each other. Before you learn how they are related, you've got to learn about them separately, and in this tutorial you'll how to define integers :)
-
What's a Reciprocal?
Reciprocals are important when it comes to dividing fractions, finding perpendicular lines, dealing with inverse proportions, and so much more! In this tutorial you can review the basics about reciprocals.
-
How Do You Rewrite Subtraction as Addition?
Subtracting a positive is the same thing as adding a negative. Subtracting a negative is the same as adding a positive. Get a closer look with this tutorial!
-
How Do You Add Two Negative Numbers?
Adding two negative numbers together? Just add the absolute value of each number together, put a negative sign in front, and you have your answer! See how it's done in this tutorial.
-
How Do You Turn an Improper Fraction Into a Mixed Fraction?
In math, it's often important to change a fraction from one type to another. It can help you work with the fraction in an equation or help make more sense of an answer. This tutorial shows you how to convert an improper fraction to a mixed fraction.
-
How Do You Find the Greatest Common Factor of Two Numbers Using Prime Factorization?
This tutorial uses something called a factor tree to find the greatest common factor of two numbers. Creating a factor tree for a number makes it easier to find its prime factors. These prime factors are used to help find the greatest common factor. Watch this tutorial and learn how to find the greatest common factor using a factor tree.
-
How Do You Subtract Decimals?
Doing math with paper and pencil can come in real handy, so make sure you're comfortable subtracting decimals by hand. After all, you don't want the calculator to be a crutch!
-
How Do You Add Decimals?
Doing math with paper and pencil can come in real handy, so make sure you're comfortable adding decimals by hand. After all, you don't want the calculator to be a crutch!
-
How Do You Multiply Decimals?
Doing math with paper and pencil can come in real handy, so make sure you're comfortable multiplying decimals by hand. After all, you don't want the calculator to be a crutch!
-
What are Trailing Zeros?
Ever wondered about the zeros that come at the end of a decimal number? What are those things anyway? Do they actually matter in terms of the value of the number? Watch the tutorial and see for yourself :)
-
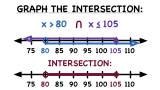
How Do You Graph the Intersection of Two Inequalities?
Number lines help make graphing the intersection of two inequalities a breeze! This tutorial shows you how to graph two inequalities on the same number line and then find the intersection. Take a look!
-
How Do You Graph the Union of Two Inequalities?
Number lines help make graphing the union of two inequalities a breeze! This tutorial shows you how to graph two inequalities on the same number line and then find the union. Check it out!
-
What's the Subtraction Property of Equality?
Solving equations can be tough, especially if you've forgotten or have trouble understanding the tools at your disposal. One of those tools is the subtraction property of equality, and it lets you subtract the same number from both sides of an equation. Watch the video to see it in action!
-
What's the Addition Property of Equality?
Solving equations can be tough, especially if you've forgotten or have trouble understanding the tools at your disposal. One of those tools is the addition property of equality, and it lets you add the same number to both sides of an equation. Watch the video to see it in action!
-
What's the Multiplication Property of Equality?
Solving equations can be tough, especially if you've forgotten or have trouble understanding the tools at your disposal. One of those tools is the multiplication property of equality, and it lets you multiply both sides of an equation by the same number. Watch the video to see it in action!
-
How Do You Solve a Word Problem with an Equation Using Addition?
Word problems are a great way to see math in action! See how to translate a word problem into an equation, solve to find the answer, and check your found answer all in this tutorial.
-
How Do You Solve an Equation Using Addition?
Solving an equation for a variable? Perform the order of operations in reverse! Check it out in this tutorial.
-
How Do You Solve a Word Problem with an Equation Using Subtraction?
Word problems are a great way to see math in action! See how to translate a word problem into an equation, solve to find the answer, and check your found answer all in this tutorial.
-
How Do You Solve a Word Problem Using an Equation Where You're Multiplying Fractions?
Working with word problems AND fractions? This tutorial shows you how to take a word problem and translate it into a mathematical equation involving fractions. Then, you'll see how to solve and get the answer. Check it out!
-
How Do You Solve an Equation Where You're Multiplying Fractions?
Solving an equation with multiple fractions in different forms isn't so bad. This tutorial shows you how to convert a mixed fraction to an improper fraction in order to solve the equation. Then, you'll see how to convert the answer back to a mixed fraction to make sense of it. Follow along with this tutorial to see how it's done!
-
How Do You Solve a Word Problem with an Equation Using Multiplication?
Working with word problems AND fractions? This tutorial shows you how to take a word problem and translate it into a mathematical equation involving fractions. Then, you'll see how to solve and get the answer. Check it out!
-
How Do You Solve an Equation Using Multiplication?
Solving an equation for a variable? Perform the order of operations in reverse! Check it out in this tutorial.
-
How Do You Solve a Word Problem with an Equation Using Division?
Word problems are a great way to see math in action! See how to translate a word problem into an equation, solve to find the answer, and check your found answer all in this tutorial.
-
How Do You Solve a Word Problem Where You Multiply Fractions and Work Backwards?
Word problems are a great way to see math in the real world. In this tutorial, you'll see how to solve a word problem by working backwards using a table. Check it out!
-
How Do You Solve an Equation by Guessing and Checking?
Want to solve an equation by guessing and checking possible answers? Then this tutorial is for you! Make sure to pay close attention to the strategy involved in guessing and checking!
-
How Do You Solve an Equation Using Subtraction?
Solving an equation for a variable? Perform the order of operations in reverse! Check it out in this tutorial.
-
What's the Division Property of Equality?
Solving equations can be tough, especially if you've forgotten or have trouble understanding the tools at your disposal. One of those tools is the division property of equality, and it lets you divide both sides of an equation by the same number. Watch the video to see it in action!
-
How Do You Solve a Word Problem Using a Percent Proportion?
Word problems allow you to see the real world uses of math! This tutorial shows you how to take a words problem and turn it into a percent proportion. Then see how to solve for the answer using the mean extremes property of proportions. Take a look!
-
How Do You Use a Proportion to Find a Percent of a Number?
Taking a percent of a number? Trying to figure out the result? Use a percent proportion to solve! This tutorial will show you how!
-
What's a Percent?
Sales tax, tips at restaurants, grades on tests... no matter what you do, you can't run away from percents. So watch this tutorial and see once and for all what percents are all about!
-
How Do You Turn a Percent into a Decimal?
Percents and Decimals are just tools that let us represent numbers, and that is why percents can be converted into decimals, and decimals can be converted into percents. In this tutorial you'll see how quickly you can convert percents into decimals!
-
How Do You Set Up a Percent Proportion from a Word Problem?
Sometimes the hardest part of a word problem is figuring out how to turn the words into an equation you can solve. This tutorial let's you see the steps to take in order to do just that! Take a look! You'll be glad you did!
-
How Do You Use an Equation to Find a Percent of a Number?
Taking a percent of a number? Trying to figure out the result? Convert the percent to a decimal and multiply it by the number! This tutorial will show you how!
-
How Do You Solve a Proportion Using Cross Products?
Want to solve a percent proportion? Just use the means extremes property of proportions to cross multiply! Solve for the variable, and you have your answer! Learn how with this tutorial.
-
How Do You Use a Proportion to Find What Percent a Part is of a Whole?
A part is some percent of a whole. Trying to calculate the percent? Use a percent proportion to solve! This tutorial will show you how!
-
What's a Percent Proportion?
Percents are important, and the reality is that percents are actually proportions in disguise. In this tutorial you'll see exactly how to connect percents with proportions, and you'll be happier for it :)
-
What's a Proportion?
The idea of proportions is that a ratio can be written in many ways and still be equal to the same value. That's why proportions are actually equations with equal ratios. This is a bit of a tricky definition, so make sure to watch the tutorial!
-
What's the Means-Extremes Property of Proportions?
The means-extremes property of proportions allows you to cross multiply, taking the product of the means and setting them equal to the product of the extremes. This property comes in handy when you're trying to solve a proportion. Watch this tutorial to learn more!
-
What's a Ratio?
Ratios are everywhere! The scale on a map or blueprint is a ratio. Ingredients sometimes need to be mixed using ratios such as the ratio of water to cement mix when making cement. Watch this tutorial to learn about ratios. Then think of some ratios you've encountered before!
-
How Do You Solve a Word Problem Using a Proportion?
This tutorial provides a great real world application of math. You'll see how to use the scale from a blueprint of a house to help find the actual height of the house. This tutorial shows you how to use a proportion to solve!
-
What's a Compound Inequality?
Wondering what a compound inequality is? Then check out this tutorial! You'll learn the definition for a compound inequality and also see how it can be written in set builder notation. Take a look!
-
What Are Some Words We Use To Write Inequalities?
Knowing the definition for a compound inequality is one thing, but being able to identify one in a word problem or phrase can be an entirely different challenge. Arm yourself by learning some of the common phrases used to describe a compound inequality and an absolute value inequality.
-
What's Scientific Notation?
Sometimes a number is so big (or so small), that it takes a while to write it all down. Luckily, this number can be written quicker using scientific notation! Watch this tutorial and learn about scientific notation.
-
How Do You Find the Greatest Common Factor of Three Numbers?
To find the greatest common factor (GCF) between numbers, take each number and write its prime factorization. Then, identify the factors common to each number and multiply those common factors together. Bam! The GCF! To see an example worked out, check out this tutorial!
-
What is a Constant?
Constants are parts of algebraic expressions that don't change. Check out this tutorial to see exactly what a constant looks like and why it doesn't change.
-
How Do You Find the Prime Factorization of a Number Using a Tree?
To write the prime factorization for a number, it's often useful to use something called a factor tree. Follow along with this tutorial and see how to use a factor tree to find the prime factorization of a given number.
-
What's Another Definition for Prime and Composite Numbers?
If the only factors a number are 1 and itself, then that number is prime. A number that is not prime is called composite. Learn about prime and composite numbers by watching this tutorial!
-
What's the Formula for the Perimeter of a Rectangle?
What is the formula for the perimeter of a rectangle? This tutorial shows you how to find that formula!
-
How Do You Find the Perimeter of a Shape?
Ever had someone come up to you on the street, hand you a shape, and ask you to find the perimeter of that shape? Watch this tutorial, and next time that happens you'll be ready!
-
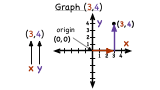
How Do You Plot Points in the Coordinate Plane?
Knowing how to plot ordered pairs is an essential part of graphing functions. In this tutorial, you'll see how to take an ordered pair and plot it on the coordinate plane. Take a look!
-
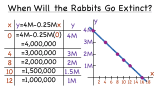
How Do You Use the Graph of a Linear Equation to Solve a Word Problem?
Word problems are a great way to see math in the real world! In this tutorial, see how to figure out how long it will take for a rabbit population to go extinct. You'll also see how to set up a table and a graph to help find the answer!
-
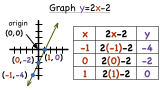
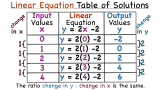
How Do You Graph a Linear Equation by Making a Table?
Graphing a function? It would be really helpful if you had a table of values that fit your equation. You could plot those values on a coordinate plane and connect the point to make your graph. See it all in this tutorial!
-
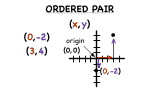
What is an Ordered Pair?
Ordered pairs are a fundamental part of graphing. Ordered pairs make up functions on a graph, and very often, you need to plot ordered pairs in order to see what the graph of a function looks like. This tutorial will introduce you to ordered pairs!
-
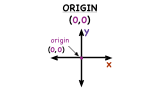
What is the Origin?
The coordinate plane has two axes: the horizontal and vertical axes. These two axes intersect one another at a point called the origin. Learn about the ordered pair that indicates the origin and its location in the coordinate plane by watching this tutorial!
-
What's a Function?
You can't go through algebra without learning about functions. This tutorial shows you a great approach to thinking about functions! Learn the definition of a function and see the different ways functions can be represented. Take a look!
-
What is the Coordinate Plane?
You can't graph a function or plot ordered pairs without a coordinate plane! Learn about the coordinate plane by watching this tutorial.
-
What are Quadrants in the Coordinate Plane?
Did you know that there are four quadrants that help make up the coordinate plane? Learn about these quadrants, and what ordered pairs are located in each, by watching this tutorial!
-
What is the X-Coordinate?
Ordered pairs are a crucial part of graphing, but you need to know how to identify the coordinates in an ordered pair if you're going to plot it on a coordinate plane. In this tutorial, you'll see how to identify the x-coordinate in an ordered pair!
-
What is the Y-Coordinate?
Ordered pairs are a crucial part of graphing, but you need to know how to identify the coordinates in an ordered pair if you're going to plot it on a coordinate plane. In this tutorial, you'll see how to identify the y-coordinate in an ordered pair!
-
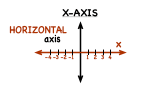
What is the X-Axis?
To graph a function or plot an ordered pair, you need to use a coordinate plane, so you should learn all about it! In this tutorial, you'll learn about the x-axis and see where it's located in the coordinate plane.
-
What is the Y-Axis?
To graph a function or plot an ordered pair, you need to use a coordinate plane, so you should learn all about it! In this tutorial, you'll learn about the y-axis and see where it's located in the coordinate plane.
-
How Do You Add Whole Numbers?
To add numbers, you can line up the numbers vertically and then add the matching places together. This tutorial shows you how to add numbers vertically!
-
How Do You Subtract Whole Numbers?
Subtracting numbers is easier than you might think! Watch this tutorial to see the step-by-step process of lining up the numbers vertically to subtract them.
-
How Do You Divide Whole Numbers?
Division is a building block of math. This tutorial shows you how to perform long division by going through the process one step at a time.
-
How Do You Solve a Word Problem by Dividing Whole Numbers?
Word problems are a great way to see math in the real world! Math can help us answer everyday questions. Watch this tutorial to see how division is used to solve a word problem.
-
How Do You Write a Whole Number Given in Expanded Form in Standard Form and in Words?
Being able to change the form of a number is an important skill! This tutorial helps you practice taking a number in expanded form and rewriting it in standard form and in words.
-
How Do You Write a Whole Number Given in Standard Form in Expanded Form and in Words?
If you have a number in standard form, you can quickly change it into expanded form or even into words with a few steps! Watch this tutorial to learn the steps needed to change from one form to another.
-
How Do You Write a Whole Number Given in Words in Standard Form and in Expanded Form?
You can take a number from words and put it into standard form or even expanded form with a few quick steps! See how to change a number from one from to another by watching this tutorial.
-
How Do You Compare Two Whole Numbers?
Comparing two large whole numbers can be a chore, but using a place value chart can make the process easier. Take a look at how to line up the digits and figure out which number is larger.
-
How Do You Put Whole Numbers in Order From Least to Greatest?
Ordering large numbers can be tricky, but a place value chart can make the process easier. This tutorial shows you how to use a place value chart to put large numbers in order!
-
How Do You Find the Value of a Digit in a Whole Number?
Understanding place value is a building block of understanding numbers. Follow along with this tutorial to see how to find the value of a digit for a given number!
-
How Do You Put Whole Numbers in Order From Greatest to Least?
If you're ordering numbers from least to greatest, you can use a place value chart to help! See how to use a place value chart to order numbers by watching this tutorial.
-
How Do You Estimate a Multiplication Problem by Rounding?
Many real world math problems can be solved mentally. In this tutorial, you'll see how to estimate the answer to a word problem by multiplying mentally!
-
How Do You Use Powers to Solve a Word Problem?
Sometimes word problems can be made a little easier if you use exponents! This tutorial shows you how to solve a word problem by writing an expression using exponents.
-
How Do You Simplify an Expression Using the Order of Operations?
You can't simplify an expression without fully understanding the order of operation! Follow along with this tutorial to see how to use the order of operations to simplify an expression.
-
How Do You Simplify an Expression Using the Order of Operations with Exponents?
If you have an expression with more than one operation, you can use the order of operations to help you simplify! Follow along with this tutorial to see how to use the order of operations to simplify an expression.
-
How Do You Evaluate an Algebraic Expression with One Variable?
If you have a value for a variable in an expression, you can evaluate that expression by plugging in that variable value and simplifying! Follow along with this tutorial to see the entire step-by-step process.
-
How Do You Evaluate an Algebraic Expression with Two Variables?
If you have an expression with two variables and you're given the values for those variables, you can plug those values into the expression and simplify to get the answer! This tutorial shows you the steps to plugging variable values into an expression and simplifying.
-
How Do You Complete a Table By Plugging in Values?
Learning how to complete a table of values is a building block of math. You can use a table to see a pattern or even make a graph! This tutorial shows you how to complete a table when you're given an expression and multiple values to plug in.
-
How Do You Write a Rule for a Table?
Patterns are everywhere in math! In this tutorial, you'll see how to find a pattern in a table and make a rule for it!
-
How Do You Use the Distributive Property to Rewrite and Solve an Addition Problem?
Did you know you can solve an addition problem using the distributive property? This tutorial shows you how to do exactly that!
-
How Do You Distribute a Number into an Addition Problem to Solve?
If you're trying to multiply the sum of two numbers, the distributive property may make things easier. Follow along with this tutorial to see how the distributive property can be used to evaluate an expression.
-
How Do You Extend a Sequence by Multiplying?
A sequence of numbers follows a pattern. If you can find that pattern, you can find more numbers in the sequence! In this tutorial, you'll see how to find the next terms in a sequence by first identifying the pattern of that sequence.
-
How Do You Find a Missing Term in a Sequence?
Finding the missing terms of a sequence is a breeze, as long as you know the pattern for the sequence. Follow along with this tutorial to see how to find the pattern of a sequence and then use it to find missing terms of that sequence!
-
How Do You Solve a Problem by Making a Table and Finding a Pattern?
Making a table can be a very helpful way to find a pattern in numbers and solve a problem. This tutorial shows you how to take the information from and word problem to create a table and use it to find the answer!
-
How Do You Solve a Problem Using the Guess, Check, and Revise Method?
Some problems can be solve by guessing an answer, checking it, and then revising your guess. This tutorial goes through that process step-by-step and shows you how to solve a word problem using the guess, test, revise method!
-
How Do You Solve a Problem by Drawing a Diagram?
When your solving a word problem involving distance, drawing a diagram is a great way to see the problem! This tutorial shows you how to make and use a diagram for a word problem involving distance.
-
How Do You Solve a Problem by Making an Organized List?
Organization is a big part of math. In this tutorial, you'll see how organizing information given in a word problem can help you solve the problem and find the answer!
-
How Do You Solve a Problem Using Logical Reasoning?
Using logic is a strong approach to solving math problems! This tutorial goes through an example of using logical reasoning to find the answer to a word problem.
-
How Do You Multiply a Three-Digit Number by a One-Digit Number?
If you want to multiply a three digit number by a one digit number, it can be helpful to line up the multiplication vertically. This tutorial takes you through the process of multiplying!
-
How Do You Multiply a Two-Digit Number by a One-Digit Number?
Multiplication is a building block of math, so it's important to be comfortable with it! This tutorial gives you practice multiplying a one digit number by a two digit number.
-
How Do You Multiply a Two-Digit Number by a Two-Digit Number?
When you're multiplying a two digit number by a two digit number, it's important to keep the places lined up correctly. This tutorial helps you practice multiplying while keeping everything organized!
-
How Do You Divide a Two-Digit Number by a One-Digit Number With No Remainder?
Performing a division? Draw a picture to help you understand it! This tutorial shows you how to draw a picture for a division problem and then takes you through the steps of performing the division.
-
How Do You Divide a Two-Digit Number by a One-Digit Number With a Remainder?
Follow along to see how to perform long division! This tutorial shows you the steps you need to become a pro at long division!
-
How Do You Find a Value to Make an Open Sentence True?
Solving open sentences can be fun! Follow along with this tutorial to see how to find the values that make an open sentence true!
-
How Do You Multiply a Two-Digit Number by a Two-Digit Number Using Partial Products?
You can use partial products to help you multiply two numbers together! Follow the steps in this tutorial to see how partial products can make a multiplication problem easier!
-
How Do You Write a Rule for a Pattern?
Some lists of numbers follow a pattern. To find that pattern, you need to know what you're looking for. This tutorial shows you how to find a pattern for a list of numbers and then write a rule that follows the pattern!
-
How Do You Divide a Three-Digit Number by a One-Digit Number?
Long division can help you keep everything in order when you divide. Follow along to see an example of using long division to solve!
-
How Do You Figure Out What a Remainder Means When You're Dividing?
Division can help you solve all kinds of everyday problems! Just check out this tutorial involving sharing animal crackers!
-
What is the Commutative Property of Addition?
When you're adding numbers together, does their order matter? This tutorial can answer that question! It looks at the Commutative Property of Addition!
-
What is the Commutative Property of Multiplication?
When you're multiplying numbers, the Commutative Property of Multiplication can be very useful! This tutorial introduces this handy property and shows you how to use it.
-
What is the Associative Property of Addition?
The Associative Property of Addition is a useful tool when you're adding multiple numbers together! Follow along with this tutorial to learn about this property and see how to use it.
-
What is the Associative Property of Multiplication?
The Associative Property of Multiplication is a building block of math! Follow along with this tutorial to learn all about this helpful property!
-
What is Place Value for Whole Numbers?
Place value is a building block of numbers. Without place value, we couldn't keep all our numbers straight! This tutorial introduces place value and shows you the parts of a place value chart.
-
What is an Exponent?
If you have a repeated multiplication, you could write it using exponents! This tutorial introduces exponents and explains how they're used.
-
What is an Open Sentence?
Understanding open sentences is a stepping stone for understanding more complex math problems. This tutorials introduces open sentences and gives you an example of how to solve one!
-
How Do You Make a Problem Solving Plan?
Planning is a key part of solving math problems. Follow along with this tutorial to see the steps involved to make a problem solving plan!
-
Which Operations Do You Do First in an Expression?
You can't simplify a problem without the order of operations. In this tutorial, you'll learn about this special order and see how to use it to solve a problem!
-
How Can You Distribute Numbers When You're Adding and Multiplying?
You can use the distributive property to help you add and multiply numbers! Follow along with this tutorial to see the uses of the distributive property.
-
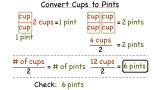
How Do You Convert Cups to Pints?
It can be helpful to see a capacity given in different units. This tutorial shows you how to take a measurement in cups and convert it to pints!
-
How Do You Convert Quarts to Gallons?
If you go to the store to buy a certain number of quarts of milk and the store only sells milk in gallons, what do you do? You need to convert those quarts into gallons! Follow along with this tutorial to learn how.
-
How Do You Convert From Milliliters to Liters?
Milliliters and liters are two units that measure capacity. In this tutorial, you'll see how to take a given number of milliliters and convert it to liters!
-
How Do You Determine the Best Customary Units to Measure a Weight?
Want to weigh an object, but not sure the best customary unity to use? This tutorial can help! It shows you how to determine the best customary unit to use for measuring the weight of a given object.
-
How Do You Determine the Best Customary Units to Measure a Capacity?
Many customary units can be used to measure the capacity of an object, but usually one unit would be better to use than the others. In this tutorial, you'll see how to choose the best customary unit to measure the capacity for a given item.
-
How Do You Determine the Best Metric Units to Measure a Mass?
Want to measure the mass of an object? There are multiple metric units to choose from! This tutorial goes through the process of choosing the best metric unit to use for measuring the mass of a given object.
-
How Do You Determine the Best Metric Units to Measure a Capacity?
There's more than one unit to measure the capacity of an object. Sometimes, one unit is a better choice to use for a particular object. In this tutorial, you'll see how to choose the best metric unit to measure the capacity of a given item.
-
How Do You Measure an Object in Inches Using a Ruler?
Measuring is a building block of math and understanding the world around us. This tutorial shows you how to measure an object in inches using a ruler.
-
How Do You Measure an Object to the Nearest Centimeter Using a Ruler?
Rulers help you measure all sorts of things, but you need to learn how to use it first! Follow along with this tutorial to see how to use a ruler to measure objects in centimeters.
-
How Do You Measure an Object in Millimeters Using a Ruler?
Knowing how to use a ruler is an important skill in both math and the real world! Follow along with this tutorial to see how to use a ruler to measure an object in millimeters!
-
What are the Customary Units of Length?
An inch, a foot, and a mile are all customary units used to measure the length of an object. This tutorial introduces you to the customary units for length.
-
What are the Customary Units of Weight?
There's more than one unit to measure the weight of an object! In this tutorial, you'll learn all about the customary units used to measure weight.
-
What are the Customary Units of Capacity?
You could use pints, quarts, or even gallons to measure the amount of milk in a container. Those units are all examples of customary units of capacity. This tutorial introduces these helpful units!
-
What are the Metric Units of Length?
If you're measuring the length of an object, you can use one of the metric units used to measure length. Follow along with this tutorial to learn about these metric units for length.
-
What are the Metric Units of Mass?
Milligrams and grams are two of the common metric units used to measure the mass of an object. In this tutorial, you'll learn about the metric units used to measure mass!
-
What are the Metric Units of Capacity?
A liter is a metric unit used to measure the capacity of an object, but there are other metric units that can also be used! This tutorial introduces the metric units for capacity.
-
What is Density?
Ever wonder why some objects float in water while others sink? The object's density plays a key role. This tutorial provides a great introduction to density.
-
How Do You Compare Two Decimals Using a Number Line?
If you have two decimals and you want to know which is larger, you can use a number line to help you compare! Follow along with this tutorial to see how to use a number line to compare decimals.
-
How Do You Round a Decimal Using a Number Line?
Rounding is an important part of math! In this tutorial, you'll see how to use a number line to help you round a decimal to a chosen place value.
-
How Do You Use Front-End Estimation to Estimate a Sum of Several Decimals?
Front-end estimation can be a great way to approximate a sum. In this tutorial, you'll see how to use front-end estimation to approximate the cost of school supplies!
-
How Do You Estimate a Sum of Decimals by Clustering?
If you want to find the approximate sum of decimals, you can round those numbers first! This tutorial shows you how to round decimals and see what numbers they're close to in order to make adding easier.
-
How Do You Regroup Numbers to Add Decimals Mentally?
Adding numbers mentally is easier than you might think! It can be a quick and fun skill! This tutorial shows you how to take decimals and add them mentally.
-
How Do You Multiply a Decimal by a Whole Number?
If you want to multiply a decimal by a whole number, just ignore the decimal point and multiply like normal! Then, bring the decimal point back for the answer. This tutorial shows you the entire process step-by-step.
-
How Do You Multiply a Decimal by a Whole Number Using Estimation to Place the Decimal?
When you multiply a decimal and a whole number, removing the decimal point can make multiplying easier, but where does the decimal point go when you want to get your answer? In this tutorial, you'll see how to estimate the location of the decimal point after you multiply!
-
How Do You Solve a Word Problem by Dividing with Decimals and Rounding Your Answer Up?
You never know where division might pop up! This word problem is a great example of using long division to solve a problem.
-
What are Decimals?
Have you ever looked at the price of something? It's usually given as a decimal! Decimals are a very helpful part of math and can be found in may places in the real world. Watch this tutorial to learn all about decimals!
-
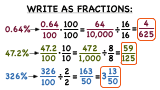
How Do You Write a Fraction as a Decimal?
Sometimes fractions can be easier to work with if they're in their decimal form. This tutorial shows you how to turn a fraction into a decimal with a few simple steps!
-
How Do You Write a Decimal as a Fraction?
You can turn a decimal into a fraction with a few simple steps! Follow along with this tutorial to learn how to write a decimal as a fraction!
-
How Do You Order Fractions and Decimals From Greatest to Least?
If you want to compare different types of numbers, start by converting all of them to one type of number! This tutorial shows you an example of this involving fractions and decimals!
-
How Do You Find a Missing Value to Make Two Fractions Equivalent?
It's important to know how to make equivalent fractions. In this tutorial, you'll see how to create an equivalent fraction when you have a fraction and the denominator of an equivalent fraction!
-
How Do You Write a Fraction in Simplest Form Using the GCF?
When you get a fraction as an answer, you're usually asked to write it in its simplest form. This tutorial shows you how to use the GCF of the numerator and denominator to fully simplify a fraction!
-
What is a Factor?
The term 'factor' is seen a lot in math, so it's important to know what it means. This tutorial introduces you to that term!
-
What is a Greatest Common Factor?
Being able to take out a greatest common factor can make a problem easier to work with, but before you do that, you need to understand what a greatest common factor is. This tutorial explains exactly that!
-
What is Simplest Form of a Fraction?
Are you ever asked to put your fraction answer into simplest form? Wonder what 'simplest form' means? This tutorial explores exactly that! Take a look at what a fraction needs in order to be in simplest form.
-
How Do You Add Two Fractions with the Same Denominator?
If you want to add fractions together, first make sure the fractions have the same denominator. If they do, then just add to numerators together to get the sum of the fractions! Follow along with this tutorial to see this process step-by-step.
-
How Do You Subtract Two Fractions with the Same Denominator?
To subtract fractions with the same denominators, just subtract the numerators! Follow along with this tutorial to see an example of subtracting fraction with the same denominators.
-
How Do You Add Two Fractions with Different Denominators?
If you're adding fractions with unlike denominators, you first need to get those denominators to be the same! This tutorial shows you how to write fractions so they have common denominators and then shows you how to add those fractions together.
-
How Do You Subtract Two Fractions with Different Denominators?
In order to subtract fractions, they must have the same denominators. To get the same denominators, you can find equivalent fractions! This tutorial shows you how to subtract fractions with unlike denominators.
-
What Does It Mean to Divide Fractions?
This tutorial gives an in-depth look at dividing fractions by showing you what it really means to divide them.
-
How Do You Figure Out a Tip?
If you need to leave a tip at a restaurant, you can quickly estimate the amount in your head! This tutorial shows you how to use estimation and mental math to estimate a tip!
-
How Do You Find Equivalent Ratios by Making a Table?
To master equivalent ratios, you need to practice. Follow along with this tutorial to practice filling in a table with equivalent ratios.
-
How Do You Use a Table of Equivalent Ratios to Predict a Value?
Patterns are everywhere! In this tutorial, you'll see how to use the pattern in a table to find an answer to a word problem.
-
How Do You Write Ratios From a Table?
This tutorial goes through the steps of creating ratios from a table of values! Follow along to see how the ratios are made!
-
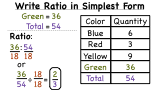
How Do You Write a Ratio in Simplest Form?
In this tutorial, you'll see how to take the information from a given table and use it to find a ratio. You'll also see how to write the answer in simplest form!
-
How Do You Estimate a Sale Price?
Sales are great, but how much are you really saving? This tutorial shows you how to estimate the sales price of an item.
-
How Do You Estimate a Percent?
When something is on sale, it's good to know how much you're saving! This tutorial shows you how to estimate a percent using an original price and a coupon!
-
How Do You Figure Out a Percent From a Part to Part Ratio?
Word problems and percents can be a fun combination! This tutorial shows you how to find the percent of something in a basket using ratios!
-
How Do You Figure Out a Percent From a Part to Whole Ratio?
If you want to find a percent in a word problem, you may be able to use a ratio to help you! This tutorial shows you how to do exactly that!
-
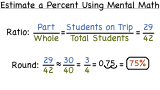
How Do You Use Mental Math to Estimate a Percent?
Mental math is a powerful tool! See how to use mental math in this tutorial to estimate a percent!
-
How do you Figure Out if Two Figures are Similar?
Follow along with this tutorial to see an example of determining if two given figures are similar.
-
What is a Degree?
Circles can't run a temperature, but they are an important part of degrees! Watch this tutorial to learn what a degree is and how it relates to a circle.
-
What are Intersecting Lines?
When two roads cross, it's called an intersection! What about if two lines cross? Is that called an intersection too? This tutorial has the answer!
-
How Do You Find the Perimeter of a Rectangle?
Finding the perimeter of a rectangle is as easy as adding up all its side lengths! This tutorial takes you through the steps needed to find the perimeter of a rectangle.
-
How Do You Find the Diameter of a Circle if You Know its Radius?
When you know the radius of a circle, you can find the diameter of that circle with a simple multiplication problem! Follow along with this tutorial to learn how to find the diameter of a circle when you're given the radius.
-
How Do You Find the Surface Area of a Rectangular Prism Using a Net?
Finding the surface area of a prism can be a little tricky, but a net can make the problem a little easier. Make your job easier and see how to use a net to find the surface area of a prism.
-
How Do You Find the Surface Area of a Cylinder Using a Net?
Nets can come in real handy, especially when you're fishing. Nets can be even more useful when you're trying to find the surface area of a three-dimensional solid! Follow along to see how a net can help you find the surface area of a cylinder!
-
What is Area?
Area is the amount of space a figure takes up! This tutorial introduces area and explains how we find the area of a figure.
-
What is the Formula for the Volume of a Rectangular Prism?
Make finding the volume of a rectangular prism a snap by using a formula! Check out this tutorial to learn more!
-
How Do You Find the Perimeter of a Rectangle in the Coordinate Plane?
Finding the perimeter of a rectangle in the coordinate plane is easier than you might think! This tutorial shows you how to use the coordinate plane to find the perimeter of a rectangle.
-
How Do You Graph a Rectangle on a Coordinate Plane?
If you want to graph a rectangle on the coordinate plane, just graph the vertices and then connect them! This tutorial takes you through this entire process step-by-step.
-
How Do You Find the Area of a Rectangle in the Coordinate Plane?
If you need to find the area of a rectangle on the coordinate plane, all you need is the length and width of that rectangle! This tutorial shows you what to do to find the area of a rectangle when it's graphed on the coordinate plane.
-
How Do You Find the Area of an Irregular Figure in the Coordinate Plane?
Finding the area of a composite figure in the coordinate plane is easier than you might think! Follow along with this tutorial to see the process step-by-step.
-
How Do You Represent Real World Situations Using Integers?
The real world has all sorts of math clues! See how to use math to represent real world situations by watching this tutorial:
-
What Does Absolute Value Mean In the Real World?
You may know how to calculate the absolute value of a number, but what are you really finding? This tutorial uses a real world example to help you gain a better understanding of absolute value.
-
How Do You Use a Line Graph to Make Predictions?
How can you use a line graph to make predictions about the future? This tutorial can show you:
-
How Do You Use a Circle Graph to Make Predictions?
Circle graphs are like a crystal ball that can help you predict the future! Let's see how to predict using a circle graph:
-
How Do You Interpret a Circle Graph?
Get a better understanding of circle graphs by watching this tutorial:
-
How Do You Interpret a Bar Graph With a Break in the Scale?
Want to practice interpreting a bar graph? Check out this tutorial:
-
What is a Double Bar Graph?
A double bar graph is like two graphs in one! Learn all about double bar graphs with this tutorial:
-
What is a Line Graph?
Want to see a line graph? Check out this tutorial! It introduces you to these neat graphs:
-
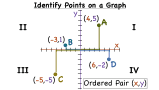
How Do You Identify Points on a Graph?
Plotting points on the coordinate plane is the foundation of graphing equations! But before you can graph equations, you should be very familiar with the coordinate plane. In this tutorial, you'll see how to identify the ordered pair of a point on the coordinate plane. Plus, see how to figure out which quadrant the point is in!
-
How Do You Graph Ordered Pairs in Each Quadrant?
Plotting points on the coordinate plane is the foundation for graphing equations! Check out this tutorial to get some practice plotting points and identifying which quadrant each point is in.
-
How Do You Add Integers Using a Number Line?
Combining integers? You could use a number line to help find the answer! In this tutorial, see how to use a number line to add together integers with the same sign and ones with opposite signs. Take a look!
-
What is Multiplication?
Multiplying integers is just like performing a bunch of additions of the same integer! Check out this tutorial and see how much multiplying and repeated addition have in common.
-
How Do You Subtract Integers Using a Number Line?
Subtracting integers? You could use a number line to help find the answer! In this tutorial, see how to use a number line to subtract integers with the same sign and ones with opposite signs. Take a look!
-
How Do You Put Integers in Order Using a Number Line?
Putting numbers in order can help you better understand how the numbers are related. This tutorial shows you how to put positive and negative temperatures in order using a number line!
-
How Do You Compare Integers Using a Number Line?
Trying to figure out if a negative number is larger than another can be a little tricky. To make things easier, you could use a number line! This tutorial shows you how to use a number line to compare two negative numbers and determine which is larger.
-
How Do You Do Long Multiplication?
Multiplying together large numbers? No problem! In this tutorial, you'll see how to perform long multiplication to find the answer (without using a calculator!). Take a look!
-
How Do You Determine Which Operations to Use in a Word Problem?
Turning a word problem into a math problem you can solve can be tricky. Luckily, there's some key words to look out for in a word problem that help tell you what math operation to use! This tutorial shows you some of these key words to look for in a word problem.
-
How Do You Translate Phrases into Numerical Expressions?
Turning a phrase from words to a math problem can be tricky, but practice can make this process easier! In this tutorial, you'll see how to look for key words that can help you translate a phrase into math.
-
What is Division?
Division is a stepping stone of math! In this tutorial, you'll see how cookies can help you find the answer to division problems!
-
What Are Numerical and Algebraic Expressions?
An expression is just a mathematical phrase. In this tutorial, you'll learn about two popular types of expressions: numerical and algebraic expressions. A numerical expression contains numbers and operations. An algebraic expression is almost exactly the same except it also contains variables. Check out this tutorial to learn about these two popular kinds of expressions!
-
What are the Rules for Using Absolute Values to Add Integers?
When you're combining numbers, there are some helpful rules to make that process a little easier. This tutorial shows you the rules for using absolute values to combine integers with the same sign or with opposite signs. Take a look!
-
What is the Opposite, or Additive Inverse, of a Number?
Have you ever combined two numbers together and found their sum to be zero? When that happens, those numbers are called additive inverses of each other! In this tutorial, you'll learn the definition for additive inverse and see examples of how to find the additive inverse of a given value.
-
What are Positive and Negative Numbers?
Positive and negative numbers (and zero!) are the building blocks of math. This tutorial will introduce you to positive and negative numbers and show you their location on a number line. Plus, learn a special thing about the number zero!
-
What is the Number Line?
A number line is a way we can visually represent numbers. This tutorial gives you a great introduction to the number line and shows you how to graph numbers on the number line in order to compare them. Check it out!
-
How Do You Solve a Two-Step Equation?
Becoming a pro at solving equations takes practice! Follow along with this tutorial to see an example of solving an equation for a variable.
-
What are Inverse Operations?
Did you know that addition is the opposite of subtraction and that division is the opposite of multiplication? In fact, these opposite operations will undo each other! These types of operations are called inverse operations. Get a great look at inverse operations with this tutorial!
-
What is an Equation?
In order to tackle the challenging topics, you first need to know the basics. Equations are the start of some very fun and challenging math problems. Watch this tutorial to learn about equations!
-
What is a Solution to an Equation?
When you get an equation, usually you want to solve it and find the answer. This tutorial explains what it means to find the solution to an equation. Take a look!
-
How Do You Find the Least Common Multiple by Listing Common Multiples?
Looking for practice finding the least common multiple (LCM)? Then be sure to check out this tutorial! Follow along with this tutorial as it goes through the process of listing multiples of given numbers and identifying the smallest of these multiples in order to find the LCM. Take a look!
-
How Do You Find the Least Common Multiple by Multiplying Common Factors?
Looking for practice finding the least common multiple (LCM)? Then be sure to check out this tutorial! Follow along with this tutorial as it goes through the process of using a factor tree for each given number in order to help find the LCM.
-
How Do You Put Fractions in Order Using an LCD?
Ordering fractions from least to greatest? Don't have common denominators? You could find the least common denominator (LCD) of the fractions and write equivalent fractions with this LCD. Then, compare the numerators to figure out their order from least to greatest! This tutorial shows you how!
-
How Do You Convert a Number in Expanded Form into Exponential Form?
Exponential form is a quick way to show that a number should be multiplied by itself a certain number of times. In this tutorial, see how to write a repeated multiplication in exponential form!
-
How Do You Convert a Number in Exponential Form into Expanded Form?
Did you know that exponents are just a quick way to show repeated multiplication? In this tutorial, see how to expand out a value in exponential form to see what it really represents!
-
How Do You Evaluate an Exponent?
Exponents just indicate repeated multiplication. Watch this tutorial to see how you can evaluate an exponent by first writing it in expanded form. Take a look!
-
How Do You Check if a Number is Divisible by 2, 3, 5, 6, and 10?
Learning about divisibility? Take a look at this tutorial! You'll see how to test if a number is divisible by 2, 3, 5, 6, and 10 using some cool tricks!
-
How Do You Find All the Factors of a Number?
Trying to find all the factors of a number? Setting up a table can be really helpful! Check out this tutorial to see how to use a table to find all the factors of a given number.
-
How Do You Factor a Greatest Common Factor Out of an Algebraic Expression?
The greatest common factor (GCF) is the largest factor two or more numbers have in common. Finding the GCF can be very useful in simplifying an expression or solving an equation. In this tutorial, see how to identify the GCF of an expression and factor it out. Check it out!
-
How Do You Find the Greatest Common Factor of Two Numbers by Listing Their Factors?
The greatest common factor (GCF) is the largest factor two or more numbers have in common. Finding the GCF can be very useful in simplifying an expression or solving an equation. Watch this tutorial and learn what it takes to find the GCF of two numbers!
-
How Do You Compare Fractions by Finding a Common Denominator?
Ordering fractions from least to greatest? Don't have common denominators? Find a common denominator by multiplying the denominators together. Use that common denominator to create equivalent fractions. Then, compare the numerators to figure out which is bigger! This tutorial shows you how!
-
What is a Multiple?
Being able to find multiples of a number is important, especially if you want to find the least common multiple (LCM) between numbers. In this tutorial, you'll be introduced to the term multiple. You'll also see how to find multiples of a given number!
-
What is a Common Multiple and Least Common Multiple?
Sometimes terms in math do a pretty good job of describing the thing they name. This is the case with common multiple and least common multiple (LCM). A common multiple is a multiple that two or more numbers have in common. You can probably guess what a least common multiple is! To get more information about these terms, check out this tutorial!
-
What are Equivalent Fractions?
Finding equivalent fractions is an important part of things like adding, subtracting, and comparing fractions. But what are they? In this tutorial, you'll learn that equivalent fractions are just fractions that have the same value, even though they may look very different! Take a look at equivalent fractions by watching this tutorial!
-
What Does Divisibility Mean?
Divisibility is an important part of math. When you're finding the factors of a number, you need to figure out what numbers you number is divisible by. Take a look at this tutorial and learn about divisibility!
-
How Do You Solve an Equation With Fractions With The Same Denominators Using Subtraction?
Looking for practice solving equations containing fractions? Then check out this tutorial! Follow along and see how to subtract fractions with common denominators in order to solve an equation for a variable.
-
How Do You Solve an Equation With Fractions With The Same Denominators Using Addition?
Looking for practice solving equations containing fractions? Then check out this tutorial! Follow along and see how to add fractions with common denominators in order to solve an equation for a variable.
-
How Do You Solve an Equation With Fractions With Different Denominators Using Subtraction?
Looking for practice solving equations containing fractions? In this tutorial, you'll see how to first convert a mixed fraction to an improper fraction and then subtract fractions with unlike denominators in order to solve an equation. Be sure to check you answers so you KNOW it's correct!
-
How Do You Solve an Equation With Fractions With Different Denominators Using Addition?
Looking for practice solving equations containing fractions? In this tutorial, see how to add fractions with unlike denominators in order to solve an equation. Then, be sure to check you answers so you KNOW it's correct!
-
How Do You Solve a Decimal Equation Using Subtraction?
Want to see how to solve an equation containing decimals? Then check out this tutorial! You'll see how to subtract decimals in order to solve an equation for a variable. Then, see how to check your answer so you can be certain it's correct!
-
How Do You Solve a Decimal Equation Using Addition?
Want to see how to solve an equation containing decimals? Then check out this tutorial! You'll see how to add decimals in order to solve an equation for a variable. Then, see how to check your answer so you can be certain it's correct!
-
How Do You Divide a Decimal by a Decimal?
Dividing decimals? Then this tutorial is a must see! Follow along and learn how you can divide decimals by rewriting the problem as a fraction and then using long division to solve. Check it out!
-
How Do You Solve a Decimal Equation Using Division?
Want to see how to solve an equation containing decimals? Then check out this tutorial! You'll see how to divide decimals in order to solve an equation for a variable. Then, see how to check your answer so you can be certain it's correct!
-
How Do You Solve a Decimal Equation Using Multiplication?
Want to see how to solve an equation containing decimals? Then check out this tutorial! You'll see how to multiply decimals in order to solve an equation for a variable. Then, see how to check your answer so you can be certain it's correct!
-
How Do You Add Mixed Fractions with the Same Denominator?
Adding mixed fractions? If they have common denominators, then you can add the whole numbers and fractions separately. In this tutorial, take a look at adding together mixed fractions!
-
How Do You Subtract Mixed Fractions with the Same Denominator?
Subtracting mixed fractions? If they have common denominators, then you can subtract the whole numbers and fractions separately. In this tutorial, take a look at subtracting mixed fractions!
-
How Do You Add Mixed Fractions with Different Denominators by Converting to Improper Fractions?
Adding mixed fractions? You could first convert each to an improper fraction. If they don't have common denominators, then find a common denominator and use it to rewrite each fraction. Then, add the fractions together and simplify. In this tutorial, take a look at adding together mixed fractions with unlike denominators!
-
How Do You Subtract Mixed Fractions with Different Denominators by Regrouping?
Subtracting mixed fractions with unlike denominators? You could first find a common denominator and use it to rewrite each fraction. Then, subtract the whole numbers and fractions separately. In this tutorial, take a look at subtracting mixed fractions with unlike denominators!
-
How Do You Subtract Mixed Fractions with Different Denominators by Converting to Improper Fractions?
Subtracting mixed fractions? You could first convert each to an improper fraction. If they don't have common denominators, then find a common denominator and use it to rewrite each fraction. Then, subtract the fractions and simplify. In this tutorial, take a look at subtracting mixed fractions with unlike denominators!
-
How Do You Multiply Mixed Numbers?
To multiply mixed fractions together, you could first convert each to an improper fraction. Then, multiply the fractions together, simplify, and convert your answer back to a mixed fraction. This tutorial will show you how!
-
How Do You Divide Fractions?
Dividing fractions? Change that division to a multiplication by multiplying the dividend by the reciprocal of the divisor. Learn all about it by watching this tutorial!
-
How Do You Divide Mixed Numbers?
To divide mixed fractions, you could first convert each to an improper fraction. Then, switch to a multiplication problem by multiply by the reciprocal of the divisor. Simplify and convert your answer back to a mixed fraction to get your answer! This tutorial will show you how!
-
How Do You Round a Decimal to a Given Place Value?
Sometimes, decimals are so long that you need a way to estimate the value of the decimal. Other times, you may only need a certain amount of exactness to get your answer. This is where rounding decimals to a chosen place can be very helpful! Watch this tutorial to learn how to round a decimal to a chosen place.
-
How Do You Turn a Fraction Into a Terminating Decimal?
Did you know that a fraction just represents a division? To turn a fraction into a decimal, divide the numerator by the denominator. In this tutorial, see how to convert a fraction into the terminating decimal it represents.
-
How Do You Turn a Terminating Decimal Into a Fraction?
If you have a terminating decimal, you can rewrite it as a fraction! Check out this tutorial to learn how to convert a terminating decimal into a fraction.
-
How Do You Turn a Fraction Into a Repeating Decimal?
Did you know that a fraction just represents a division? To turn a fraction into a decimal, divide the numerator by the denominator. In this tutorial, see how to convert a fraction into the repeating decimal it represents.
-
What is Place Value?
Did you know numbers have place values? This tutorial introduces you to the term place value and shows you some of the most seen place values. Take a look!
-
What is a Terminating Decimal?
A terminating decimal is a decimal that ends. It's a decimal with a finite number of digits. Did you know that all terminating decimals can be rewritten as fractions? Watch this tutorial to learn about terminating decimals and see some examples!
-
What is a Repeating Decimal?
A repeating decimal is a decimal that has a digit, or a block of digits, that repeat over and over and over again without ever ending. Did you know that all repeating decimals can be rewritten as fractions? To make these kinds of decimals easier to write, there's a special notation you can use! Learn about repeating decimals in this tutorial.
-
How Do You Find Equivalent Ratios?
Ratios are used to compare numbers. When you're working with ratios, it's sometimes easier to work with an equivalent ratio. Equivalent ratios have different numbers but represent the same relationship. In this tutorial, you'll see how to find equivalent ratios by first writing the given ratio as a fraction. Take a look!
-
How Do You Write a Ratio From a Word Problem?
Sometimes the hardest part of a word problem is figuring out how to turn the words into a math problem. In this tutorial, you'll see how to take the information given in a word problem and write a ratio. Then, reduce the ratio and explain its meaning. See it all in this tutorial!
-
How Do You Use Unit Rates to Compare Rates?
Word problems allow you to see the real world uses of math! This tutorial shows you how to use ratios to figure out which store has a better deal on cupcakes. Take a look!
-
How Do You Figure Out What Size a Model Should Be if You Have a Scale?
Before tall sky scrapers are build, a scale model of the building is made, but how does the architect know what size the model should be? Follow along with this tutorial to find out!
-
What are Equivalent Ratios?
Equivalent ratios are just like equivalent fractions. If two ratios have the same value, then they are equivalent, even though they may look very different! In this tutorial, take a look at equivalent ratios and learn how to tell if you have equivalent ratios.
-
What is a Scale?
In math, the term scale is used to represent the relationship between a measurement on a model and the corresponding measurement on the actual object. Without scales, maps and blueprints would be pretty useless. Check out this tutorial and learn about scale factor!
-
How Do You Put Fractions, Decimals, and Percents in Order?
Ordering numbers from least to greatest? Are the numbers in different forms? To make comparing easier, convert all the numbers to decimals. Then, plot those decimals on a number line and compare them! This tutorial shows you how!
-
How Do You Turn a Percent Into a Fraction?
Looking for some practice converting percents to fractions? Then this tutorial was made for you! Follow along as this tutorial shows you how to convert a percent to a fraction. Then, reduce the fraction to put it in simplest form. Check it out!
-
How Do You Turn a Fraction Into a Percent?
Looking for some practice converting fractions to percents? Then this tutorial was made for you! Follow along as this tutorial shows you how to convert a fraction to a percent.Take a look!
-
How Do You Turn a Decimal into a Percent?
Converting decimals into percents is easier than you may think! To convert a decimal to a percent, just move the decimal point to places to the right and put a percent sign at the end! To see it done, check out this tutorial!
-
How Do You Use a Proportion to Find a Whole?
Taking a percent of a number? Trying to figure out the result? Use a percent proportion to solve! This tutorial will show you how!
-
How Do You Use an Equation to Find a Whole?
Taking a percent of a number? Trying to figure out the result? Use a percent equation to solve! This tutorial will show you how!
-
How Do You Find Percents Mentally Using Fractions?
Some fractions are seen so often in math that it can be helpful to know the percent that goes with it. This tutorial shows you some common percent-fraction relationships!
-
How Do You Use Compatible Numbers to Estimate a Part of a Whole?
If you're trying to find the percent of a number, it may be helpful to use compatible numbers to find an estimated answer. Follow along with this tutorial to see how to use compatible numbers to estimate the percent of a number!
-
How Do You Make a Table of Values for a Linear Equation?
To graph a linear equation, you could make a table of values to plot, but first you'll need to know how to make the table. Take a look at this tutorial! You'll see how to set up a table, choose appropriate x-values, plug those values into the equation, and simplify to get the respective y-values. This tutorial shows you how to set up a table of values for a linear equation!
-
What is a Linear Equation?
If you're learning about graphs, you're bound to see a bunch of linear equations, so it's a good idea to understand what makes an equation a linear equation. This tutorial explains linear equations and shows you the difference between equations that are linear and ones that are not. Check it out!
-
What is Rate of Change?
Trying to describe the how something changes in relation to something else? Use rate of change! In this tutorial, learn about rate of change and see the difference between positive and negative rates of change!
-
How Do You Use Complementary Angles to Find a Missing Angle?
If two angles are complementary, that means that they add up to 90 degrees. This is very useful knowledge if you have a figure with complementary angles and you know the measurement of one of those angles. In this tutorial, see how to use what you know about complementary angles to find a missing angle measurement!
-
How Do You Use Supplementary Angles to Find a Missing Angle?
If angles combine to form a straight angle, then those angles are called supplementary. In this tutorial, you'll see how to use your knowledge of supplementary angles to set up an equation and solve for a missing angle measurement. Take a look!
-
How Do You Classify Quadrilaterals?
Got a figure with four sides? Then you have a quadrilateral! But there are many special types of quadrilateral. Follow along as this tutorial shows you how to figure out the possible names for a given quadrilateral!
-
How Do You Use a Graph to Reflect a Figure Over the Y-Axis?
Reflecting a figure over the y-axis can be a little tricky, unless you have a plan. In this tutorial, see how to use the graph of a figure to perform the reflection. Check it out!
-
How Do You Use a Graph to Reflect a Figure Over the X-Axis?
Reflecting a figure over the x-axis can be a little tricky, unless you have a plan. In this tutorial, see how to use the graph of a figure to perform the reflection. Check it out!
-
How Do You Classify Triangles?
Being able to classify triangles is an important skill. If you know what kind of triangle you're looking at, it's much easier to figure out how to solve for various sides and angles. Practice classifying triangles with this tutorial!
-
How Do You Find a Missing Angle in a Triangle?
Trying to find a missing interior angle measurement in a triangle? Already know the other two interior angle measurements? Then you're set! Just remember that the interior angles of a triangle ALWAYS add up to 180 degrees. This tutorial shows you how to put this knowledge into an equation and solve to find that missing measurement!
-
How Do You Identify Corresponding Parts in Congruent Triangles?
When you have two congruent figures, that means that corresponding sides and corresponding angles are congruent. Get some practice identifying corresponding sides and angles by following along with this tutorial!
-
How Do You Use a Graph to Translate a Figure Horizontally?
Translating a figure on the coordinate plane is easier than you might think! In this tutorial, see how to use the graph of the original figure to perform the translation. Take a look!
-
How Do You Use a Graph to Translate a Figure Vertically?
Translating a figure on the coordinate plane is easier than you might think! In this tutorial, see how to use the graph of the original figure to perform the translation. Take a look!
-
What is a Rectangle?
A rectangle is one of the many fundamental shapes you'll see in math. Rectangles have special properties that can be very useful in helping you solve a problem. This tutorial introduces you to rectangles and explains their interesting qualities!
-
What is a Triangle?
You can't learn everything about math without dealing with triangles. Did you know that there are different types of triangles? Check out this tutorial, and learn about triangles and their different types!
-
What is a Parallelogram?
A parallelogram is a special type of quadrilateral with some special properties. In this tutorial, take a look at parallelograms and learn what kinds of quadrilaterals can also be called parallelograms!
-
What is a Trapezoid?
A trapezoid is a special type of quadrilateral with some special properties. This tutorial introduces you to trapezoids and gives you a look at the special properties needed for a quadrilateral to be called a trapezoid. Check it out!
-
What is a Point?
A point is a fundamental building block of math. Without points, you couldn't make lines, planes, angles, or polygons. That also means that graphing would be impossible. Needless to say, learning about points is very important! That makes this tutorial a must see!
-
What is a Plane?
You can't learn everything about math without seeing planes. Did you know that there are rules for naming a plane? This tutorial introduces you to planes and shows you how to name them. Take a look!
-
What is a Line Segment?
A math term can really tell you a lot about the thing it's describing. Take the term line segment. A line segment is just part of a line! In this tutorial, learn about line segments, how to name them, and what the midpoint of a line segment is!
-
What is a Ray?
Rays are a very useful part of math. Two rays can create an angle. Multiple angles can create a polygon. Add another dimension, and you get three-dimensional solids! This tutorial introduces you to rays and shows you how to name them.
-
What is an Angle?
Angles are a fundamental building block for creating all sorts of shapes! In this tutorial, learn about how an angle is formed, how to name an angle, and how an angle is measured. Take a look!
-
What is a Polygon?
Got a closed figure with three or more sides? Then you have a polygon! In this tutorial, you'll learn about the properties of a polygon, see the names of the most popular polygons, and learn how to identify polygons. Check it out!
-
What are Acute, Obtuse, Right, and Straight Angles?
Did you know that there are different kinds of angles? Knowing how to identify these angles is an important part of solving many problems involving angles. Check out this tutorial and learn about the different kinds of angles!
-
What are Complementary Angles?
Do complementary angles always have something nice to say? Maybe. One thing complementary angles always do is add up to 90 degrees. In this tutorial, learn about complementary angles and see how to use this knowledge to solve a problem involving these special types of angles!
-
What are Supplementary Angles?
Knowing about supplementary angles can be very useful in solving for missing angle measurements. This tutorial introduces you to supplementary angles and shows you how to use them to solve for a missing angle measurement. Take a look!
-
What are Vertical Angles?
Vertical angles have a very special quality. They are always congruent to one another! Check out this tutorial to learn about and see how to identify vertical angles!
-
What Does Congruent Mean?
If two figures have the same size and shape, then they are congruent. The term congruent is often used to describe figures like this. In this tutorial, take a look at the term congruent!
-
What are Parallel Lines?
Lines that are parallel have a very special quality. Without this quality, these lines are not parallel. In this tutorial, take a look at parallel lines and see how they are different from any other kind of lines!
-
What are Perpendicular Lines?
Perpendicular lines have a special property. The angles formed by perpendicular lines will always be the same. Check out this tutorial to learn about perpendicular lines and see a cool trick involving these special lines!
-
What are Skew Lines?
Ever heard of skew lines? They're pretty cool! Take a look at this tutorial and you'll be introduced to skew lines.
-
What is a Transversal?
Ever heard of a transversal? It's not as confusing as the term sounds. This tutorial will introduce you to transversals and show you the neat things that happen when a transversal meets two parallel lines. Take a look!
-
What are Acute, Obtuse, and Right Triangles?
Did you know that there are different kinds of triangles? Knowing how to identify these triangles is an important part of solving many problems involving these triangles. Check out this tutorial and learn about some of the different kinds of triangles!
-
What is a Quadrilateral?
The term quadrilateral is a really fancy sounding name for a certain kind of polygon. Did you know that there are special types of quadrilaterals? Watch this tutorial to learn about quadrilaterals and their special types.
-
What is a Square?
A square is one of the many fundamental shapes you’ll see in math. Squares have special properties that can be very useful in helping you solve a problem. This tutorial introduces you to squares and explains their interesting qualities!
-
What is a Rhombus?
A rhombus is a special kind of quadrilateral. Knowing about the special properties of a rhombus is important to identifying and using these special polygons. This tutorial introduces you to the rhombus and explains its unique qualities. Take a look!
-
What is a Regular Polygon?
Polygons come in all different shapes and sizes. Some polygons have special properties that allow them to be called regular polygons. In this tutorial, you'll see what it takes for a polygon to be given this special name. Check it out!
-
What are Equilateral, Isosceles, and Scalene Triangles?
Did you know that there are different kinds of triangles? Knowing how to identify these triangles is an important part of solving many problems involving these triangles. Check out this tutorial and learn about some of the different kinds of triangles!
-
What is a Reflection?
When you look in the mirror, you see your reflection. In math, you can create mirror images of figures by reflecting them over a given line. This tutorial introduces you to reflections and shows you some examples of reflections. Take a look!
-
What is a Rotation?
Ever turned a door handle? You were performing a rotation! In math, rotations are just the same! Check out this tutorial to learn about rotations.
-
What is a Translation?
Ever slide something across a table? If so, then you have performed a translation! In this tutorial, learn the definition of translation and see some really neat examples. Take a look!
-
What is a Line of Reflection?
Just about everything in math has a name! Did you know that the line you reflect a figure over has a special name? It's called the line of reflection! Watch this tutorial and learn about the line of reflection.
-
What is a Transformation?
Transformations can be really fun! They allow you to change or move a figure. In this tutorial, learn about all the different kinds of transformations!
-
What is an Image?
Just about everything in math has a name! Did you know that when you're dealing with transformations, the new figure you get is called an image? Check out this tutorial and learn about this math term!
-
What is Rotational Symmetry?
Ever notice that some shapes look the same after you rotate them? These shapes have a property called rotational symmetry! Check out this tutorial to learn more.
-
What is the Triangle Sum Theorem?
When you're dealing with triangles, the Triangle Sum theorem can be very useful in finding interior angle measurements. In this tutorial, learn how to find this helpful theorem!
-
How Do You Find the Area of a Rectangle?
To find the area of a rectangle, multiply the length times the width! This tutorial will show you how to find the area of a rectangle. Check it out!
-
How Do You Find the Area of a Triangle?
Finding the area of a triangle? Know the length of the base and the height? Then just take those values and plug them into the formula for the area of a triangle and solve! This tutorial shows you how.
-
How Do You Find the Area of a Parallelogram?
Looking for the area of a parallelogram? Got the length of the base and the height? Then plug those values into the formula for the area of a parallelogram and solve. This tutorial takes you through the process!
-
How Do You Find the Area of a Trapezoid?
Want to find the area of a trapezoid? If you have the length of each base and the height, you can use them to find the area. In this tutorial, you'll see how to identify those values and plug them into the formula for the area of a trapezoid. Then see how to simplify to get your answer!
-
How Do You Find the Area of a Composite Figure?
Composite figures are just a combination of simpler figures in disguise! In this tutorial, you'll see how to break down a composite figure into simpler figures. Then, see how to find the area of each of those individual figures to find the area of the entire composite figure. Watch the whole process in this tutorial!
-
How Do You Find the Circumference of a Circle if You Know the Diameter?
Trying to find the circumference of a circle? Know the diameter? Then you can use the formula for the circumference of a circle to get the answer! Just plug the value for the diameter into the formula and solve. This tutorial shows you how!
-
How Do You Find the Circumference of a Circle if You Know the Radius?
Trying to find the circumference of a circle? Know the radius? Then you can use the formula for the circumference of a circle to get the answer! Just plug the value for the radius into the formula and solve. This tutorial shows you how!
-
How Do You Find the Area of a Circle if You Know the Radius?
If you know the radius of a circle, you can use it to find the area of that circle. Just plug that value into the formula for the area of a circle and solve. Watch this tutorial to see how it's done!
-
How Do You Find the Area of a Circle if You Know the Diameter?
If you have the diameter of a circle, you can use it to find the area of that circle. Just plug that value into the formula for the area of a circle and solve. Watch this tutorial to see how it's done!
-
How Do You Find the Volume of a Rectangular Prism?
Finding the volume of a rectangular prism isn't so bad, especially if you already know the length, width, and height. In this tutorial, you'll see how to use that information and the formula for the volume of a rectangular prism to get the answer. Check it out!
-
How Do You Find the Volume of a Triangular Prism?
Finding the volume of a triangular prism isn't so bad, especially if you already know the length and height of the base and the height of the prism. In this tutorial, you'll see how to use that information and the formula for the volume of a triangular prism to get the answer. Take a look!
-
What is a Circle?
Circles are a fundamental part of math! In this tutorial, you'll be introduced to circles and see the different parts of a circle such as the diameter, radius, and chord. Check out this tutorial to learn about circles!
-
What is Pi?
Some numbers are just so cool that they get their own tutorial. Take the irrational number pi. This number is simply a ratio, but people have been working for years to find more and more digits of pi. Check out this tutorial to learn about pi!
-
What is a Prism?
The term prism is a cool name for a special kind of three-dimensional solid. This tutorial defines the term prism and shows you how to name a prism using the shape of its bases. Check it out!
-
What is a Pyramid?
You've probably seen pictures of Egyptian pyramids, or maybe you've even seen them in person! Those pyramids mostly have either triangular or rectangular bases, but did you know that there are other types of pyramids? In this tutorial, you'll see what a three-dimensional solid needs to be called a pyramid. You'll also see how to name these pyramids, so take a look!
-
What is a Solid?
Understanding solids is a building block for finding their lateral area, surface area, and volume. In this tutorial, you'll see examples of solids and learn their different parts. Take a look!
-
What is a Sphere?
Every played with a bouncy ball, a volleyball, a basketball, or a baseball? Those are all spheres! Check out this tutorial to see what defines a sphere and learn its different parts. Take a look!
-
What is a Cylinder?
Soda cans, coffee cans, and some candles are just a few examples of cylinders. In this tutorial, you'll see what defines a cylinder. You'll also see the different parts of a cylinder. Take a look!
-
What is a Cone?
Did you know that an ice cream cone is named for its shape? Many ice cream cones are actually cones! Check out this tutorial to see what defines a cone in math. You'll also see the different parts of a cone. Take a look!
-
What is a Net?
Ever wonder what a box would look like if you unfolded and flatted it? That new picture would be called a net! This tutorial introduces you to nets, a two-dimensional version of a three-dimensional solid. Check it out!
-
What is Volume?
When you fill a jar with marbles or fill a pool with water, you are taking up volume! In this tutorial, you’ll be introduced to volume and learn what it really means. Take a look!
-
What is the Formula for the Area of a Rectangle?
Trying to find the area of a rectangle? There's a formula that can help! Check out this tutorial to learn about the formula for the area of a rectangle.
-
What is the Formula for the Area of a Triangle?
Did you know that the formula for the area of a triangle can be found by using the formula for the area of a parallelogram? In this tutorial, you'll see how it's done! Take a look!
-
What is the Formula for the Area of a Parallelogram?
Parallelograms and rectangles are pretty similar. In fact, you can turn a parallelogram into a rectangle to find the formula for the area of a parallelogram! Check out this tutorial to see how it's done!
-
What is the Formula for the Area of a Trapezoid?
Trying to figure out the formula for the area of a trapezoid? You could start by creating a parallelogram out of two trapezoids. Then, use the formula for the area of a parallelogram to figure out the formula for the area of one trapezoid. This tutorial shows you how!
-
What is Circumference?
The circumference of a circle is the distance around that circle. But what is the formula to find the circumference? In this tutorial, you'll learn the formulas for the circumference of a circle. Take a look!
-
What is the Formula for the Area of a Circle?
Did you know that you can figure out the formula for the area of a circle by first turning the circle into a parallelogram? It seems a little weird, but it really works! Watch this tutorial to see how it's done!
-
What is the Formula for the Volume of a Prism?
Trying to find the volume of a prism? Did you know that there's a formula to find that volume? In this tutorial, you'll learn about the formula for the volume of a prism. Check it out!
-
How Do You Determine All the Possible Outcomes of an Experiment?
When you perform an experiment, how do you figure out all the possible outcomes? Follow along with this tutorial to see!
-
What is an Unbiased Sample?
When you're trying to learn about a population, it can be helpful to look at an unbiased sample. An unbiased sample can be an accurate representation of the entire population and can help you draw conclusions about the population. This tutorial introduces you to unbiased sampling!