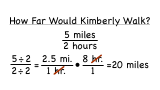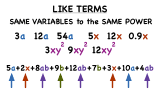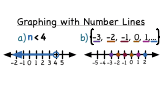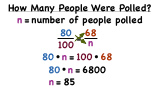Grade 7

More Specific Topics in Grade 7

Popular Tutorials in Grade 7

What are the Multiplication Properties of 0 and -1?

Understanding the multiplication properties of 0 and -1 are fundamental building blocks in learning all there is to know about the operation of multiplication. In this tutorial, you'll learn about these two important properties.
What are the Inverse Properties of Addition and Multiplication?

Inverse properties of addition and multiplication got you stumped? This tutorial should help! Check it out and learn these two important inverse properties.
How Do You Solve a Word Problem Using Unit Rates?

Word problems allow you to see the real world uses of math! This tutorial shows you how to take a rate and convert it to a unit rate. Then, you can use that unit rate to calculate your answer. Watch this tutorial to learn all about it!
How Do You Convert a Rate To A Unit Rate?

When you talk about the speed of a car, you usually say something in miles per hour. For example, you say, 'I drove 40 miles per hour.' Normally, you don't say, 'I drove 120 miles per 3 hours.' Figure out how to convert a rate like 120 miles per 3 hours to the unit rate of 40 miles per hour by watching this tutorial.
What are Rates and Unit Rates?

Can you do 100 sit-ups in 2 minutes? That's a rate! Driving a car going 40 miles per hour? That's a unit rate! Watch this tutorial to learn about rate and unit rate (and the difference!).
What is a Scale Drawing?

Without a blueprint, it would be really hard to construct a building. Without a road map, you'd be lost! Scale drawings make it easy to see large things, like buildings and roads, on paper. Even a GPS uses scale drawings! Check out this tutorial to learn all about scale drawings.
How Do You Find the Constant of Variation from a Direct Variation Equation?

The constant of variation is the number that relates two variables that are directly proportional or inversely proportional to one another. Watch this tutorial to see how to find the constant of variation for a direct variation equation. Take a look!
How Do You Write an Equation for Direct Variation Given a Point?

Looking for some practice with direct variation? Watch this tutorial, and get that practice! This tutorial shows you how to take given information and turn it into a direct variation equation. Then, see how to use that equation to find the value of one of the variables.
How Do You Write an Equation for Direct Variation from a Table?

Looking for some practice with direct variation? Watch this tutorial, and get that practice! This tutorial shows you how to take a table of values and describe the relation using a direct variation equation.
What's the Constant of Variation?

The constant of variation is the number that relates two variables that are directly proportional or inversely proportional to one another. But why is it called the constant of variation? This tutorial answers that question, so take a look!
What Does Direct Variation Look Like on a Graph?

Want to know what a direct variation looks like graphically? Basically, it's a straight line that goes through the origin. To get a better picture, check out this tutorial!
What are Multiplicative Inverses?

Multiplicative inverses. That's a mouthful! Really, this term just refers to numbers that when multiplied together equal 1. These numbers are also called reciprocals of each other! Learn about multiplicative inverses by watching this tutorial.
How Do You Use a Tree Diagram to Count the Number of Outcomes in a Sample Space?

When you're trying to figure out all the possibilities from different options, it can be helpful to make a tree diagram. In this tutorial, you'll see how to use a tree diagram to figure out how many different outfits can be created from the possible shirts, bottoms, and shoes given. Check it out!
How Do You Find the Probability of a Simple Event?

Working with probabilities? Check out this tutorial! You'll see how to calculate the probability of picking a certain marble out of a bag.
How Do You Find the Probability of Independent Events?

Calculating probabilities? Take a look at this tutorial and see how to figure out the probability of independently drawing certain cards from a deck!
How Do You Find the Probability of Dependent Events?

Sometimes probabilities depend on the outcomes of other events. Check out this tutorial to see probabilities of dependent events in action!
How Do You Find the Mode of a Data Set?

The mode of a data set is the number that occurs most frequently in the set. To easily find the mode, put the numbers in order from least to greatest and count how many times each number occurs. The number that occurs the most is the mode! Follow along with this tutorial and see how to find the mode of a set of data.
How Do You Find the Range of a Data Set?

The range of a data set is the difference between the largest number and the smallest number. In this tutorial, you'll see how to find the range of a set of data. Check it out!
How Do You Determine If Events are Independent or Dependent?
How Do You Find a Number of Combinations Using the Fundamental Counting Principle?

In this word problem, you'll see how to use the Fundamental Counting Principle to find the number of possible lunch combinations! Take a look!
How Do You Use a Simulation to Solve a Problem?

Simulators are a great way to model an experiment without actually performing the experiment in real life. This tutorial looks at using a simulator to figure out what might happen if you randomly guessed on a true/false quiz.
How Do You Compare Two Data Sets Using Box-and-Whisker Plots?
What is an Outcome?

When you're conducting an experiment, the outcome is a very important part. The outcome of an experiment is any possible result of the experiment. Learn about outcomes by watching this tutorial!
What is a Sample Space?

In an experiment, it's good to know your sample space. The sample space is the set of all possible outcomes of an experiment. Watch this tutorial to get a look at the sample space of an experiment!
What is Probability?

Probability can help you solve all sorts of everyday problems, but first you need to know what probability is! Follow along with this tutorial to learn about probability!
What are Compound Events?
What is the Median of a Data Set?

The median is one of many measures of central tendency. Check out this tutorial to learn what the median is and how you can find it!
What is the Mode of a Data Set?

Finding the mode of a set of data can help you understand the data better. This tutorial introduces you to mode and shows you how to find this helpful measure of central tendency!
What is the Mean of a Data Set?

When you think of the mean of a data set, think of the word average. 'Mean' and 'average' are the same thing when you're talking about a set of data! This tutorial introduces you to mean and shows you how to find it!
What is Experimental Probability?

Do real life situations always work out the way your mathematical models tell you they should? No! This tutorial describes how experimental probability differs from theoretical probability.
What are Like Terms?

Combining like terms together is a key part of simplifying mathematical expressions, so check out this tutorial to see how you can easily pick out like terms from an expression
What is the Distributive Property?

The distributive property is a very deep math principle that helps make math work. It's the rule that lets you expand parentheses, and so it's really critical to understand if you want to get good at simplifying expressions. So check out the tutorial and let us know what you think!
How Do You Do Long Division with Decimals?

We know that calculators are everywhere, but that doesn't mean that long division isn't important! Sometimes you won't be allowed to use a calculator, and when those times occur, you'll be thankful that you watched this video!
How Do You Solve a Word Problem That Compares Two Fractions?

Got a word problem where you're comparing fractions? No sweat! See how to translate a word problem into a mathematical expression, simplify it, and compare the resulting fractions using the cross products. This tutorial lays it all out step-by-step!
How Do You Subtract Fractions with Different Denominators?

Subtracting fractions with unlike denominators doesn't have to be a nightmare. Just find a common denominator and everything calms down! See how to keep your fraction subtraction calm with this tutorial.
How Do You Subtract Fractions with the Same Denominator?

Subtracting fractions with like denominators? Just subtract the numerators and put the result over the common denominator! To see this process in action, check out this tutorial!
How Do You Multiply and Divide Numbers with Different Signs?

A positive times a positive is a positive. A negative times a negative is a positive. What about a negative times a positive? This tutorial gives you the answer by showing you how multiply (and divide!) with mixed signs.
How Do You Multiply Fractions?

Working with fractions can be intimidating, but if you arm yourself with the right tools, you'll find that working with fractions is no harder than working with basic numbers. In this tutorial you'll see the process for multiplying 3 very simple fractions. Enjoy!
How Do You Add Fractions with Different Denominators?

Trying to add fractions with unlike denominators? You're going to need a common denominator first! Follow along with this tutorial and see what you need to do to add these fractions together.
How Do You Add a Negative Number to a Positive Number?

How do you combine a positive and a negative number? This tutorial shows you how. You even get to see it explained with a number line!
How Do You Multiply a Whole Number by a Fraction?

Multiplying a whole number and a fraction can be confusing, but this tutorial helps to sort things out. Check it out!
How Do You Figure Out the Sign of a Product or Quotient?

Multiplying and dividing numbers takes a good amount of thinking, and it's easy to make a mistake. But you can make sure that you're on the right track if you check whether the answer should be positive or negative. In this tutorial you'll see exactly how to tell if your answer will be positive or negative, even if you don't know the exact value of the answer. That way you'll always be able to check your answers!
How Do You Add Fractions with the Same Denominator?

While adding fractions can be hard, adding fractions with the same denominator is just as easy as adding numbers. That's why when you add fractions you first get all of them to have the same denominator, and then add them up. In this tutorial you get to see just how easy it is to add up fractions once they have the same denominator!
How Do You Subtract a Positive Number from a Negative Number?

Subtracting a positive from a negative? Just remember: subtracting a positive is the same as adding a negative. See how it works in this tutorial!
How Do You Subtract a Negative Number from a Positive Number?

Subtracting a negative from a positive? Just remember: subtracting a negative is the same as adding a positive. See how it works in this tutorial!
How Do You Graph an Inequality or an Infinite Set on a Number Line?

Number lines are really useful in visualizing an inequality or a set. In this tutorial, you'll see how to graph both. Take a look!
What's a Rational Number?

There are lots of different kind of numbers that you should know about, and that includes rational numbers. Check out the tutorial!
How Do You Simplify a Fraction Over a Whole Number?

Complex fractions are, well, complex. But if you watch this tutorial, you'll see how to make these complex fractions much simpler!
What's a Complex Fraction?

Ever wondered what makes complex fractions so complex? Check out this video tutorial and wonder no more :)
What's the Reciprocal Rule of Division?

When you divide fractions, the trick is to rewrite division as a multiplication. But the truth is that you can always rewrite division as a multiplication, and in this tutorial you'll see the rule that makes that possible!
How Do You Simplify a Fraction Over a Fraction?

Complex fractions can be pretty complex. Luckily, you can simplify a complex fraction to make it much easier to work with. See how in this tutorial!
What's a Reciprocal?

Reciprocals are important when it comes to dividing fractions, finding perpendicular lines, dealing with inverse proportions, and so much more! In this tutorial you can review the basics about reciprocals.
How Do You Add and Subtract a Bunch of Numbers with Different Signs?

Some problems require adding and subtracting a combination of positive and negative numbers. Watch this tutorial and learn how to keep everything organized so you can find the answer!
How Do You Rewrite Subtraction as Addition?

Subtracting a positive is the same thing as adding a negative. Subtracting a negative is the same as adding a positive. Get a closer look with this tutorial!
How Do You Add Two Negative Numbers?

Adding two negative numbers together? Just add the absolute value of each number together, put a negative sign in front, and you have your answer! See how it's done in this tutorial.
How Do You Solve a Word Problem Where You Multiply and Subtract Whole Numbers and Fractions?

Word problems are a great way to see math in the real world. In this tutorial, you'll see how to translate a word problem to a mathematical equation. Then, see how to use the order of operations to get the answer!
How Do You Solve a Word Problem Where You Divide Fractions?

Working with word problems AND fractions? This tutorial shows you how to take a word problem and translate it into a mathematical equation involving a complex fraction. Then, you'll see how to simplify the complex fraction to get the answer. Check it out!
How Do You Subtract a Whole Number from a Fraction?

Subtracting a whole number from a fraction can be tricky. Luckily, watching this tutorial can make this subtraction no big deal!
How Do You Subtract Decimals?

Doing math with paper and pencil can come in real handy, so make sure you're comfortable subtracting decimals by hand. After all, you don't want the calculator to be a crutch!
How Do You Add Decimals?

Doing math with paper and pencil can come in real handy, so make sure you're comfortable adding decimals by hand. After all, you don't want the calculator to be a crutch!
How Do You Multiply Decimals?

Doing math with paper and pencil can come in real handy, so make sure you're comfortable multiplying decimals by hand. After all, you don't want the calculator to be a crutch!
How Do You Distribute With Whole Numbers and Fractions?

This tutorial shows you how to distribute a whole number into the sum of fractions. It's an important skill to have when you're solving equations, and you never know when it can come up. Be sure to check out this tutorial!
How Do You Solve a Word Problem with an Equation Using Addition?

Word problems are a great way to see math in action! See how to translate a word problem into an equation, solve to find the answer, and check your found answer all in this tutorial.
How Do You Solve a Word Problem with an Equation Using Subtraction?

Word problems are a great way to see math in action! See how to translate a word problem into an equation, solve to find the answer, and check your found answer all in this tutorial.
How Do You Solve a Word Problem Using an Equation Where You're Multiplying Fractions?

Working with word problems AND fractions? This tutorial shows you how to take a word problem and translate it into a mathematical equation involving fractions. Then, you'll see how to solve and get the answer. Check it out!
How Do You Solve a Word Problem with an Equation Using Multiplication?

Working with word problems AND fractions? This tutorial shows you how to take a word problem and translate it into a mathematical equation involving fractions. Then, you'll see how to solve and get the answer. Check it out!
How Do You Solve a Word Problem with an Equation Using Division?

Word problems are a great way to see math in action! See how to translate a word problem into an equation, solve to find the answer, and check your found answer all in this tutorial.
How Do You Find the Length of a Rectangle if You Know its Width and Area?

How do you find the length of a rectangle if you're given the width and the area? This tutorial shows you how!
How Do You Use an Equation with Consecutive Numbers to Solve a Word Problem?

Word problems are a great way to see math in the real world. In this tutorial, you'll see how to translate a word problem into a mathematical equation involving consecutive numbers. Then you'll see how to solve that equation and check your answer!
How Do You Solve a Word Problem Where You Multiply Fractions and Work Backwards?

Word problems are a great way to see math in the real world. In this tutorial, you'll see how to solve a word problem by working backwards using a table. Check it out!
How Do You Solve a Word Problem Using a Two-Step Equation with Decimals?

Word problems are a great way to see math in the real world. In this tutorial, you'll see how to translate a word problem into a mathematical equation. Then you'll see how to solve that equation and check your answer!
How Do You Solve a Word Problem Using a Percent Proportion?

Word problems allow you to see the real world uses of math! This tutorial shows you how to take a words problem and turn it into a percent proportion. Then see how to solve for the answer using the mean extremes property of proportions. Take a look!
How Do You Set Up a Proportion from a Word Problem?

Sometimes the hardest part of a word problem is figuring out how to turn the words into an equation you can solve. This tutorial let's you see the steps to take in order to turn a word problem involving a blueprint into a proportion. Take a look!
How Do You Use a Proportion to Find a Part of a Whole?

Taking a percent of a number? Trying to figure out the result? Use a percent proportion to solve! This tutorial will show you how!
How Do You Use the Formula for Simple Interest?

If you already have a bank account or if you plan to have one in the future, then this tutorial is a must see! Follow along as this tutorial goes through a word problem involving simple interest.
What is the Formula for Simple Interest?

Interest is found in a bunch of places: savings accounts, mortgages, loans, investments, credit cards, and more! Watch this tutorial and learn how to calculate simple interest!
How Do You Figure Out a Percent of Change?

Word problems allow you to see the real world uses of math! In this tutorial, learn how to calculate the percent of increase using the percent of change formula.
What's a Percent of Change?

Lots of things in this world change their value such as cars, video games, and computers. When something either increases or decreases in value, it can be useful to know the percent of that change in value. To figure out that percent, you'll need the percent of change formula. Learn it with this tutorial!
How Do You Figure Out How Much Something is Marked Down?

Going shopping? Is something you want on sale? Trying to figure out the sale price of that item? Follow along with this word problem and you'll see how to calculate that price!
How Do You Set Up a Percent Proportion from a Word Problem?

Sometimes the hardest part of a word problem is figuring out how to turn the words into an equation you can solve. This tutorial let's you see the steps to take in order to do just that! Take a look! You'll be glad you did!
How Do You Figure Out Sales Tax?

Going shopping can be tons of fun, but things can go sour when you get to the register and realize that the sales tax puts you over your budget. Always stay under budget by figuring out your total cost BEFORE you hit the check out. Watch this tutorial and learn how to calculate sales tax!
How Do You Use an Equation to Find a Part of a Whole?

Taking a percent of a number? Trying to figure out the result? Convert the percent to a decimal and multiply it by the number! This tutorial will show you how!
How Do You Solve a Word Problem Using the Direct Variation Formula?

Word problems allow you to see math in action! Take a look at this word problem involving an object's weight on Earth compared to its weight on the Moon. See how the formula for direct variation plays an important role in finding the solution. Then use that formula to see how much you would weigh on the Moon!
What's the Direct Variation or Direct Proportionality Formula?

Ever heard of two things being directly proportional? Well, a good example is speed and distance. The bigger your speed, the farther you'll go over a given time period. So as one variable goes up, the other goes up too, and that's the idea of direct proportionality. But you can express direct proportionality using equations, and that's an important thing to do in algebra. See how to do that in the tutorial!
How Do You Use the Formula for Direct Variation?

If two things are directly proportional, you can bet that you'll need to use the formula for direct variation to solve! In this tutorial, you'll see how to use the formula for direct variation to find the constant of variation and then solve for your answer.
How Do You Solve a Proportion Using Cross Products?

Want to solve a percent proportion? Just use the means extremes property of proportions to cross multiply! Solve for the variable, and you have your answer! Learn how with this tutorial.
How Do You Use a Proportion to Find What Percent a Part is of a Whole?

A part is some percent of a whole. Trying to calculate the percent? Use a percent proportion to solve! This tutorial will show you how!
What's a Proportion?

The idea of proportions is that a ratio can be written in many ways and still be equal to the same value. That's why proportions are actually equations with equal ratios. This is a bit of a tricky definition, so make sure to watch the tutorial!
What's the Means-Extremes Property of Proportions?

The means-extremes property of proportions allows you to cross multiply, taking the product of the means and setting them equal to the product of the extremes. This property comes in handy when you're trying to solve a proportion. Watch this tutorial to learn more!
What are the Means and Extremes of Proportions?

A proportion is just an equation where two ratios are equal, and each piece of the proportion has a special name. This tutorial will teach you those names, and this will help you understand cross multiplication when you learn it later!
How Do You Solve a Word Problem Using a Proportion?

This tutorial provides a great real world application of math. You'll see how to use the scale from a blueprint of a house to help find the actual height of the house. This tutorial shows you how to use a proportion to solve!
How Do You Use Subtraction to Solve an Inequality Word Problem?

Word problems allow you to see the real world uses of math! In this tutorial, learn how to translate a word problem into an inequality. Then see how to solve the inequality and understand the meaning of the answer.
How Do You Use Addition to Solve an Inequality Word Problem?

Word problems allow you to see math in action! This tutorial deals with inequalities and money in a bank account. See how to translate a word problem into an inequality, solve the problem, and understand the answer. Take a look!
How Do You Use Division with Positive Numbers to Solve an Inequality Word Problem?

This tutorial provides a great real world application of math. See how to turn a word problem into an inequality. Then solve the inequality by performing the order of operations in reverse. Don't forget that if you multiply or divide by a negative number, you MUST flip the sign of the inequality! That's one of the big differences between solving equalities and solving inequalities.
How Do You Use Division with Negative Numbers to Solve an Inequality Word Problem?

This tutorial provides a great real world application of math. See how to turn a word problem into an inequality. Then solve the inequality by performing the order of operations in reverse. Don't forget that if you multiply or divide by a negative number, you MUST flip the sign of the inequality! That's one of the big differences between solving equalities and solving inequalities.
How Do You Use Multiplication with Positive Numbers to Solve an Inequality Word Problem?

This tutorial provides a great real world application of math. See how to turn a word problem into an inequality. Then solve the inequality by performing the order of operations in reverse. Don't forget that if you multiply or divide by a negative number, you MUST flip the sign of the inequality! That's one of the big differences between solving equalities and solving inequalities.
How Do You Use Multiplication with Negative Numbers to Solve an Inequality Word Problem?

This tutorial provides a great real world application of math. See how to turn a word problem into an inequality. Then solve the inequality by performing the order of operations in reverse. Don't forget that if you multiply or divide by a negative number, you MUST flip the sign of the inequality! That's one of the big differences between solving equalities and solving inequalities.
How Do You Solve a Word Problem Using an Inequality Where You're Multiplying Positive Fractions?

This tutorial provides a great real world application of math. See how to turn a word problem into an inequality. Then solve the inequality by performing the order of operations in reverse. Don't forget that if you multiply or divide by a negative number, you MUST flip the sign of the inequality! That's one of the big differences between solving equalities and solving inequalities.
How Do You Solve a Word Problem Using an Inequality Where You're Multiplying Negative Fractions?

This tutorial provides a great real world application of math. See how to turn a word problem into an inequality. Then solve the inequality by performing the order of operations in reverse. Don't forget that if you multiply or divide by a negative number, you MUST flip the sign of the inequality! That's one of the big differences between solving equalities and solving inequalities.
How Do You Solve a Word Problem Using a Multi-Step Inequality?

This tutorial provides a great real world application of math. See how to turn a word problem into an inequality. Then solve the inequality by performing the order of operations in reverse. Don't forget that if you multiply or divide by a negative number, you MUST flip the sign of the inequality! That's one of the big differences between solving equalities and solving inequalities.
How Do You Solve a Word Problem Using an Inequality With Variables on Both Sides?

This tutorial provides a great real world application of math. See how to turn a word problem into an inequality. Then solve the inequality by performing the order of operations in reverse. Don't forget that if you multiply or divide by a negative number, you MUST flip the sign of the inequality! That's one of the big differences between solving equalities and solving inequalities.
Why Can't You Divide By 0?

Why can't you divide by 0? This may be one of the most asked math questions. Get this question answered once and for all by watching this tutorial!
How Do You Determine Whether a Triangle Can Be Formed Given Three Side Lengths?

If you're given 3 side measurements, there's a quick way to determine if those three sides can form a triangle. Follow along with this tutorial and learn what relationship these sides need in order to form a triangle.
How Do You Solve a Problem by Making an Organized List?

Organization is a big part of math. In this tutorial, you'll see how organizing information given in a word problem can help you solve the problem and find the answer!
How Do You Figure Out a Tip?

If you need to leave a tip at a restaurant, you can quickly estimate the amount in your head! This tutorial shows you how to use estimation and mental math to estimate a tip!
How Do You Find Equivalent Ratios by Making a Table?

To master equivalent ratios, you need to practice. Follow along with this tutorial to practice filling in a table with equivalent ratios.
How Do You Estimate a Sale Price?

Sales are great, but how much are you really saving? This tutorial shows you how to estimate the sales price of an item.
How Do You Figure Out a Percent From a Part to Part Ratio?

Word problems and percents can be a fun combination! This tutorial shows you how to find the percent of something in a basket using ratios!
How Do You Figure Out a Percent From a Part to Whole Ratio?

If you want to find a percent in a word problem, you may be able to use a ratio to help you! This tutorial shows you how to do exactly that!
How Do You Add Integers Using a Number Line?

Combining integers? You could use a number line to help find the answer! In this tutorial, see how to use a number line to add together integers with the same sign and ones with opposite signs. Take a look!
How Do You Subtract Integers Using a Number Line?

Subtracting integers? You could use a number line to help find the answer! In this tutorial, see how to use a number line to subtract integers with the same sign and ones with opposite signs. Take a look!
What are the Rules for Using Absolute Values to Add Integers?

When you're combining numbers, there are some helpful rules to make that process a little easier. This tutorial shows you the rules for using absolute values to combine integers with the same sign or with opposite signs. Take a look!
What is the Opposite, or Additive Inverse, of a Number?

Have you ever combined two numbers together and found their sum to be zero? When that happens, those numbers are called additive inverses of each other! In this tutorial, you'll learn the definition for additive inverse and see examples of how to find the additive inverse of a given value.
How Do You Solve a Two-Step Equation?

Becoming a pro at solving equations takes practice! Follow along with this tutorial to see an example of solving an equation for a variable.
How Do You Factor a Greatest Common Factor Out of an Expression?

The greatest common factor (GCF) is the largest factor two or more numbers have in common. Finding the GCF can be very useful in simplifying an expression or solving an equation. In this tutorial, see how to identify the GCF of an expression and factor it out. Check it out!
How Do You Divide a Decimal by a Decimal?

Dividing decimals? Then this tutorial is a must see! Follow along and learn how you can divide decimals by rewriting the problem as a fraction and then using long division to solve. Check it out!
How Do You Divide Fractions?

Dividing fractions? Change that division to a multiplication by multiplying the dividend by the reciprocal of the divisor. Learn all about it by watching this tutorial!
How Do You Turn a Fraction Into a Terminating Decimal?

Did you know that a fraction just represents a division? To turn a fraction into a decimal, divide the numerator by the denominator. In this tutorial, see how to convert a fraction into the terminating decimal it represents.
How Do You Turn a Fraction Into a Repeating Decimal?

Did you know that a fraction just represents a division? To turn a fraction into a decimal, divide the numerator by the denominator. In this tutorial, see how to convert a fraction into the repeating decimal it represents.
What is a Terminating Decimal?

A terminating decimal is a decimal that ends. It's a decimal with a finite number of digits. Did you know that all terminating decimals can be rewritten as fractions? Watch this tutorial to learn about terminating decimals and see some examples!
What is a Repeating Decimal?

A repeating decimal is a decimal that has a digit, or a block of digits, that repeat over and over and over again without ever ending. Did you know that all repeating decimals can be rewritten as fractions? To make these kinds of decimals easier to write, there's a special notation you can use! Learn about repeating decimals in this tutorial.
How Do You Find Equivalent Ratios?

Ratios are used to compare numbers. When you're working with ratios, it's sometimes easier to work with an equivalent ratio. Equivalent ratios have different numbers but represent the same relationship. In this tutorial, you'll see how to find equivalent ratios by first writing the given ratio as a fraction. Take a look!
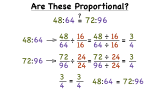
How Do You Know If Two Ratios are Proportional?

Ratios are proportional if they represent the same relationship. One way to see if two ratios are proportional is to write them as fractions and then reduce them. If the reduced fractions are the same, your ratios are proportional. To see this process in action, check out this tutorial!
How Do You Use Unit Rates to Compare Rates?

Word problems allow you to see the real world uses of math! This tutorial shows you how to use ratios to figure out which store has a better deal on cupcakes. Take a look!
How Do You Determine if Two Ratios are Proportional Using Cross Products?

Trying to figure out if two ratios are proportional? If they're in fraction form, set them equal to each other to test if they are proportional. Cross multiply and simplify. If you get a true statement, then the ratios are proportional! This tutorial gives you a great example!
How Do You Solve a Proportion by Finding an Equivalent Ratio?

Trying to find a missing value in order to create a proportion with two ratios? Take the ratios in fraction form and identify their relationship. Use that relationship to find your missing value. This tutorial will show you how!
How Do You Determine Whether Values in a Table are Proportional?

To see if multiple ratios are proportional, you could write them as fractions, reduce them, and compare them. If the reduced fractions are all the same, then you have proportional ratios. To see this process step-by-step, check out this tutorial!
How Do You Solve a Proportion Using the Multiplication Property of Equality?

Trying to find a missing value in a ratio to create proportional ratios? You could use the multiplication property of equality! In this tutorial, see how to use this property to find a missing value in a ratio. Take a look!
How Do You Solve a Scale Model Problem Using a Scale Factor?

This tutorial provides a great real world application of math! You'll see how to use the scale on a house blueprint to find the scale factor. Then, see how to use the scale factor and a measurement from the blueprint to find the measurement on the actual house! Check out this tutorial and see the usefulness blueprints and scale factor!
How Do You Find the Scale of a Model?

Want some practice with scale? Then check out this tutorial and you'll see how to find the scale of a model given the lengths of the model and the actual object. Take a look!
How Do You Use the Scale on a Map to Find an Actual Distance?

Maps help us get from one place to another. In this tutorial, you'll learn how to use a map to find an actual distance.
How Do You Figure Out What Size a Model Should Be if You Have a Scale?

Before tall sky scrapers are build, a scale model of the building is made, but how does the architect know what size the model should be? Follow along with this tutorial to find out!
How Do You Solve a Word Problem Using Ratios?

This tutorial shows you how to use a ratio to create equivalent ratios. Then, use a multiplier to find a missing value and solve the word problem. Take a look!
What are Equivalent Ratios?

Equivalent ratios are just like equivalent fractions. If two ratios have the same value, then they are equivalent, even though they may look very different! In this tutorial, take a look at equivalent ratios and learn how to tell if you have equivalent ratios.
What is a Scale?

In math, the term scale is used to represent the relationship between a measurement on a model and the corresponding measurement on the actual object. Without scales, maps and blueprints would be pretty useless. Check out this tutorial and learn about scale factor!
How Do You Figure Out the Price of a Marked Up Item?

The price of items is always changing. You've probably went to the store to buy an item and found that its price has been marked up. In this tutorial, learn how to figure out the new price of an item that was marked up. Take a look!
How Do You Figure Out Whether a Percent of Change is an Increase or a Decrease?

Word problems are a great way to see the real world applications of math! In this tutorial, you'll see how the percent of change can be found from the information given in a word problem. Check it out!
How Do You Use Complementary Angles to Find a Missing Angle?

If two angles are complementary, that means that they add up to 90 degrees. This is very useful knowledge if you have a figure with complementary angles and you know the measurement of one of those angles. In this tutorial, see how to use what you know about complementary angles to find a missing angle measurement!
How Do You Use Supplementary Angles to Find a Missing Angle?

If angles combine to form a straight angle, then those angles are called supplementary. In this tutorial, you'll see how to use your knowledge of supplementary angles to set up an equation and solve for a missing angle measurement. Take a look!
How Do You Find Angle Measures for Adjacent Angles?

Learning how to find missing angle measurements is a very useful skill. In this tutorial, get some practice finding missing angle measurements by first creating an equation. Take a look!
What are Complementary Angles?

Do complementary angles always have something nice to say? Maybe. One thing complementary angles always do is add up to 90 degrees. In this tutorial, learn about complementary angles and see how to use this knowledge to solve a problem involving these special types of angles!
What are Supplementary Angles?

Knowing about supplementary angles can be very useful in solving for missing angle measurements. This tutorial introduces you to supplementary angles and shows you how to use them to solve for a missing angle measurement. Take a look!
What are Vertical Angles?

Vertical angles have a very special quality. They are always congruent to one another! Check out this tutorial to learn about and see how to identify vertical angles!
How Do You Find the Width of a Rectangle if You Know its Length and Perimeter?

Trying to figure out a missing side length of a rectangle? Got the perimeter and the other side length? Then you can use that information and the formula for the perimeter of a rectangle to find that missing length! This tutorial will show you how!
How Do You Find the Area of a Rectangle?

To find the area of a rectangle, multiply the length times the width! This tutorial will show you how to find the area of a rectangle. Check it out!
How Do You Find the Area of a Triangle?

Finding the area of a triangle? Know the length of the base and the height? Then just take those values and plug them into the formula for the area of a triangle and solve! This tutorial shows you how.
How Do You Find the Area of a Parallelogram?

Looking for the area of a parallelogram? Got the length of the base and the height? Then plug those values into the formula for the area of a parallelogram and solve. This tutorial takes you through the process!
How Do You Find the Area of a Trapezoid?

Want to find the area of a trapezoid? If you have the length of each base and the height, you can use them to find the area. In this tutorial, you'll see how to identify those values and plug them into the formula for the area of a trapezoid. Then see how to simplify to get your answer!
How Do You Find the Area of a Composite Figure?

Composite figures are just a combination of simpler figures in disguise! In this tutorial, you'll see how to break down a composite figure into simpler figures. Then, see how to find the area of each of those individual figures to find the area of the entire composite figure. Watch the whole process in this tutorial!
How Do You Find the Circumference of a Circle if You Know the Diameter?

Trying to find the circumference of a circle? Know the diameter? Then you can use the formula for the circumference of a circle to get the answer! Just plug the value for the diameter into the formula and solve. This tutorial shows you how!
How Do You Find the Circumference of a Circle if You Know the Radius?

Trying to find the circumference of a circle? Know the radius? Then you can use the formula for the circumference of a circle to get the answer! Just plug the value for the radius into the formula and solve. This tutorial shows you how!
How Do You Find the Radius of a Circle if You Know the Circumference?

Want to find the radius of a circle? Already have the circumference? Then you can use the formula for the circumference of a circle to solve! This tutorial shows you how to use that formula and the given value for the circumference to find the radius. Take a look!
How Do You Find the Area of a Circle if You Know the Radius?

If you know the radius of a circle, you can use it to find the area of that circle. Just plug that value into the formula for the area of a circle and solve. Watch this tutorial to see how it's done!
How Do You Find the Radius of a Circle if You Know the Area?

Want to find the radius of a circle? Already have the area? Then you can use the formula for the area of a circle to solve! This tutorial shows you how to use that formula and the given value for the area to find the radius. Take a look!
How Do You Find the Area of a Circle if You Know the Diameter?

If you have the diameter of a circle, you can use it to find the area of that circle. Just plug that value into the formula for the area of a circle and solve. Watch this tutorial to see how it's done!
How Do You Find the Volume of a Rectangular Prism?

Finding the volume of a rectangular prism isn't so bad, especially if you already know the length, width, and height. In this tutorial, you'll see how to use that information and the formula for the volume of a rectangular prism to get the answer. Check it out!
How Do You Find the Volume of a Triangular Prism?

Finding the volume of a triangular prism isn't so bad, especially if you already know the length and height of the base and the height of the prism. In this tutorial, you'll see how to use that information and the formula for the volume of a triangular prism to get the answer. Take a look!
How Do You Find the Volume of a Composite Figure?

Composite figures are just a combination of simpler figures in disguise! In this tutorial, you'll see how to break down a composite figure into simpler figures. Then, see how to find the volume of each of those individual figures to find the volume of the entire composite figure. Watch the whole process in this tutorial!
How Do You Find the Lateral and Surface Areas of a Rectangular Prism?

The lateral area of a three-dimensional solid is the area of all the lateral faces. In this tutorial, you'll see how to use the dimensions of a rectangular prism to find the lateral area. Take a look!
How Do You Find the Lateral and Surface Areas of a Triangular Prism?

Want to know how the find the lateral and surface areas of a triangular prism? Then check out this tutorial! You'll see how to apply each formula to the given information to find the lateral area and surface area. Take a look!
What is a Circle?

Circles are a fundamental part of math! In this tutorial, you'll be introduced to circles and see the different parts of a circle such as the diameter, radius, and chord. Check out this tutorial to learn about circles!
What is a Composite Figure?

Ever notice that some figures look like a combination of multiple other figures? These types of figures are called composite figures. This tutorial introduces you to composite figures and shows you how to break up a composite figure into multiple shapes. Take a look!
What is the Formula for the Area of a Rectangle?

Trying to find the area of a rectangle? There's a formula that can help! Check out this tutorial to learn about the formula for the area of a rectangle.
What is the Formula for the Area of a Triangle?

Did you know that the formula for the area of a triangle can be found by using the formula for the area of a parallelogram? In this tutorial, you'll see how it's done! Take a look!
What is the Formula for the Area of a Parallelogram?

Parallelograms and rectangles are pretty similar. In fact, you can turn a parallelogram into a rectangle to find the formula for the area of a parallelogram! Check out this tutorial to see how it's done!
What is the Formula for the Area of a Trapezoid?

Trying to figure out the formula for the area of a trapezoid? You could start by creating a parallelogram out of two trapezoids. Then, use the formula for the area of a parallelogram to figure out the formula for the area of one trapezoid. This tutorial shows you how!
What is Circumference?

The circumference of a circle is the distance around that circle. But what is the formula to find the circumference? In this tutorial, you'll learn the formulas for the circumference of a circle. Take a look!
What is the Formula for the Area of a Circle?

Did you know that you can figure out the formula for the area of a circle by first turning the circle into a parallelogram? It seems a little weird, but it really works! Watch this tutorial to see how it's done!
What is the Formula for the Volume of a Prism?

Trying to find the volume of a prism? Did you know that there's a formula to find that volume? In this tutorial, you'll learn about the formula for the volume of a prism. Check it out!
What is the Formula for the Surface Area of a Prism?

To find the lateral and surface areas of a prism, itâ€™s important to know their formulas. In this tutorial, youâ€™ll learn about each of these formulas and see them used in an example. Check it out!
How Do You Use a Sample to Make Predictions About a Population?
How Do You Determine All the Possible Outcomes of an Experiment?

When you perform an experiment, how do you figure out all the possible outcomes? Follow along with this tutorial to see!
What is an Unbiased Sample?

When you're trying to learn about a population, it can be helpful to look at an unbiased sample. An unbiased sample can be an accurate representation of the entire population and can help you draw conclusions about the population. This tutorial introduces you to unbiased sampling!