Proof and congruence. The student uses the process skills with deductive reasoning to prove and apply theorems by using a variety of methods such as coordinate, transformational, and axiomatic and formats such as two-column, paragraph, and flow chart.

More Specific Topics in Proof and congruence. The student uses the process skills with deductive reasoning to prove and apply theorems by using a variety of methods such as coordinate, transformational, and axiomatic and formats such as two-column, paragraph, and flow chart.

Popular Tutorials in Proof and congruence. The student uses the process skills with deductive reasoning to prove and apply theorems by using a variety of methods such as coordinate, transformational, and axiomatic and formats such as two-column, paragraph, and flow chart.

How Do You Find the Distance Between Two Points?

Trying to find the distance between two points? Use the distance formula! Want to see how it's done? Check out this tutorial!
What are the Legs and Hypotenuse of a Right Triangle?

Looking for some terminology used with right triangles? Then this tutorial was made for you! In this tutorial, you'll be introduced to the names for the different parts of a right triangle. Check it out!
What is the Converse of the Pythagorean Theorem?

The converse of the Pythagorean Theorem is like the the Pythagorean Theorem in reverse. You can use it both forward and backward! Not all theorems work this way, but the Pythagorean Theorem does! This tutorial will show you how to use both the Pythagorean Theorem and its converse.
What is the Pythagorean Theorem?

The Pythagorean theorem is a very popular theorem that shows a special relationship between the sides of a right triangle. In this tutorial, you'll get introduced to the Pythagorean theorem and see how it's used to solve for a missing length on a right triangle!
What is the Distributive Property?

The distributive property is a very deep math principle that helps make math work. It's the rule that lets you expand parentheses, and so it's really critical to understand if you want to get good at simplifying expressions. So check out the tutorial and let us know what you think!
What is a Theorem?

Theorems are a way for mathematicians to make a general mathematical statement. These statements can be used to help you solve all sorts of problems! See an introductions to theorems with this tutorial.
How Do You Use the Law of Detachment to Draw a Valid Conclusion?

See the Law of Detachment in action! This tutorial shows you an example that uses the Law of Detachment to make a conclusion.
What is the Law of Syllogism?

If you have related conditional statements, the Law of Syllogism can help you link those conditional statements into one conditional statement. In this tutorial, you'll see how to combine related conditional statements using the Law of Syllogism.
How Do You Use Parallel and Perpendicular Theorems to Prove a Relationship Between Two Lines?

Parallel and perpendicular lines can be found in all sorts of places! In this tutorial, see how to use your knowledge of parallel and perpendicular lines to solve a word problem.
What is the Corresponding Angles Postulate?

When a transversal intersects parallel lines, the corresponding angles created have a special relationship. The corresponding angles postulate looks at that relationship! Follow along with this tutorial to learn about this postulate.
What is the Converse of the Corresponding Angles Postulate?

The corresponding angles postulate states that when a transversal intersects parallel lines, the corresponding angles are congruent. What if you go the other way and start with corresponding angles that are congruent? Is the converse of this postulate true? This tutorial explores exactly that!
How Do You Use a Congruence Postulate to Prove Triangles are Congruent?

When proving two triangles are congruent, you use information and postulates you already know to create a logical trail from what you know to what you want to show. This tutorial shows an example of using a congruence postulate to show two triangles are congruent!
How Do You Show that Corresponding Parts of Congruent Triangles are Congruent?

If you're given information about two triangles and asked to prove parts of the triangles are congruent, see if you can show the two triangles are congruent. If they are, then you know that the corresponding parts are congruent! Follow along with this tutorial to see an example.
How Do You Prove that Two Overlapping Triangles are Congruent?

Proofs are an important part of geometry. This tutorial shows you how to use given information to prove that two overlapping triangles are congruent!
How Do You Identify Common Parts in Overlapping Triangles?

It might seem like a challenge to make sense of figures with overlapping triangles, but it's not so difficult! This video gives some commonsense advice for identifying common angles and common sides in these figures.
What is the Angle-Side-Angle Postulate for Triangle Congruence?

What is the Angle-Side-Angle postulate? This postulate is just one of many postulates you can use to prove two triangles are congruent! This tutorial explains the ASA postulate.
What is CPCTC?

The term CPCTC can come up a lot when you're dealing with congruent triangles, but what does it mean? This tutorial gives a great explanation and shows you how to use it in an example!
What is the Hypotenuse-Leg Congruence Theorem?

There's a special theorem that helps you quickly figure out if two right triangles are congruent. This tutorial introduces you to that theorem and shows you how to use it!
How Can You Tell if a Point is on the Perpendicular Bisector of a Line Segment?

If you want to determine if a point is on the perpendicular bisector of a line segment, the Perpendicular Bisector Theorem and its converse might come in handy. This tutorial gives a great example of how to tell if a given point is a perpendicular bisector of a segment!
How Do You Use the Centroid to Find Segment Lengths in a Triangle?

When you're given the centroid of a triangle and a few measurements of that triangle, you can use that information to find missing measurements in the triangle! This tutorial shows you how it's done.
How Do You Determine Whether a Triangle Can Be Formed Given Three Side Lengths?

If you're given 3 side measurements, there's a quick way to determine if those three sides can form a triangle. Follow along with this tutorial and learn what relationship these sides need in order to form a triangle.
How Do You Use the Hinge Theorem to Compare Side Lengths in Two Triangles?

The Hinge Theorem helps you compare side measurements of two triangles when you have two sets of congruent sides. Follow along with this tutorial to see this theorem used to find the relationship between the sides of two triangles.
How Do You Write an Indirect Proof?

This tutorial looks at using an indirect proof to show that an equilateral triangle cannot have a right angle.
How Do You Put the Sides of a Triangle in Order According to Size if You Know Two Angles of the Triangle?

Trying to figure out which side of a trianlge is the shortest? How about the longest? All you need to get your answer are the angle measurements of the triangle! How does it work? Watch this tutorial to find out!
What is the Circumcenter of a Triangle?

This tutorial explains the ins and outs of the circumcenter of a triangle. You'll see how to build up from the Perpendicular Bisector Theorem to find the circumcenter of a triangle.
What is the Incenter of a Triangle?

The incenter of a triangle deals with the angle bisectors of a triangle. This tutorial shows you how to find the incenter of a triangle by first finding the angle bisectors.
What is the Hinge Theorem?

What's the Hinge Theorem all about? Watch this tutorial to find out!
What is the Triangle Midsegment Theorem?

The triangle midsegment theorem looks at the relationship between a midsegment of a triangle and the triangle's third side. Follow along with this tutorial to learn about the triangle midsegment theorem.
What is a Median of a Triangle?

A median of a triangle is a special line segment that connects two pieces of a triangle. This tutorial introduces you to the median of a triangle and shows you how many medians each triangle has!
How Do You Find Values for Variables in the Angles of a Quadrilateral To Make it a Parallelogram?

When you're given a quadrilateral with some of the interior angles defined with variables, you can find what values those variables need to have to make that quadrilateral a parallelogram. Follow along with this tutorial to learn what steps to take to get the answer!
How Do You Use the Diagonals of a Rectangle to Find the Value of a Variable?

When you make diagonals inside a rectangle, those diagonals are congruent. With this information, you can find the value of a variable that's part of a measurement of a diagonal. This tutorial shows you the steps.
How Do You Write a Coordinate Proof?

In this tutorial, you'll see how to write a coordinate proof to prove that two triangles are congruent. Check it out!
How Do You Find the Value for a Variable in the Angles of a Quadrilateral To Make it a Rhombus?

A parallelogram needs to have certain qualities in order to be a rhombus. In this tutorial, you'll use your knowledge of these shapes in order to find the value for a variable that will make a given parallelogram a rhombus.
How Do You Graph a Glide Reflection?

When you graph a composition of two transformations, you have to be very careful to perform all the steps in the right order! Watch this tutorial to see how to graph a glide reflection.
How Do You Identify a Congruence Transformation?

Want to figure out whether two figures are congruent? There's a mathematically precise way to do this! Watch this tutorial on congruence transformations to learn more.
What is a Congruence Transformation, or Isometry?

Not all transformations are created equal! Congruence transformations, or isometries, have a special property that distinguishes them from other transformations. This tutorial will show you what makes them special!
How Do You Determine Whether Two Chords are Equidistant From the Center of a Circle?

When you want to know if two chords are the same distance away from the center of the circle, there's a quick way to get the answer. In this tutorial, you'll learn how to find that answer and figure out which chords are equidistant from the center.
How Do You Use Intersecting Chords to Find Arc Measures in a Circle?

This tutorial shows you how to use your knowledge of intersecting arcs in a circle to find a missing arc measurement. Take a look!
How Do You Find the Measure of an Angle Created by Intersecting Chords in a Circle?

This tutorial shows you how to use your knowledge of intersecting chords in a circle to find a missing angle measurement.
How Do You Determine Whether a Line is Tangent to a Circle?

In this tutorial, you'll see how the Converse of the Pythagorean Theorem is used to figure out if a line is tangent to a circle.
What is a Tangent Line to a Circle?

What does it mean when a line is tangent to a circle? In this tutorial, you'll learn what a line needs to do to be a tangent line to a circle.
What is the Substitution Property of Equality?

If you ever plug a value in for a variable into an expression or equation, you're using the Substitution Property of Equality. This property allows you to substitute quantities for each other into an expression as long as those quantities are equal. Watch this tutorial to learn about this useful property!
How Do You Find Missing Angles in an Isosceles Triangle?

Trying to find a missing interior angle measurement in a triangle? See if you're working with a special type of triangle such as an equilateral or isosceles triangle. If you are, that knowledge can help you. In this tutorial, see how identifying your triangle first can be very helpful in solving for that missing measurement. Take a look!
How Do You Find Missing Angles in a Triangle With Variables?

Looking for the measurements of the interior angles of a given triangle? The Triangle Sum theorem might help. This theorem states that the interior angles of a triangle ALWAYS add up to 180 degrees! This tutorial shows you how to use that information to find those interior angle measurements.
How Do You Identify Corresponding Parts in Congruent Triangles?

When you have two congruent figures, that means that corresponding sides and corresponding angles are congruent. Get some practice identifying corresponding sides and angles by following along with this tutorial!
How Do You Find Missing Angles in an Isosceles Triangle Using Right Triangles?

Trying to find a missing interior angle measurement in a triangle? See if you're working with a special type of triangle such as an equilateral or isosceles triangle. If you are, that knowledge can help you. In this tutorial, see how identifying your triangle first can be very helpful in solving for that missing measurement. Take a look!
How Do You Find Missing Angles in a Transversal Diagram?

Got a diagram of a transversal intersecting parallel lines? Trying to figure out all the angle measurements? Take a look at this tutorial, and you'll see how find all the missing angle measurements by identifying vertical, corresponding, adjacent, and alternate exterior angles!
How Do You Find a Value for x that Makes Two Lines Parallel?

Got a transversal intersecting two lines? Trying to figure out if those lines are parallel? You could test to see if corresponding angles are congruent. This tutorial shows you how!
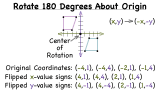
How Do You Rotate a Figure 180 Degrees Around the Origin?

Rotating a figure about the origin can be a little tricky, but this tutorial can help! This tutorial shows you how to rotate coordinates from the original figure about the origin. Then, simply connect the points to create the new figure. See this process in action by watching this tutorial!
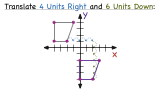
How Do You Use a Graph to Translate a Figure Diagonally?

Performing multiple translations on the graph of a figure is easier than you might think! In this tutorial, see how to use the graph of the original figure to perform each translation in order to get the graph of the new figure. Check it out!
What is a Rectangle?

A rectangle is one of the many fundamental shapes you'll see in math. Rectangles have special properties that can be very useful in helping you solve a problem. This tutorial introduces you to rectangles and explains their interesting qualities!
What is a Parallelogram?

A parallelogram is a special type of quadrilateral with some special properties. In this tutorial, take a look at parallelograms and learn what kinds of quadrilaterals can also be called parallelograms!
What is an Angle?

Angles are a fundamental building block for creating all sorts of shapes! In this tutorial, learn about how an angle is formed, how to name an angle, and how an angle is measured. Take a look!
What are Acute, Obtuse, Right, and Straight Angles?

Did you know that there are different kinds of angles? Knowing how to identify these angles is an important part of solving many problems involving angles. Check out this tutorial and learn about the different kinds of angles!
What are Supplementary Angles?

Knowing about supplementary angles can be very useful in solving for missing angle measurements. This tutorial introduces you to supplementary angles and shows you how to use them to solve for a missing angle measurement. Take a look!
What are Vertical Angles?

Vertical angles have a very special quality. They are always congruent to one another! Check out this tutorial to learn about and see how to identify vertical angles!
What are Parallel Lines?

Lines that are parallel have a very special quality. Without this quality, these lines are not parallel. In this tutorial, take a look at parallel lines and see how they are different from any other kind of lines!
What are Skew Lines?

Ever heard of skew lines? They're pretty cool! Take a look at this tutorial and you'll be introduced to skew lines.
What are Equilateral, Isosceles, and Scalene Triangles?

Did you know that there are different kinds of triangles? Knowing how to identify these triangles is an important part of solving many problems involving these triangles. Check out this tutorial and learn about some of the different kinds of triangles!
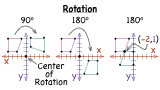
What is a Rotation?

Ever turned a door handle? You were performing a rotation! In math, rotations are just the same! Check out this tutorial to learn about rotations.
What is a Translation?

Ever slide something across a table? If so, then you have performed a translation! In this tutorial, learn the definition of translation and see some really neat examples. Take a look!
How Do You Find the Sum of the Interior Angles of a Polygon?

Polygons have all kinds of neat properties! For example, if you know the number of sides of a polygon, you can figure out the sum of the interior angles. That knowledge can be very useful when you're solving for a missing interior angle measurement. Check out this tutorial to learn how to find the sum of the interior angles of a polygon!
What is the Triangle Sum Theorem?

When you're dealing with triangles, the Triangle Sum theorem can be very useful in finding interior angle measurements. In this tutorial, learn how to find this helpful theorem!
What is a 45Â°-45Â°-90Â° Triangle?

A 45-45-90 triangle is a special right triangle with some very special characteristics. If you have a 45-45-90 triangle, you can find a missing side length without using the Pythagorean theorem! Check out this tutorial to learn about 45-45-90 triangles!
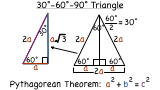
What is a 30-60-90 Degree Triangle?

A 30-60-90 triangle is a special right triangle with some very special characteristics. If you have a 30-60-90 degree triangle, you can find a missing side length without using the Pythagorean theorem! Check out this tutorial to learn about 30-60-90 triangles!