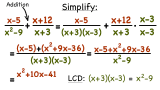Rational Functions and Equations
More Specific Topics in Rational Functions and Equations
Popular Tutorials in Rational Functions and Equations
-
How Do You Solve a Rational Equation by Adding Fractions?
Want some extra practice solving rational equations? This tutorial gives you just that! You'll see how to solve a rational equation containing rational expressions with common denominators. Then, you'll see how to solve an equation containing rational expressions with unlike denominators. Take a look!
-
How Do You Solve a Rational Equation by LCD Multiplication?
Want some extra practice solving rational equations? This tutorial gives you just that! You'll see how to solve a rational equation containing rational expressions with common denominators. Then, you'll see how to solve an equation containing rational expressions with unlike denominators. Take a look!
-
How Do You Divide Two Polynomials by Factoring and Canceling?
Simplifying a rational expression? You could factor the numerator and denominator and then cancel like factors. Learn what to do in this tutorial!
-
How Do You Identify Transformations in the Equation of a Rational Function?
If you can figure out how the equation of a rational function was transformed, then you can make a good sketch of that function without finding lots of individual points. Take a look at the video to learn more!
-
What is the Parent Function for a Rational Function?
Like linear, quadratic, and cubic functions, rational functions also have a parent. It's also the parent function for the reciprocal function family. Check out this tutorial to learn more about its characteristics!
-
What's the Inverse Variation or Indirect Proportionality Formula?
Ever heard of two things being inversely proportional? Well, a good example is speed and time. The bigger your speed, the less time it takes to get to where you are going. So when one variable is big, the other is small, and that's the idea of inverse proportionality. But you can express inverse proportionality using equations, and that's an important thing to do in algebra. See how to do that in the tutorial!
-
How Do You Use the Formula for Inverse Variation to Write an Equation?
If two things are inversely proportional, you can bet that you'll need to use the formula for inverse variation to solve! In this tutorial, you'll see how to use the formula for inverse variation to find the constant of inverse variation and then solve for your answer.
-
How Do You Add Two Rational Expressions with Different Denominators?
Adding rational expressions together? Don't have common denominators? No problem! Find the least common denominator (LCD) and change each rational expression into an equivalent expression with that LCD. Once you have common denominators, you're ready to add and simplify! Watch it all in this tutorial!
-
How Do You Find the Least Common Denominator of Two Rational Expressions?
When adding or subtracting rational expressions, you need have common denominators just like any other fraction. If you don't have common denominators, then you'll need to find the least common denominator (LCD) and use it to get those denominators to be the same. Learn how to find the LCD of two rational expressions by watching this tutorial!
-
How Do You Multiply Two Rational Expressions?
Multiplying together two rational expression isn't so hard, especially if you know the proper steps! This tutorial will take you through all the steps necessary to multiply together two rational expressions and then simplify the product to get the answer. Check it out!
-
How Do You Graph a Rational Function by Finding its Asymptotes and Zeros?
In a rational function, the denominator of the rational expression holds the key to the location of the vertical asymptotes. The relationship between degrees of the numerator and denominator determine the nature of the horizontal asymptotes. Check out the tutorial to learn more!
-
How Do You Use the Equation for a Rational Function to Find Its Horizontal Asymptotes?
Rational functions behave predictably as they head off to infinity. Follow this tutorial to learn more about their asymptotic behavior!