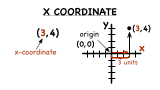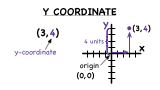Background Tutorials

• Use a pair of perpendicular number lines, called axes, to define a coordinate system, with the intersection of the lines (the origin) arranged to coincide with the 0 on each line and a given point in the plane located by using an ordered pair of numbers, called its coordinates. Understand that the first number indicates how far to travel from the origin in the direction of one axis, and the second number indicates how far to travel in the direction of the second axis, with the convention that the names of the two axes and the coordinates correspond (e.g., x-axis and x-coordinate, y-axis and y-coordinate).

  • • 

    What is the X-Coordinate?

    Ordered pairs are a crucial part of graphing, but you need to know how to identify the coordinates in an ordered pair if you're going to plot it on a coordinate plane. In this tutorial, you'll see how to identify the x-coordinate in an ordered pair!

  • • 

    What is the Y-Coordinate?

    Ordered pairs are a crucial part of graphing, but you need to know how to identify the coordinates in an ordered pair if you're going to plot it on a coordinate plane. In this tutorial, you'll see how to identify the y-coordinate in an ordered pair!

• Use similar triangles to explain why the slope m is the same between any two distinct points on a non-vertical line in the coordinate plane; derive the equation y = mx for a line through the origin and the equation y = mx + b for a line intercepting the vertical axis at b.

  • • 

    What Does the Slope of a Line Mean?

    You can't learn about linear equations without learning about slope. The slope of a line is the steepness of the line. There are many ways to think about slope. Slope is the rise over the run, the change in 'y' over the change in 'x', or the gradient of a line. Check out this tutorial to learn about slope!