www.VirtualNerd.com

What is the Formula for the Volume of a Pyramid?

What is the formula for the volume of a pyramid?

Summary

  1. The triangle has a base length of 4 inches and height of 2 inches
  2. The triangular bases of this pyramid and prism have the same measurements
  3. Both the prism and the pyramid are 10 inches high
  4. V is volume, the red V is for the pyramid
  5. B is the area of the base
  6. h is the height of the prism or pyramid
  7. B=(1/2)bh1 which is 1/2 times the base (b) and height (h1) of the triangular base
  8. h2 is the height of the solid

Notes

    1. Prisms and pyramids are both solids with polygon bases
    2. For our example, the polygon bases are triangles
    3. A polygon is a closed figure with three or more sides that are line segments
    4. The triangles each have a base length of 4 inches and height of 2 inches
    5. The prism and the pyramid are both 10 inches high
    6. The height of a pyramid is a line perpendicular to the base and extending to the peak
    1. The prism and pyramid must have the same base measurements and the same height for this to be true
    1. V stands for volume
    2. The volume of a pyramid is one-third the volume of a prism with the same measurements
    1. A is the area of the triangle
    2. Since our base is a triangle, B, the area of the base, is the same as A
    3. b is the base of the triangle
    4. h is the height of the triangle
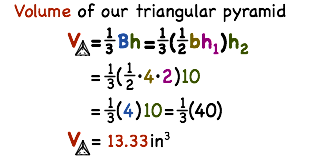
    1. V stands for volume
    2. B is the area of the base and h is the height of the pyramid
    3. B=(1/2)bh1 which is (1/2)(base of the triangle)(height of the triangle)
    4. We'll call the height of the pyramid 'h2' so we don't confuse it with the height of the triangle
    5. b=4 in, h1=2 in, and h2=10 in
    6. (1/3)(40) = 13.333... so let's round to 2 decimal places
    7. Volume is in 'inches cubed'
    1. V stands for volume
    2. B is the area of the base and h is the height of the prism
    3. B=(1/2)bh1 which is (1/2)(base of the triangle)(height of the triangle)
    4. We'll call the height of the prism 'h2' so we don't confuse it with the height of the triangle
    5. b=4 inches and h1=2 inches
    6. h2=10 inches
    7. B=(1/2)(4)(2)=4 square inches
    8. Volume is in 'inches cubed'
    1. Let's prove that the volume we calculated for the pyramid is indeed one-third the volume of its related prism
    2. 40 is the volume of the prism
    3. ÷3 is dividing by three which is the same as multiplying by 1/3
    4. 13.333... is a repeating decimal which we can also say as '13.3 repeating'
    5. ≈ means approximately
    6. 13.3 repeating rounded to two decimal places is 13.33
    7. 13.33 is the volume of the pyramid