
www.VirtualNerd.com
How Do You Find the Lateral and Surface Areas of a Triangular Prism?
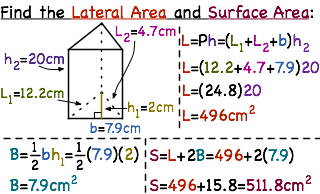
Find the lateral and surface areas of the triangular prism
Summary
- h1 is the height of the triangular base, h2 is the height of the prism
- L1 and L2 are the lengths of the legs of the triangular base
- 'b' is the length of the base of the triangular base
- The lateral area, 'L', is the perimeter of the base, 'P', times the height of the prism
- 'B' stands for the area of the triangular base
- The surface area, 'S', is the lateral area plus the area of the bases
Notes
-

- The lateral area is the sum of all the faces that are not bases
-

- We can find 'P', the perimeter of the base, by adding together the three sides of the triangle
- So we add 12.2+4.7+7.9 to get P=24.8
- Then we multiply by the height of the prism, 20 cm, to find 'L', the lateral area
- Multiplying 24.8 by 20 gives us the lateral area: L=496 cm2
-

- Both bases are triangles
-

- Both bases are triangles
- Congruent triangles have the same area
- Remember, the formula for the area of a triangle is (1/2)bh, where 'b' is the base and 'h' is the height
- In our diagram, the base of the triangular base is represented by 'h1
-

- Surface area is equal to the lateral area plus the area of the bases
-

- L, the lateral area, we found to be 496
- We also found that B, the area of one of the bases, is 7.9
- Since the bases are congruent, we can just multiply 7.9 by 2 and then add 496, the lateral area
- This will give us the surface area of the prism