
www.VirtualNerd.com
How Do You Find the Area of a Sector of a Circle?
Find the area of the shaded sector in the circle at the right. Use 3.14 for π . Round your answer to the nearest hundredths.
Summary
- Remember. to find the area of a sector, you need to know the measure of the central angle and the radius, both of which we know!
- 'A' is the area of the sector (the shaded region)
- The degree measure of the central angle, 'N', is 135
- Pi, '
π ', is an irrational number, so we its approximation of 3.14 - The radius, 'r', is 9 yards
- We plug our values in and use the order of operations
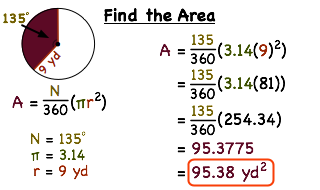
Notes
-

- Remember. to find the area of a sector, you need to know the measure of the central angle and the radius, both of which we know!
-

-
Our formula is "A=N/360(
π r2)" - 'A' is the area of the sector (the shaded region)
- The degree measure of the central angle, 'N', is 135
-
Pi, '
π ', is an irrational number, so we its approximation of 3.14 - The radius, 'r', is 9 yards
-
Our formula is "A=N/360(
-

-
Our formula is "A=N/360(
π r2)" - The degree measure of the central angle, 'N', is 135
-
Pi, '
π ', is an irrational number, so we its approximation of 3.14 - The radius, 'r', is 9 yards
- After plugging in, we get "A=135/360(3.14(9)2)"
-
Our formula is "A=N/360(
-

- Use the order of operations to solve this problem
- First, square the 9
- 92=81
- Then simplify by multiplying what's in the parentheses
- 3.14(81)=254.34
- 135/360(254.34)=95.3775
- Since we need to round to the nearest hundredths, or two decimal places, the area of the sector is 95.38 yards squared