
www.VirtualNerd.com
How Do You Write and Use a Prediction Equation?
Draw a line of fit for the data given and write its equation in slope-intercept form. Then use the equation to predict the number of staph infections at a hospital 16 months after the initial outbreak.
Summary
- We have a scatter plot of 6 points
- The points (6,14) and (14,10) allow us to find the slope, -0.5
- We can use a point and the slope to find the point-slope form of the line
- Then we can convert it to slope-intercept form
- Our equation gave us the point (16,9), which means that after 16 months there will be 9 staph infections
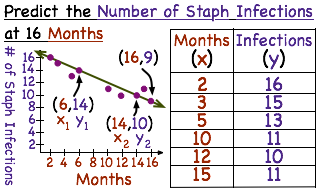
Notes
-

- A 'line of fit' is a line that fits closely to the set of data on a scatter plot
-

- We can use these two points with the slope formula to find the slope of the line
-

-

- 'm' stands for slope
-
∆ y means the change in y-coordinates and∆ x means the change in x-coordinates - We've plugged (6,14) in for (x1,y1) and (14,10) in for (x2,y2)
-

- Point slope form is y-y1=m(x-x1)
-

- Point slope form is y-y1=m(x-x1)
- We found 'm' in step 3 - so we can plug -0.5 in for 'm'
- We labeled one of our points (x1, y1) in step 2, so we can plug 6 in for x1 and 14 in for y1
-

- You'll have to distribute the -0.5 first
- Then you'll have to add 14 to both sides
-

- Distributing the -0.5, we get -0.5x-[(-0.5)•6]
- -[(-0.5)•6]=+3
- Then we can add 14 to both sides to solve for y
- y=-0.5x+17 is the slope-intercept form of the line
-

- Since we have a slope-intercept form of this line, we can estimate the number of staph infections by plugging 16 months into the equation
-

- The number of months is represented by 'x', so we can plug 16 in for x to find the number of staph infections
- -0.5•16=-8
- -8+17=9
- Our equation gave us the point (16,9), which means that after 16 months there will be 9 staph infections