How Do You Find a Scale Factor in Similar Figures?

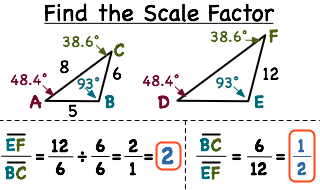
Triangles ABC and DEF are similar figures
Similar figures have corresponding angles that are equal and corresponding sides that are proportional
The 'o' stands for degrees
Sides EF and BC are corresponding sides, so we can use them to set up a ratio
The bars above EF and BC mean that they are line segments
When we reduce the ratio, we get a scale factor of 2
We could also flip our ratio over to get a scale factor of 1/2

1. Since triangles ABC and DEF are similar figures, their corresponding sides are proportional
2. That means that you can multiply each side of one triangle by the same number to get the sides of the other triangle
3. That number is the scale factor, which is what we're trying to find
1. To find the scale factor, we need to find the ratio between the corresponding sides of the triangles
2. When we reduce this ratio, it will give us a number that describes the relationship between the sides
3. This number will be our scale factor
1. EF and BC are corresponding sides, because they fall between the same angles in the two triangles
2. We have values for both EF and BC, so we can use those values to set up a ratio
3. EF is 12 units and BC is 6 units
4. This gives us a ratio of 12/6
1. To simplify, we need to divide the numerator and denominator by the greatest common factor of the two numbers
2. The greatest common factor of 12 and 6 is 6
3. When we divide 12 by 6 we get 2
4. When we divide 6 by 6 we get 1
5. This gives us a reduced ratio of 2/1, or 2
6. So our scale factor is 2!
1. If we multiply each side of triangle ABC by 2, we will get triangle DEF
2. This means that 2 is the scale factor
3. We could have started by putting BC over EF when we made our ratio
4. Then we would have gotten a scale factor of 1/2
5. This scale factor just tells us how to get from triangle DEF to ABC instead
6. If we multiply each side of triangle DEF by 1/2, we will get triangle ABC

Given two similar figures, find the scale factor.