
www.VirtualNerd.com
How Do You Find Missing Measurements of Similar Figures Using a Scale Factor?
Use a scale factor to find the missing measurement, 'x'.
Summary
- Here, 'x' is the length of the longest side of the DEF triangle
- Triangle ABC is similar to triangle DEF because all angles are the same
- EF and BC are corresponding sides and can be compared using a ratio
- The bar over EF and BC means they are line segments
- The length of EF is 12, and the length of BC is 6
- The greatest common factor of 12 and 6 is 6, so divide both numbers by 6 to get 2/1, or 2
- A scale factor of 2 means the sides of triangle DEF are twice those of triangle ABC
- We can flip our initial ratio and get the same answer!
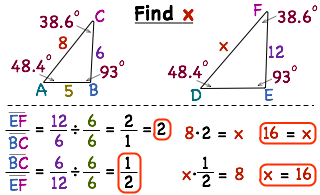
Notes
-

- Here, 'x' is the same as saying 'side DF' of triangle DEF
-

- A ratio of corresponding sides will help us find the scale factor between the two triangles
- The given figures show us that EF=12 and BC=6
- The sides correspond because they sit between angles with the same measurement
-

- Up to this point, our ratio was 12/6
- The greatest common factor of 12 and 6 is 6, so divide them both by 6 to get 2/1, or 2
- A scale factor of 2 means triangle DEF is twice as big as triangle ABC
- So the sides of triangle DEF are twice as long as those of triangle ABC
-

- A scale factor of 2 means triangle DEF is twice as big as triangle ABC
- So the sides of triangle DEF are twice as long as those of triangle ABC
- That means we can just multiply 8 by 2 to find x
-

-
Our equation '8
• 2 = x' simplifies to '16 = x' - So 'x', or side DF, has a length of 16!
-
Our equation '8
-

-
BC/EF = 6/12
÷ 6/6 = 1/2 - A scale factor of 1/2 means triangle ABC is half as big as triangle DEF
- So the sides of triangle ABC are half as long as those of triangle DEF
- So our equation becomes:
-
'x
• (1/2) = 8' - Multiplying both sides of this equation by 2 will cancel 1/2 on the left and we get 'x=16'!
-
BC/EF = 6/12