
www.VirtualNerd.com
How Do You Turn a Repeating Decimal Into a Fraction?
Write the repeating decimal 0.4444444... as a fraction.
Summary
- The bar above the 4 means that it's a repeating decimal
- Let the variable 'x' stand for the repeating decimal 0.4444...
- Then we can form an equation, x=0.44444....
- 4 is the only repeating digit in our repeating decimal, which means we multiply both sides of our equation by 10
- Remember, x=0.44444..., so we can put x instead of 0.44444...
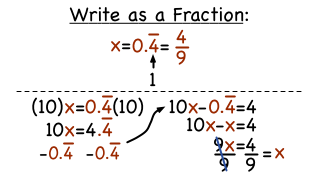
Notes
-

-
- Let the variable 'x' stand for the repeating decimal 0.44444...
- Then we can form an equation, x=0.44444....
-

- The number of repeating digits tells us how many zeros to put behind the 1
- 4 is the only repeating digit in our repeating decimal, which means we multiply both sides of our equation by 10
- If there were 2 repeating digits, like 0.424242..., we'd multiply by 100
- If there were 3 repeating digits,0.425425..., we'd multiply by 1000
-

- The Multiplication Property of Equality tells us that if we multiply one side of an equation by a number, we must also multiply the other side by that number
- This allows us to multiply by numbers while still preserving the equality!
-

- The number of repeating digits tells us how many zeros to put behind the 1
- If there were 2 repeating digits, like 0.424242..., we'd multiply by 100
- If there were 3 repeating digits,0.425425..., we'd multiply by 1000
-

- The Subtraction Property of Equality says that if subtract a number from one side of an equation, we have to subtract it from the other
- The bar above the 4 means that it's the repeating decimal 0.44444...
-

- The bar above the 4 means that it's the repeating decimal 0.44444...
- We're substituting x for 0.44444...
-

- Let the variable 'x' stand for the repeating decimal 0.4444...
-

- If any two things are equal to the same third thing, then they are all equal to each other!
- The variable 'x' stands for the repeating decimal 0.4444...