What Does Divisibility Mean?

Work out the long division of 165÷5 and see that you end up with the whole number 33 and no remainder
The definition of divisibility states that a whole number 'p' is divisible by another whole number 'q' if you get a whole number when you divide 'p' by 'q'
So in our first example, 165 is divisible by 5 since we had no remainder or decimals in our final answer of 33
Work out the long division of 542 ÷6

1. If we work out the long division of 165÷5, we find that we end up with the whole number 33, with no remainder
1. Here, 'p' and 'q' are variables representing whole numbers
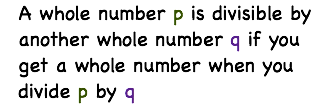
1. This is the definition of divisibility!
2. Here, 'p' and 'q' are variables representing whole numbers
3. So in our first example, 165 is divisible by 5 since we had no remainder or decimals in our final answer of 33
1. This is also part of the definition of divisibility!
2. Here, 'p' and 'q' are variables representing whole numbers
3. So in our first example, since 165 is divisible by 5, 5 is a factor of 165 and so is the answer 33
1. If we try to divide 542 by 6, we get a decimal in our answer
2. Since 90.33... is not a whole number, 542 is not divisible by 6 and 6 is not a factor of 542

Definition: Divisibility