
www.VirtualNerd.com
How do you find the distance travelled given constant speed and time?
A radio message is sent to the Mars Rover. The message moves at a constant speed of 3x108 m/s, and travels for 1 minute and 45 seconds. How far did the message travel a) in meters? b) in miles?
Summary
- E- Earth
- MR- Mars Rover
- d- distance, an unknown
- t- time of travel is 1 minute and 45 seconds
- s- speed, in this case the speed of light in meters per second
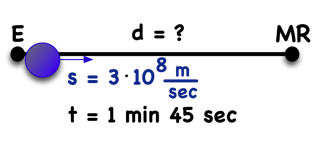
Notes
-

- Constant speed means that you don't get faster, and you don't get slower
- Think d=s•t when you see constant speed
-

- d- distance
- s- speed
- t- time
- Think of this with constant speed problems
-

- t- time
- min- minutes
- sec- seconds
- We need the total time in seconds, since that is the unit given in the speed
-

- t- time
- sec- seconds
- We need the total time in seconds, since that is the unit given in the speed
-

- d- distance
- m- meters
- sec- seconds
- Plug numbers into the equation!
-

- d- distance
-

- Convert meters to miles
- m- meters
- cm- centimeters
- in- inches
- ft- feet
- mi- miles
-

- d- distance
- Here we've converted meters to miles
-
- m- meters
- sec- seconds
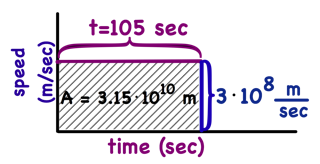
- Constant speed on a speed vs. time graph mean a flat line
- Height of a constant speed line on the graph is speed
- Length of the constant speed line is the time
- Area of the rectangle gives total distance
-

- The area under a speed vs. time graph tells you the distance traveled
-

- For a rectangle, just take the base and multiply by the height, and you get the area
-

- m- meters
- sec- seconds
- Plug the numbers in to find the area of the rectangle, and therefore the distance
-

- d- distance
- Using graphs alone we found the same answer as what we got with algebra