
www.VirtualNerd.com
How Do You Graph the Natural Base Exponential Function?
Graph f(x) = ex by making a table.
Summary
- f(x) = ex is the natural base exponential function
- 'e' is the natural base
- '
≈ ' means 'approximately equal to' - Plug each 'x' value into ex
- You can either use the 'e' button on your calculator or use the approximation 2.718 for 'e' to find each value
- f(x) = ex has a horizontal asymptote along the x-axis
- An asymptote is an imaginary line that a graph approaches but never reaches
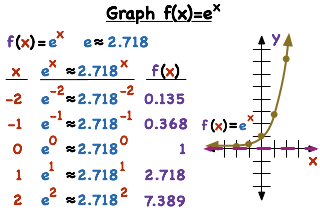
Notes
-

- We can choose some values for 'x' to plug into f(x) = ex to find points to graph
- Remember, our variable here is 'x', NOT 'e'
- 'e' is the natural base and is approximately equal to 2.718 -- it is NOT a variable
-

- Remember, our function is the natural base exponential function f(x) = ex
- So we need to choose some values for 'x' that we can plug into ex to get values for f(x)
-

- If you press the ex button on your calculator and then enter each value for 'x', it will approximate those values for you
- Remember, we know that 'e' is approximately equal to 2.718
- So you could also use 2.718 in place of 'e' to get an approximation
-

- Now that we have values for x and f(x), we can plot points in order to graph our function
-

- Remember, f(x) is the same thing as y
- So we can turn each pair of x and f(x) values into an ordered pair and plot them in the coordinate plane
- Plot the points (-2,0.135), (-1,0.368), (0,1), (1,2.718), and (2,7.389)
- Connecting the points gives us the graph of our exponential function
-

- Notice how the graph looks like it gets really close to the x-axis, but never actually touches it
- The x-axis is where y = 0
-

- An asymptote is an imaginary line that a graph approaches but never reaches
- As our x-values get more and more negative, our f(x) values will get closer and closer to 0, but will never actually reach 0