www.VirtualNerd.com

How Do You Convert From Exponential Form to Natural Logarithmic Form?

Write ex = 9 in natural logarithmic form.

Summary

  1. 'e' is called the 'natural base' and is approximately equal to 2.71828
  2. You can change between exponential form and logarithmic form
  3. 'b' stands for the base
  4. 'x' represents the exponent
  5. 'log' is short for 'logarithm'
  6. '≈' means 'approximately equal to'
  7. 'ln' stands for natural log
  8. logex is usually written as 'ln(x)'
  9. ln(9) = x is ex = 9 in natural logarithmic form

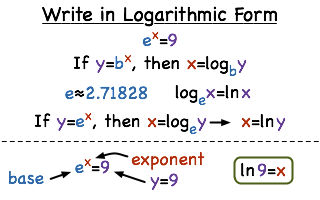
Notes

    1. A logarithm is the opposite, or inverse, of an exponential expression
    1. The exponential expression y = bx is equivalent to the logarithmic expression x = logby
    2. logby = x is asking, 'What number 'x' do we need to raise 'b' to in order to get 'y'?'
    1. A natural logarithm is just a logarithm whose base is the natural base 'e'
    2. 'e' is an irrational number approximately equal to 2.71828
    1. If y = ex, then x = logey
    2. 'e' is NOT a variable -- it's always equal to the same irrational number, which we can approximate to 2.71828
    3. 'e' is also known as the 'natural base'
    4. logey is usually written as 'ln(x)'
    1. The base of a logarithm is always the same as the base of its corresponding exponential expression
    1. The base of an exponential function is always the number that's being raised to a power
    2. Here we're raising 'e' to the 'x' power, so 'e' is our base
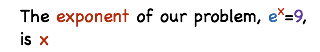
    1. In exponential form, the exponent is always the power that some number is being raised to
    2. Here we're raising 'e' to the 'x' power, so the 'x' is our exponent
    1. 'y' is what the exponential expression is equal to
    1. We usually write 'ln' instead of 'loge
    1. 9, the number the exponential expression is equal to, goes inside the logarithm
    2. We end up with 'ln(9) = x'