
www.VirtualNerd.com
How Do You Convert From Logarithmic Form to Exponential Form?
Write log2 8 = 3 in exponential form.
Summary
- 'log' stands for 'logarithm'
- A logarithm is the inverse of an exponential
- You can go back and forth between y = bx and x = logby
- 'b' stands for the base
- 'x' is the exponent
- For log28 = 3, the base is 2
- The number that the log is equal to, 3, will be the exponent
- The number we're taking the log of, 8, is what the exponent will be equal to
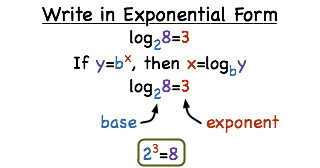
Notes
-

-

- 'log' stands for 'logarithm'
- A logarithm is the inverse of an exponential
- y = bx is in exponential form
- This definition means that you can go back and forth between y = bx and x = logby
-

- The base in logarithmic form will be the same base in exponential form
-

- The base is the 'b' in both exponential and logarithmic forms
-

- The base is the 'b' in both exponential and logarithmic forms
- Logarithmic form is logby = x
- In this case, b = 2
-

-
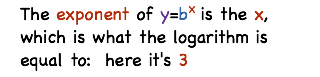
- For log28 = 3, the base is 2 and the exponent will be 3
- 'log' stands for 'logarithm'
-

- Exponential form is y = bx
- 'b' stands for the base
- 'x' is the exponent
-

- Remember the definition of a logarithm:
- If y = bx, then x = logby
- 'b' stands for the base
- 'x' is the exponent
- Starting with log28 = 3, we end up with 23 = 8 in exponential form
- 23 = 2•2•2, which does equal 8!
- That means we've done this problem correctly