
www.VirtualNerd.com
What is Quadratic Form of a Polynomial Equation?
What is Quadratic Form?
Summary
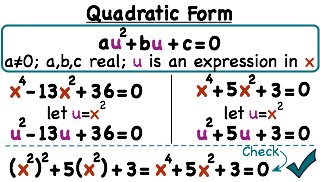
- x4-13x3+36 is a 4th degree polynomial
- 'u' is an expression in 'x' that we can substitute into a higher degree polynomial to make it look like a quadratic
- u2-13u+36 is x4-13x2+36 rewritten in quadratic form
- You can solve equations in quadratic form just like regular quadratic equations
- u2=(x2)2 which equals x4
- 'a', 'b', and 'c' must all be real numbers, and 'a' cannot equal 0
- 'u' doesn't always have to equal 'x2'
- For example, to put '4x6-6x3+5' in quadratic form, you would let 'u=2x3'
Notes
-

- x4-13x3+36 is a 4th degree polynomial
- We know how to solve quadratic equations, so let's find a way to rewrite this to look like a quadratic
-

- Quadratics are 2nd degree polynomials, which means their highest power term is an x2 term
- We can rewrite the term x4 to look sort of like a 2nd degree term:
- x4 = x(2•2) = (x2)2
- Substituting 'u' for 'x2' makes the 4th and 2nd degree terms look like 2nd and 1st degree terms
- It looks like a quadratic equation with three terms that's easy to solve!
-

- A quadratic is a 2nd degree polynomial
- au2+bu+c is called the quadratic form of the equivalent expression in 'x'
- 'u' is an expression in terms of 'x' that we can substitute for 'x' to make the polynomial look like a quadratic
-

- A quadratic is a 2nd degree polynomial
- 'a', 'b', and 'c' are constant coefficients of each term
- If a=0, then we wouldn't have a quadratic because 0•u2=0, and we need a squared term to have a quadratic
- An 'expression in x' means that a substitute variable, like 'u', represents an algebraic expression with variable 'x' in it
-

- A quadratic is a 2nd degree polynomial
- To be quadratic in form, you MUST be able to find an expression in x that will make the polynomial appear to be a quadratic
- An 'expression in x' means that a substitute variable, like 'u', represents an algebraic expression with variable 'x' in it
- x3-x2+6 is an example of a polynomial that CANNOT be written in quadratic form
-

- x4+5x2+3 is a 4th degree polynomial
- Substituting 'u' for 'x2' puts this polynomial in quadratic form
- 'u' just needs to be an expression with 'x' in it that will make the polynomial look like a quadratic when we substitute it in
- For example, to get the quadratic form of '4x6-6x3+5', let 'u=2x3'
-

- Remember, when you're solving a quadratic equation you're solving for 'x'
- So after you find the answer in 'u', you'll have to substitute the 'x' expression back in for 'u'
- Then you can finish solving for 'x'
-

- This may help you remember what to do
- To go to a Halloween party, you need to change into a costume
- At the party you appear to be a ghost
- 'u' disguises an expression in 'x' just like a costume masks who you are
- But you have to remove the disguise to get back to reality