www.VirtualNerd.com

What is a Quadratic Inequality?

What is a quadratic inequality?

Summary

  1. A quadratic inequality looks like a quadratic equation, but with a <, >, ≤, or ≥ instead of a =
  2. '>' means 'greater than' and '<' means 'less than'
  3. '≤' means 'less than or equal to' and '≥' means 'greater than or equal to'
  4. The highest degree term in a quadratic inequality will always have a degree of 2
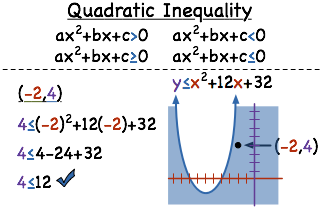
  5. y≤x2+12x+32 is a quadratic inequality we're graphing
  6. Getting a true statement by plugging (-2,4) into the quadratic inequality means that (-2,4) is part of the solution set
  7. 4≤12 is a true statement, so (-2,4) is definitely in the solution set

Notes

    1. A quadratic equation has the form ax2+bx+c=0
    2. So a quadratic inequality would look a bit like that, but with a <, >, ≤, or ≥ instead of a =
    3. '<' stands for 'less than'
    4. '>' stands for 'greater than'
    5. '≤' stands for 'less than or equal to'
    6. '≥' stands for 'greater than or equal to'
    1. The highest degree term needs to be 2 for a quadratic
    2. If there is a term with a higher degree, or if there is no x2 term, it is NOT a quadratic inequality!
    3. A quadratic equation takes the form ax2+bx+c=0
    4. So a quadratic inequality would look a bit like that, but with a <, >, ≤, or ≥ instead of a =
    5. '<' stands for 'less than'
    6. '>' stands for 'greater than'
    7. '≤' stands for 'less than or equal to'
    8. '≥' stands for 'greater than or equal to'
    1. To be a quadratic inequality, the highest term needs to be an x2 term, and it needs to have an inequality symbol!
    2. '<' stands for 'less than'
    3. '≥' stands for 'greater than or equal to'
    4. '≤' stands for 'less than or equal to'
    5. '>' stands for 'greater than'
    6. If it doesn't look like an inequality follows the standard form, try moving all the terms to one side
    1. To be a quadratic inequality, the highest term needs to be an x2 term, and it needs to have an inequality symbol!
    2. x3<0 is not a quadratic inequality because one side has a degree of 3
    3. 8x2-5=0 is a quadratic equation because of the '='
    4. x4-6x2≤0 is not a quadratic inequality because there's a 4th degree term on the left side
    5. 4x>7 has no x2 term, which means it's a linear inequality not a quadratic
    1. Graphing a quadratic inequality is like graphing a linear inequality, except instead of a line there will be a parabola
    2. You can also represent the quadratic inequality as a quadratic function
    3. Then you can graph that function, and shade the included region
    1. Any ordered pair in the shaded region will give you a true statement if you plug it into the quadratic function y≤x2+12x+32
    2. '≤' stands for 'less than or equal to'
    1. Plug (-2,4) into the inequality y≤x2+12x+32
    2. '≤' stands for 'less than or equal to'
    3. This gives us 4≤(-2)2+12•(-2)+32
    4. When we simplify on the right we get 4-24+32=12
    5. 4 is less than or equal to 12 is a true statement!
    6. We can see that (-2,4) also lies in the shaded region of our graph, which means that it is definitely a solution!