
www.VirtualNerd.com
How Do You Convert a Quadratic Equation from Intercept Form to Standard Form?
Write y = 2(x + 3)(x - 1) in standard form.
Summary
- y=2(x+3)(x-1) is in intercept form
- y=a(x-p)(x-q) is the general form for intercept form
- In intercept form, 'p' and 'q' are the x-intercepts
- So in our equation, the x-intercepts are -3 and 1
- y=ax2+bx+c is the general form for standard form
- 'a', 'b', 'c', 'p', and 'q' are constants
- Multiply the binomials together using FOIL
- Distribute the 2 into the parentheses
- y=2x2+4x-6 is standard form of the equation y=2(x+3)(x-1)
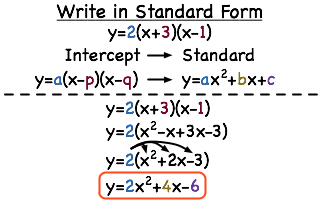
Notes
-

-

- y=a(x-p)(x-q) is the general form for intercept form
- In intercept form, 'p' and 'q' are the x-intercepts
- Right now our equation, y=2(x+3)(x-1), is in intercept form
- We want to get put it into standard form, which is the form y=ax2+bx+c
- 'a', 'b', 'c', 'p', and 'q' are all constants
- Notice that 'a' is the same in both standard and intercept form
-

- FOIL together the two binomials on the right, (x+3)(x-1)
-

- Make sure to keep the 2 out front as you FOIL!
- Multiply the First terms together, x•x=x2
- Multiply the Outer terms together, x•(-1)=-x
- Multiply the Inner terms together, 3•x=3x
- Multiply the Last terms together, 3•(-1)=-3
-

- Distribute in the 2 in front of x2+2x-3
-

- Distribute in the 2 in front of x2+2x-3
- To distribute the 2, multiply it by each term of x2+2x-3
-

- Notice that a = 2 in both standard form and intercept form!