
www.VirtualNerd.com
How Do You Write a Quadratic Equation in Vertex Form if You Have the Vertex and Another Point?
Write an equation in vertex form for the parabola that has a vertex of (-6,-4) and passes through the point (-2,12).
Summary
- Vertex form of a quadratic equation is y=a(x-h)2+k, where (h,k) is the vertex of the parabola
- The vertex of a parabola is the point at the top or bottom of the parabola
- 'h' is -6, the first coordinate in the vertex
- 'k' is -4, the second coordinate in the vertex
- 'x' is -2, the first coordinate in the other point
- 'y' is 12, the second coordinate in the other point
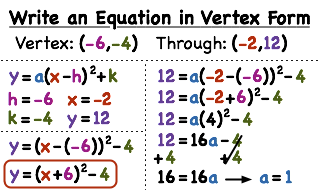
Notes
-

- Vertex form of a quadratic equation is y=a(x-h)2+k, where (h,k) is the vertex of the parabola
-

- Vertex form is useful, because it lets us pick out the vertex of a parabola really quickly just by looking at the equation!
- Notice how there is a minus sign in front of 'h' in vertex form
- So we'll need to be careful when we pick out 'h', because we'll have to switch the sign that's in front of it in the equation
-

- Once we figure out what we've been given, we'll be able to tell what we still need to find
-

- The vertex of a parabola is the point at the top or bottom of the parabola
- Remember, in vertex form the vertex is the point (h,k)
- So the first coordinate in the vertex, -6, will be 'h' in our equation
- The second coordinate in the vertex, -4, will be 'k' in our equation
-

- We know that our parabola also goes through the point (-2,12)
- Remember, ordered pairs are of the form (x,y)
- Since we know (-2,12) is on our graph, we can use those values for 'x' and 'y' to help us find our equation
- The first coordinate in an ordered pair is the x-coordinate, so x will be -2
- The second coordinate in an ordered pair is the y-coordinate, so y will be 12
-

- We have everything we need to write our equation in vertex form except for 'a'
- But if we plug everything else into the vertex form equation, we'll be able to solve for 'a'
- Remember, vertex form is y=a(x-h)2+k
- We know that h = -6, k = -4, x = -2, and y = 12
- Since our value for 'k' is negative, we'll subtract it instead of adding it
-

- The only thing we're missing in our equation is 'a'
- So we can simplify and solve our equation to find 'a'
-

- First we need to simplify inside the parentheses by subtracting -6 from -2 to get 4
- Then we square 4 to get 16
- Add 4 to both sides to get the 'a' term by itself
- Then divide both sides by 16: the 16's cancel out on both sides, leaving us with a=1
-

- Now that we have values for 'a', 'h', and 'k', we can write a general equation for our parabola using vertex form
- Remember, vertex form is y=a(x-h)2+k
-

- Remember, vertex form is y=a(x-h)2+k
- To write a general equation for our parabola, we want to leave 'x' and 'y' as variables but have values for everything else
- We found that a=1, h=-6, and k=-4, so we can plug these values into the general form to get our equation
- So we get y=(x-(-6))2-4 when we plug in
- Simplifying inside the parentheses we get y=(x+6)2-4 for our final equation
-

- Remember, vertex form is y=a(x-h)2+k
- To write a general equation for our parabola, we want to leave 'x' and 'y' as variables but have values for everything else
- We found that a=1, h=-6, and k=-4, so we can plug these values into the general form to get our equation
- So we get y=(x-(-6))2-4 when we plug in
- Simplifying inside the parentheses we get y=(x+6)2-4 for our final equation