
www.VirtualNerd.com
How Do You Convert a Quadratic from Standard Form to Vertex Form by Completing the Square if a≠1?
Write y = 2x2 + 12x – 4 in vertex form by completing the square.
Summary
- Our equation is in standard form to begin with: y=ax2+bx+c
- We want to put it into vertex form: y=a(x-h)2+k
- We can convert to vertex form by completing the square on the right hand side
- Adding 18 to both sides gives us a perfect square trinomial on the right
- The vertex of a quadratic equation in vertex form is (h,k), so our vertex is (-3,-22)
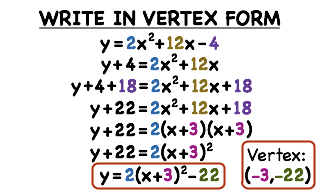
Notes
-

- Right now our quadratic equation, y=2x2+12x-4 is in standard form
- We want to get it into vertex form
- To do this, we are going to use the method of completing the square
-

- Our equation is y=2x2+12x-4
- So a = 2, b = 12, and c = -4 in our equation
- Vertex form allows us to easily pick out the vertex of a quadratic function
- Remember, the vertex is the point where a parabola crosses its axis of symmetry
-

- Usually when we are completing the square, we are trying to solve for 'x'
- Here we're not actually trying to find a value for 'x' - we're just using the same method to rearrange the equation
- Since vertex form also has an 'x' and a 'y', we need to keep that 'y' in our equation while we complete the square on the other side
-

- A perfect square trinomial is a trinomial that can be factored into something squared
- So when you factor it, you would have two identical binomials being multiplied together
-

- We want to be able to factor the right hand side into two identical binomials
- We can't do that if our last term is -4, so we need to move it over to the other side
- Then we can figure out what we DO need to add to get it to factor how we want it
-

- Make sure to add 4 on BOTH sides to preserve the equality!
- The 4's cancel out on the right, leaving us with just 2x2+12x
-

- In order to complete the square, we want the coefficient in front of x2 to be 1
- So we need to factor a 2 out of the right hand side first
- Then we can complete the square with what is left
- We can just ignore the 2 for now and focus on the x2+6x
- Don't forget about the 2 though - we'll come back to it later!
-

- A perfect square trinomial has the form ax2+bx+c, just like standard form
- So 'c' is the constant term we're adding at the end
- We want to find a value for 'c' that will allow us to factor the right hand side into two identical binomials
-

- A perfect square trinomial factors into the product of two identical binomials
- Since our x2 term doesn't have a coefficient in front of it, we know the first terms of our binomials will be 'x'
- Since the middle term of the trinomial is positive, the signs of our binomials will be positive as well
- When we multiply out (x+3) times (x+3), we will get 3x+3x for our middle terms, which adds up to 6x
- For our last term we would get 3•3, or 9, which is our value for 'c'
-

- 9 is the number that we need to add INSIDE the parentheses
- But we're still multiplying everything in the parentheses by 2
- So we can't just add 9 to both sides - we need to add 9 inside the parentheses then multiply the 2 back through
- Then we can find out what number we ACTUALLY need to add to the left hand side to keep the equation equal
-

- We want to add 9 inside the parentheses to create the perfect square trinomial we were trying to make
- When we distribute the 2, we can see that we are actually adding 18 to the right hand side
-

- When we multiplied out the right hand side, we saw that we were actually adding 18
- So to keep the equation equal, we need to add 18 to the left as well
-

- We already had the right hand side factored from before, so this should be pretty easy!
-

- Factoring out the 2 gives us the perfect square trinomial we had in the parentheses before
-

- Perfect square trinomials factor into the product of two identical binomials
- And we've done that already too!
- We used the factored form to figure out how to make the perfect square trinomial in the first place!
- Multiplying two identical things is the same as squaring that thing, so we can rewrite (x+3)•(x+3) as (x+3)2
-

- Our equation is ALMOST in vertex form - but we need to have 'y' by itself on one side
- In order to do that, we need to subtract 22 from both sides to get rid of it on the left
-

- Make sure to subtract 22 from BOTH sides to preserve the equality!
- The 22's cancel on the left, leaving 'y' by itself, which is what we wanted!
-

- Remember that vertex form of a quadratic equation is y=a(x-h)2+k
- Then the vertex is the point (h,k)
- Notice that in vertex form, the 'h' has a minus sign in front of it
- This means that when we pick out the value for 'h', we need to flip the sign that's in front of it
- So since the 3 has a plus sign in front of it, our value for 'h' is -3
- But 'k' has a plus sign in front of it in vertex form, so the sign stays the same and our value for 'k' is -22