www.VirtualNerd.com

How Do You Solve a Quadratic Inequality by Graphing?

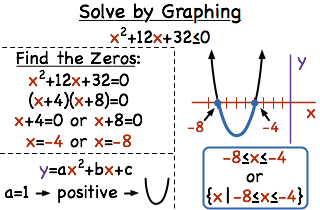
Solve x2 + 12x + 32 ≤ 0 by graphing.

Summary

  1. '≤' means 'less than or equal to'
  2. The zeros are where the graph crosses the x-axis, or where the function equals 0
  3. y=ax2+bx+c is the standard form of a quadratic
  4. Since coefficient in front of x2, 1, is positive, the parabola will open upwards
  5. The blue highlighted region is the part of the parabola that's less than or equal to 0
  6. The solution can be a compound inequality or in set notation
  7. The vertical bar in set notation means 'such that'

Notes

    1. The inequality is x2+12x+32≤0
    2. '≤' means 'less than or equal to'
    1. The inequality is x2+12x+32≤0
    2. Once we have the equation x2+12x+32=y, we can graph it to help us find the solutions
    3. The points where the y-values are equal to 0 are called the zeros of a function
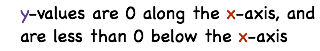
    1. Our inequality is asking where x2+12x+32, the graph of our parabola, is LESS THAN OR EQUAL TO 0
    2. So we're looking for those x-values make the graph dip below the x-axis
    1. The zeros of our parabola are where the graph crosses the x-axis
    2. These are also the values for 'x' that make the left side of the inequality equal 0
    3. The zeros of a quadratic inequality are also called critical values
    1. We'll solve x2+12x+32=0 for x
    2. This will give us the zeros of the function and let us know where the graph crosses the x-axis
    1. 'x' is the variable for the x-coordinate in x2+12x+32=0
    2. Our equation factors pretty easily, so we'll factor to solve for 'x'
    3. x2+12x+32=0 factors into (x+4)(x+8)=0
    1. Since we have two binomials multiplied together to equal 0, we can set each binomial equal to 0 to solve for 'x'
    2. So we have x+4=0 or x+8=0
    1. Remember, standard form of a quadratic is y=ax2+bx+c
    2. 'a' is the coefficient in front of x2
    3. The sign of 'a' will tell us whether our parabola opens up or down
    1. The equation is x2+12x+32=0
    2. Since there is no number in front of x2, that means the coefficient is 1
    1. The sketch of the graph doesn't need to be exact here
    2. We just need to see where it goes below the x-axis
    3. So as long as the graph is the right shape, we can figure out the answer
    1. Remember, we have zeros at x = -4 and x = -8
    1. Any point below the x-axis will have a negative y-value
    2. So the points on the parabola will have negative y-values below the x-axis
    3. This means that the graph will be less than 0 when it is below the x-axis, so those x-values will be part of the solution set
    4. The zeros, -4 and -8, are also part of the solution set, because they are where the graph equals 0
    1. Remember, the solution is the set of all x-values that make the graph less than or equal to 0
    1. You can write the solution as a compound inequality with two '≤' signs: -8≤x≤-4
    2. This means that 'x' needs to either be equal to -4 or -8 or between them
    3. You can also write the solution in set builder notation
    4. Like this: {x|-8≤x≤-4}
    5. The vertical bar in set notation means 'such that'
    6. '≤' means 'less than or equal to'