
www.VirtualNerd.com
How Do You Use The Discriminant to Determine the Number of Real or Complex Solutions to a Quadratic Equation?
Use the discriminant to determine if a quadratic equation has two real solutions, one real solution, or two complex solutions.
Summary
- Since there is no coefficient in front of x2, that means there is an invisible 1
- So 'a' for our equation is 1
- 'b', the coefficient in front of the 'x', is -2
- 'c', the constant term, is 3
- b2-4ac is the expression for the discriminant
- -8 is the value for our discriminant
- Since the discriminant is negative, our equation has two complex solutions
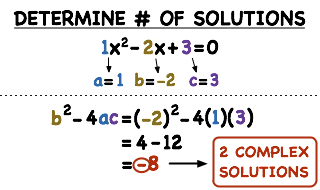
Notes
-

-

- The quadratic equation we're working with is x2-2x+3=0
- Remember, the discriminant tells us how many and what type of solutions a quadratic equation has
-

- The discriminant comes from the Quadratic Formula
- It tells us how many and what type of solutions a quadratic equation has
-

- Remember, the discriminant tells us how many and what type of solutions a quadratic equation has
- The symbol going over the b2-4ac is called a 'radical', or square root symbol
-
The '
± ' symbol means 'plus or minus' - The 'a', 'b', and 'c' values in the Quadratic Formula come from the general form of a quadratic: ax2+bx+c=0
- The discriminant is the expression under the radical in the Quadratic Formula: b2-4ac
-

- Remember, the discriminant is equal to b2-4ac
- The 'a', 'b', and 'c' values come from the general form of a quadratic equation: ax2+bx+c=0
- In the Quadratic Formula we have a plus and a minus in front of the radical
- This will give us two different answers, since we need to both add and subtract
- If we get 0 under the radical, we'd be adding and subtracting 0 since the square root of 0 is 0
- That would give us the same answer either way
-

- The standard form of a quadratic equation is ax2+bx+c=0
- Our quadratic equation is x2-2x+3=0
- Since there is no coefficient in front of x2, that means there is an invisible 1
- So 'a' for our equation is 1
- 'b', the coefficient in front of the 'x', is -2
- Remember to include the sign with the rest of the number!
- 'c', the constant term, is 3
-

- We need to plug our 'a', 'b', and 'c' values into the expression for the discriminant: b2-4ac
- For our equation a=1, b=-2, and c=3
- When we plug in for the discriminant we get (-2)2-4(1)(3)
- Squaring -2 gives us 4 and multiplying -4•1•3 gives us -12
- Taking 4-12 gives us -8, which is the value of our discriminant
-

-

- The discriminant is -8, so the number under the square root in the Quadratic Formula is negative
- The square root of a negative number gives us an imaginary number
- Since there is a plus or minus in front of the square root, we're both adding and subtracting an imaginary number
- So we will have two complex solutions to our equation