
www.VirtualNerd.com
How Do You Add Complex Numbers by Graphing in the Complex Plane?
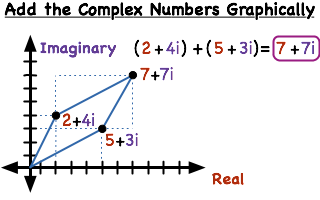
How do you add 2 + 4i and 5 + 3i graphically?
Summary
- 'i' is the imaginary unit and is equal to the square root of -1
- On the complex plane, the horizontal axis is the real axis
- The vertical axis is the imaginary axis
- Draw lines from each graphed point to the origin
- Starting at 5+3i, draw another line by moving right 2 and up 4
- Starting at 2+4i, draw another line by moving right 5 and up 3
- The point where the lines meet is the sum of the complex numbers, 7+7i
Notes
-

- The complex plane is the coordinate system we use to graph complex numbers
- Just like the coordinate plane, the complex plane has two axes
- But the horizontal axis is the real axis and the vertical axis is the imaginary axis
- So we graph the real part of the complex number on the real axis and the imaginary part on the imaginary axis
-

-

- 'i' is the imaginary unit and is equal to the square root of -1
- 2 is the real part of the complex number
- Since 2 is positive, we move 2 units to the right on the real (horizontal) axis
- 4i is the imaginary part of the complex number
- Since 4i is also positive, we move 4 units up on the imaginary (vertical) axis
-

- 'i' is the imaginary unit and is equal to the square root of -1
- 5 is the real part of the complex number
- Since 5 is positive, we move 5 units to the right on the real (horizontal) axis
- 3i is the imaginary part of the complex number
- Since 3i is also positive, we move 3 units up on the imaginary (vertical) axis
-

- Remember, the origin is the point (0,0)
- We'll use these lines later when we actually go to add the complex numbers
-

- Remember, a parallelogram has opposite sides that are parallel and congruent
- Congruent sides are the same length
-

- To create a parallelogram, we need to make two more sides that are parallel and congruent to the two we already have
- Parallel lines have the same slope, or rise over run
- Start at 5+3i, then use the rise over run from 2+4i to draw a new line parallel and congruent to the line we drew to the origin
- Then start at 2+4i, and use the rise over run from 5+3i to draw a new line parallel and congruent to the line we drew to the origin
-

- The new point we reached when we made our parallelogram is the sum of the two complex numbers
- Counting on the real axis, we've gone right 7 units
- That means the real part of the solution will be positive 7
- Counting on the imaginary axis, we've gone up 7 units
- That means the imaginary part of the solution will be positive 7i
- Put the two parts together, and our sum is 7+7i!