
www.VirtualNerd.com
How Do You Solve a Rational Equation?
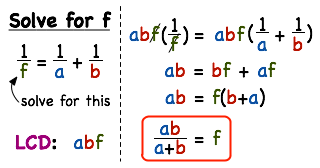
Solve the equation 1/f = 1/a + 1/b for f.
Summary
- Our contains three variables: 'f', 'a', and 'b'
- We want to solve for the 'f', which is in the denominator of the first fraction
- Since each denominator is unique, the LCD is the product of all three: 'abf'
- Multiplying each term by the LCD will help us eliminate any fractions in our equation
- abf(1/f) = ab(1) = ab
- abf(1/a) = bf(1) = bf
- abf(1/b) = af(1) = af
- We can factor an 'f' out of 'bf+af' to get: 'f(b+a)'
- Dividing by (a+b), or (b+a), will give us 'f' by itself on the right
- So we get: 'f = (ab)/(a+b)'
Notes
-

-

- Our equation is '1/f = 1/a + 1/b'
- 'f', 'a', and 'b' are all variables
-

- The variables in our equation are: 'f', 'a', and 'b'
- Our problem says to solve for 'f'
-

- Our problem says to solve for 'f'
- Our first fraction is '1/f', where 'f' is the variable we want to solve for
-

- Our problem says to solve for 'f', so we want to get it by itself
- Our equation is '1/f = 1/a + 1/b'
-

- Our equation is '1/f = 1/a + 1/b'
- 'f' is the variable we want to solve for
-

- Our first fraction is '1/f', where 'f' is the variable we want to solve for
- We need to figure out how to get 'f' out of the denominator and by itself
-

- The fractions in our equation are '1/f', '1/a', and '1/b'
- So we'll need to look at the denominators of each fraction
-

- The fractions in our equation are '1/f', '1/a', and '1/b'
- The denominators of each fraction are 'f', 'a', and 'b'
-

- The denominators of each fraction are 'f', 'a', and 'b'
- Since each denominator is unique, the LCD is the product of all three: 'abf'
-

- Since each denominator is unique, the LCD is the product of all three: 'abf'
-

- We determined the LCD to be 'abf'
- Multiplying 'abf' by each term will help us get 'f' by itself
-

- Left of the equal sign, we have abf(1/f) = ab(1) = ab
-

- Right of the equal sign, we have abf(1/a + 1/b) = abf(1/a) + abf(1/b) = bf(1) + af(1) = bf + af
-

- Our equation now looks like: 'ab = bf + af'
-

- Up to this point, our equation looked like: 'ab = bf + af'
- On the right side, both terms contain 'f' so we factor an 'f' out to get: 'f(b+a)'
-

- Up to this point, our equation looked like: 'ab = f(b + a)'
- Recognize that dividing by '(a+b)' is the same as dividing by '(b+a)
- Remember, if we divide one side by '(a+b)', we must divide the other side by the same
-

- The division by '(a+b)' gave us 'f' by itself!
- Remember, 'f' is the variable we were trying to solve for