
www.VirtualNerd.com
How Do You Solve a Word Problem with a Rational Equation?
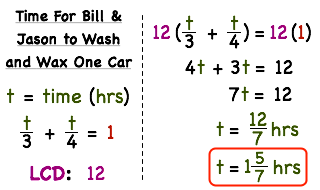
The local high school is holding a car wash to raise funds for varsity sports. Bill can wash and wax one car in 3 hours. Jason can wash and wax one car in 4 hours. If Bill and Jason work together, how long will it take them to wash and wax one car?
Summary
- If time is 't', then 't/3' is the number of cars Bill can wash and wax in 't' hours, and 't/4' is the number of cars Jason can wash and wax in 't' hours
- Since we're combining their efforts to finish 1 car, we add 't/3' and 't/4', setting them equal to 1
- 't/3+t/4=1' is a rational equation
- The smallest number that the denominators '3' and '4' are both factors of is '12'
- Multiplying by the LCD will help us to get 't' by itself
- The time it takes Bill and Jason to wash and wax one car is '12/7' hours, but if we convert this to the mixed fraction '1 and 5/7', it's easier to understand
Notes
-

-

- We know the time it takes for each to wash and wax one car on their own
- Bill can wash and wax a car in 3 hours
- Jason can wash and wax a car in 4 hours
-

- We're looking for the time it takes Bill and Jason to wash and wax one car together
-

- So, 't' is the variable we're trying to solve for, and represents the 'time' it takes the two boys to wash and wax a car together
-

- Remember, 'time' will be represented by the variable 't'
-

- Remember, 't' is the variable we're trying to solve for, and represents the 'time' it takes the two boys to wash and wax a car together
-

- For Bill alone, "1 car = 3 hrs"
-

- For Bill alone, "1 car = 3 hrs"
- If we want to see what Bill can accomplish in one hour, divide by 3 to get: "1/3 car = 1 hr"
-

- For Bill alone, "1 car = 3 hrs"
- If we want to see what Bill can accomplish in one hour, divide by 3 to get: "1/3 car = 1 hr"
- So for any amount of time, 't', 't/3' will represent the number of cars Bill can wash and wax in 't' hours
-

- For Jason alone, "1 car = 4 hrs"
-

- For Jason alone, "1 car = 4 hrs"
- If we want to see what Jason can accomplish in one hour, divide by 4 to get: "1/4 car = 1 hr"
-

- For Jason alone, "1 car = 4 hrs"
- If we want to see what Jason can accomplish in one hour, divide by 4 to get: "1/4 car = 1 hr"
- So for any amount of time, 't', 't/4' will represent the number of cars Jason can wash and wax in 't' hours
-

- Since we don't know 't', we'll use 't/3' for Bill and 't/4' for Jason
- Combining them just means adding them together
-

- Since we don't know 't', we'll use 't/3' for Bill and 't/4' for Jason
- Combining them just means adding them together
- t/3 + t/4 = 1
-

- t/3 + t/4 = 1
- This is a rational equation
-

- Our equation is: 't/3 + t/4 = 1'
- This is a rational equation
- Remember, 't' is the variable we're trying to solve for, and represents the 'time' it takes the two boys to wash and wax a car together
-

- Our equation is: 't/3 + t/4 = 1'
- This is a rational equation
-

- Our equation is: 't/3 + t/4 = 1'
- This is a rational equation
- Our two fractions are 't/3' and 't/4'
- '3' and '4' are the denominators of our fractions
- The smallest number that '3' and '4' are both factors of is '12'
-

- Our equation is: 't/3 + t/4 = 1'
- This is a rational equation
-

- Up to this point, our equation looks like: '12(t/3 + t/4) = 12(1)'
- 12(t/3 + t/4) = 12(t/3) + 12(t/4) = 4t + 3t
- 12(1) = 12
-

- Up to this point, our equation looks like: '4t + 3t = 12'
- Remember, 't' is the variable we're trying to solve for, and represents the 'time' it takes the two boys to wash and wax a car together
-

- Up to this point, our equation looks like: '4t + 3t = 12'
- '4t' and '3t' are like terms!
- Combining them just means adding them together: 4t+3t=7t
-

- Up to this point, our equation looks like: '7t = 12'
- If we divide one side by '7', we must divide the other side by '7'
- 7t/7 = t
-

- Remember, 't' is the variable we're trying to solve for, and represents the 'time' it takes the two boys to wash and wax a car together
- We found 't' to equal '12/7 hours'
-

- '12/7' is not very easy to understand
- In this case, a mixed fraction form of '12/7' makes more sense
-

- '12/7' can be expressed as '1 and 5/7'
- Since we're dealing with hours, "one and five-sevenths hours" sounds better than "twelve-sevenths hours"