
www.VirtualNerd.com
How Do You Know if You Have a Difference of Squares?
How to identify a difference of squares problem
Summary
- 'Difference' means subtraction
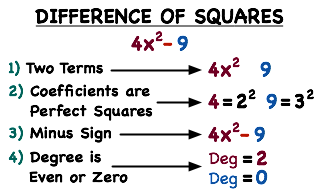
- 4x2-9 is an example of a difference of squares
- The two terms are 4x2 and 9
- A perfect square gives a whole number when you take its square root
- 4 and 9 are the coefficients of our terms
- The square root of 4 is 2 and the square root of 9 is 3
- The degree is the sum of all the exponents of a term
- The degree of 4x2 is 2 and the degree of 9 is 0
Notes
-

- 'Difference of squares' means we will be subtracting two perfect squares
-

- 4x2 and 9 are two perfect squares that are being subtracted
-

-

-

-

- 4x2 and 9 are the terms of this polynomial
-

- 4x2 and 9 are the terms of this polynomial
-

- 4x2 and 9 are the terms of this polynomial
-

- A perfect square is a number that gives a whole number when you take the square root
-

- A perfect square is a number that gives a whole number when you take the square root
-

- Our coefficients are 4 and 9
-

- 4 is the same as 2•2, or 22
- So 4 is a perfect square
-

- 4 is the same as 2•2, or 22
- So 4 is a perfect square
-

- 9 is the same as 3•3, or 32
- So 9 is a perfect square
-

- 9 is the same as 3•3, or 32
- So 9 is a perfect square
-

- We are looking for a DIFFERENCE of squares
- Since 'difference' means subtraction, we need a MINUS sign between our terms
-

- We are looking for a DIFFERENCE of squares
- Since 'difference' means subtraction, we need a MINUS sign between our terms
-

- We are subtracting 4x2 and 9, so we have a difference
-

- The degree of a monomial or term is just the sum of all the exponents of all the variables of that term
-

- Our terms are 4x2 and 9
-

- The exponent on x is 2, so its degree is 2
- Since 2 is EVEN, it fits the characteristic
-

- Not having a variable is the same as having a variable to the 0 power, since anything to the 0 power is 1
-

- Anything to the 0 power is 1
-
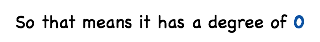
- So 9 has a degree of 0
-
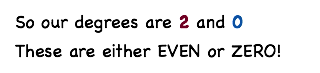
- Both of our terms have degrees that are even or zero, since 2 is even and 0 is zero
-
