
www.VirtualNerd.com
What's the Standard Form of a Polynomial?
What's the standard form of a polynomial?
Summary
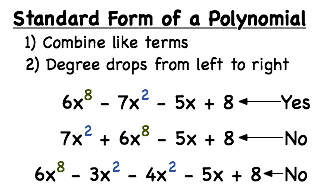
- 6x8-7x2-5x+8 is in standard form because all like terms are combined and the degree drops from 8 to 2, to 1, and to 0
- 7x2+6x8-5x+8 is in not in standard form because the degrees do not drop from left to right, 2 goes up to 8
- 6x8-3x2-4x2-5x+8 is not in standard form because all like terms are not combined
- There are two x2 terms
Notes
-

-

-

- 'x' is a variable
-

- Remember, to be in standard form all like terms must be combined, and the degree of each term must drop as we move from left to right
-

- Remember, to be in standard form all like terms must be combined, and the degree of each term must drop as we move from left to right
-

-

- These represent the type of terms in 6x8-7x2-5x+8
- 'x' is a variable
- There is only one x8 term
- There is only one x2 term
- There is only one 'x' term
- There is only one constant
-

-

- The degrees of 6x8-7x2-5x+8 go from 8, to 2, to 1, to 0
-

- Going from 8 to 2, to 1, and then to 0 satisfies the second criterion
-

- Going from 8 to 2, to 1, and then to 0 satisfies the second criterion
-

- Remember, to be in standard form all like terms must be combined, and the degree of each term must drop as we move from left to right
-

- 'x' is a variable
-

- Remember, to be in standard form all like terms must be combined, and the degree of each term must drop as we move from left to right
-

- Remember, to be in standard form all like terms must be combined, and the degree of each term must drop as we move from left to right
-

-

- These represent the type of terms in 7x2+6x8-5x+8
- 'x' is a variable
- There is only one x2 term
- There is only one x8 term
- There is only one 'x' term
- There is only one constant
-

-

-

-

- In standard form, the degrees can only go down from left to right, so this polynomial is not in standard form
-

- In standard form, the degrees can only go down from left to right, so this polynomial is not in standard form
-

-

- Remember, to be in standard form all like terms must be combined, and the degree of each term must drop as we move from left to right
-

-

-

- These represent the type of terms in 6x8-3x2-4x2-5x+8
- 'x' is a variable
- There is only one x8 term
- There are two x2 terms
- There is only one 'x' term
- There is only one constant
-

- The first criterion says that we cannot have like terms that are not combined in the polynomial
- -3x2 and -4x2 are like terms
- There are two x2 terms
- 'x' is a variable
-

- The first criterion says that we cannot have like terms that are not combined in the polynomial
-

-
