
www.VirtualNerd.com
How Do You Factor a Common Factor Out Of a Difference of Squares?
Factor 8x7-18xy2
Summary
- Factor out a greatest common factor of 2x
- The square root of 4, 2, is the coefficient of the first terms
- The square root of 9, 3, is the coefficient of the second terms
- In the first terms the variable is still x, and the exponent is half the degree, which is 3
- In the second terms the variable is still y, and the exponent is half the degree, which is 1
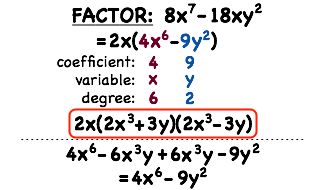
Notes
-

-

-

- Our polynomial is 8x7-18xy2
-

- 8 factors into 2•2•2
- 18 factors into 2•3•3
-

- x7 is the same as multiplying x by itself 7 times
- So each of our terms has at least one factor of x
-

- There is a factor of 2 and x in each of our terms
- This means we can factor them out of both terms
-

- To find the greatest common factor, just multiply together all the common factors
- In our case, the GCF is 2•x, or 2x
-

- To factor out the GCF, just divide it by each term in the polynomial
-
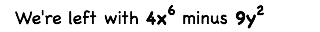
- 8x7/2x=4x6
- -18xy2/2x=-9y2
-

- Now that we've factored out the GCF, 2x, we need to factor what's left, 4x6-9y2
-

- The square root of 4 is 2
- The square root of 9 is 3
-
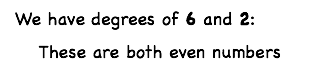
- The degree of x6 is 6
- The degree of y2 is 2
-

- Since both our coefficients are perfect squares and our degrees are even, we can use difference of squares
-

- We can use difference of squares to factor the polynomial
-

- 4x6 is the first term in our polynomial
- Its coefficient is 4
- Its variable is x
- Its degree is 6
-

- 9y2 is the second term in our polynomial
- Its coefficient is 9
- Its variable is y
- Its degree is 2
-

- When we factor the difference of two squares, it will always be an addition multiplied by a subtraction
-

- Remember, our coefficients were 4 and 9
- The coefficients of our new terms will be the square roots of 4 and 9
-

- 2 will be the coefficient of the first terms in our new binomials
-

- 3 will be the coefficient of the second terms in our new binomials
-

- Remember, our degrees were 6 and 2
- So half of 6 is 3 and half of 2 is 1
-

-

- We keep the same variable we had in the first term, x
- The 3 we got when we divided the degree in half is the new exponent
-

-

- We keep the same variable we had in the second term, y
- The 1 we got when we divided the degree in half is the new exponent
-

-

- We can do this by FOILing the binomials back together
-

- We can do this by FOILing
- Remember, FOIL is a method we use to multiply two binomials
-
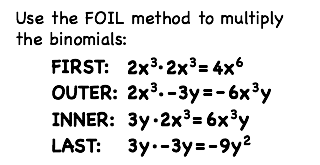
- We need to multiply the first, outer, inner, and last terms of our binomials together
- Multiply the first terms, 2x3•2x3, to get 4x6
- Multiply the outer terms, 2x3•-3y, to get -6x3y
- Multiply the inner terms, 3y•2x3, to get 6x3y
- Multiply the last terms, 3y•-3y, to get -9y2
-

- -6x3y and 6x3y are like terms, because they have the same variables to the same degrees
- -6x3y+6x3y=0
- So the two terms cancel out, leaving us with 4x6-9y2
-

- Remember, we had a 2x out in front of our polynomial before we factored it
-

- The 2x is still going to be part of our factored polynomial
-

-
