
www.VirtualNerd.com
What's FOIL?
Definition: FOIL
Summary
- FOIL is an acronym that tells us the steps we need to multiply two binomials
- a+b and c+d are binomials
- Multiply the first terms, ac
- Multiply the outer terms, ad
- Multiply the inner terms, bc
- Multiply the last terms, bd
- 4x+2 and 2x-3 are binomials
- 4x•2x are the first terms
- 4x•-3 are the outer terms
- 2•2x are the inner terms
- 2•-3 are the last terms
- Multiply out each term to simplify
- -12x+4x=-8x
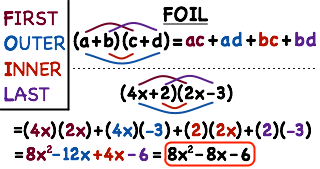
Notes
-

-

- A binomial is a polynomial with two terms
-

-

- First we multiply the first terms of each binomial together
- Then we multiply the first term of the first binomial by the second term of the second binomial
- Then we multiply the second term of the first binomial by the first term of the second binomial
- Finally we multiply the second terms of each binomial together
-

-

- a+b and c+b are binomials, because they each have two terms
-

- Our FIRST terms are 'a' and 'c'
- Multiply 'a' and 'c' together to get 'ac'
-

- Our OUTER terms are 'a' and 'd'
- Multiply 'a' and 'c' together to get 'ad'
-

- Our INNER terms are 'b' and 'c'
- Multiply 'b' and 'c' together to get 'bc'
-

- Our LAST terms are 'b' and 'd'
- Multiply 'b' and 'd' together to get 'bd'
-

-

- 4x+2 and 2x-3 are binomials, because they each have two terms
-

- The first terms are 4x and 2x
- This gives us the first term in our polynomial
-

- The outer terms are 4x and -3
- This gives us the second term in our polynomial
-

- The inner terms are 2 and 2x
- This gives us the third term in our polynomial
-

- The last terms are 2 and -3
- This gives us the fourth term in our polynomial
-

- Multiply out each of the terms in the polynomial to simplify
-

- -12x and 4x are like terms, because they both have x raised to the first power
- Combining -12x and 4x gives us -8x
-

-

- First multiply the first terms
- Then multiply the outer terms
- Then multiply the inner terms
- Then multiply the last terms