
www.VirtualNerd.com
How Do You Find the Degree of a Monomial?
Definition: Degree of a Monomial
Summary
- Taking the sum means we'll add all the exponents of all the variables
- 3y3 is a monomial with 1 variable: y3
- 7x2y3z is a monomial with 3 variables: x2, y3, and z
- 12 is a monomial with no variables
- The degree of 3y3 is 3
- The 'z' in 7x2y3z has an invisible exponent of 1
- The degree of 7x2y3z is 6
- The degree of 12 is 0
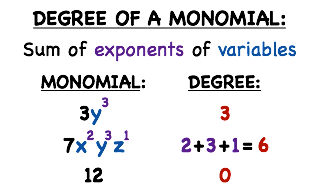
Notes
-

-

-

-

-

- These examples are all monomials, because nothing is being added or subtracted
-

-

- The variable is y and its exponent is 3
-

- Since there is only one exponent, there is nothing to add!
- So our degree is just the value of the exponent, 3
-

-

- The variables here are x, y, and z
- x has an exponent of 2
- y has an exponent of 3
- z has an invisible exponent of 1
- If a variable has no exponent, that means its exponent is actually 1, we just don't write it
-

- So we'll need to add together 2+3+1
-

- 2+3+1=6
-

- 12 has no variables, but it's still a monomial
-

- Since there are no variables, there are no exponents!
- So the degree will be 0
-
