www.VirtualNerd.com

How Do You Find the Area of a Rectangle Whose Sides are Binomials?

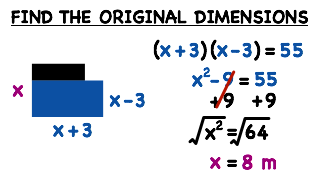
Jamey decided to replant her square garden as a rectangle. She cut out three meters worth of plants on one side and added it to another side. If the new area of the garden is 55 square meters, what were the lengths of the original sides?

Summary

  1. x represents the length of one side of the original square garden
  2. 3 meters were added to the length of the garden, so the new length is x+3
  3. 3 meters were subtracted from the width of the garden, so the new width is x-3
  4. Area equals length times width
  5. The area of the new garden was 55
  6. The formula for the product of a sum and a difference is (a+b)(a-b)=a2-b2

Notes

    1. This is what we are trying to find
    1. x is a variable that will represent one side of the original square garden
    1. Remember, squares have all sides the same length
    2. So x is both the length and the width
    1. Moving this section changes the dimensions of the garden
    2. So we no longer have a square, and the length and width are no longer equal
    1. The rectangular garden will have new dimensions, because the length and width are no longer equal
    1. The rectangular garden will have new dimensions, because the length and width are no longer equal
    1. Jamey cut 3 meters off the top of the garden and moved it to the side
    2. So the garden is now 3 meters longer than it was originally
    3. This means we need to add 3 meters to the original length, x
    4. So x+3 will be the length of the new garden
    1. The 3 meters she moved to the side of the garden were taken off the top
    2. So our width is now 3 meters shorter
    3. To get the new width, we need to subtract 3 meters from the original width, x
    4. So our new width is x-3
    1. Since we know the area, length, and width of the new garden, we can use the area equation to solve for x
    2. Remember, area equals length times width
    1. Remember, area equals length times width
    1. When we plug our length, width, and area into the equation, we get (x+3)(x-3)=55
    1. Remember, x represents the length of a side of the original garden
    2. Now we can solve this equation for x to find the original dimensions
    1. Remember, x represents the length of a side of the original garden
    1. Since x+3 is a sum and x-3 is a difference, we can use this formula to find their product instead of multiplying it out
    2. Remember, a product is the result you get when you multiply
    3. We can plug 'x' in for 'a' and '3' in for 'b' in the formula
    4. So we'll have x2-32
    1. 32=9
    2. So the product of x+3 and x-3 is x2-9
    1. Remember, x represents the length of a side of the original garden
    1. Addition is the opposite of subtraction
    2. So add 9 to both sides to undo the subtraction
    3. The 9's cancel on the left to leave just x2
    4. 55+9=64 on the right hand side
    1. To undo a square, we need to take the square root
    2. The square root of x2 is just x
    3. The square root of 64 is 8
    1. Remember, x represents the length of a side of the original garden
    2. So this means that each side of the original square garden was 8 meters