
www.VirtualNerd.com
How Do You Find the Volume of a Box Whose Sides are Monomials?
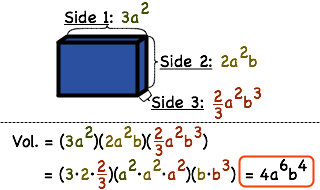
Find the volume of a rectangular box whose sides have the following lengths:
Side 1 = 3a2
Side 2 = 2a2b
Side 3 = 2/3 • a2b3
Write your answer as a polynomial in simplest form.
Summary
- 'a' and 'b' are variables
- Notice that each side is a monomial
- Use the associative property of multiplication to group terms together
- When multiplying variables we can just add the exponents
- a2•a2•a2=a2+2+2=a6
- b•b3=b1+3=b4
Notes
-

-

-

- 'a' and 'b' are variables
- The length is side 1, 3a2
- The width is side 2, 2a2b
- The height is side 3, 2/3 • a2b3
-

- The volume of the box is (side 1)•(side 2)•(side 3)
-

- 'a' and 'b' are variables
- Notice that the sides are monomials
-

-

-

-

- The constants were 3, 2 and 2/3
-

- 'a' is a variable
- Our 'a' terms were a2, a2 and a2
-

- 'b' is a variable
- Our 'b' terms were b and b3
-

- First let's multiply the constants together
- The constants are 3, 2 and 2/3
-

- We can just add the exponents together to find the product
- 'a' is a variable
- a2•a2•a2=a2+2+2=a6
-

- We can just add the exponents together to find the product
- 'b' is a variable
- 'b' is the same as b1
- b•b3=b1+3=b4
-

- 'a' and 'b' are variables