
www.VirtualNerd.com
How Do You Figure Out if an Absolute Value Inequality is an AND or OR Compound Inequality?
Determine if these absolute value inequalities represent AND or OR compound inequalities: |x-5| < 3 and |x-5| > 3
Summary
- A pair of vertical lines represents an absolute value
- For absolute value inequalities:
- '<' tells you it will be an AND compound inequality
- '>' tells you it will be an OR compound inequality
- Open circles represent '<' or '>' symbols
- Notice that the inequality symbol points in the same direction as the arrow in our graph
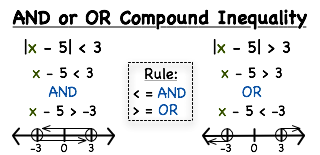
Notes
-

-

- It is important to recognize what type of compound inequality an absolute value inequality will breakdown into
-

- For absolute value inequalities, '<' means you will have an AND compound inequality
-

- For absolute value inequalities, '>' means you will have an OR compound inequality
-

- For absolute value inequalities:
- '<' means you will have an AND compound inequality
- '>' means you will have an OR compound inequality
-

- The first absolute value inequality is '|x-5|<3'
-

- For absolute value inequalities:
- '<' means you will have an AND compound inequality
- The '<' symbol means LESS THAN
-

- Since we're dealing with an absolute value, we have two options for inequalities
-

- The absolute value inequality is '|x-5|<3'
- Taking away the absolute value symbols, we get 'x-5<3'
-

- The absolute value inequality is '|x-5|<3'
- Taking away the absolute value symbols, we get 'x-5<3'
- Flipping the inequality symbol and changing the sign on the right, we get 'x-5>-3'
-

- Our compound inequality is 'x-5<3 AND x-5>-3'
-

- The second absolute value inequality is '|x-5|>3'
-

- For absolute value inequalities:
- '>' means you will have an OR compound inequality
- The '>' symbol means GREATER THAN
-

- Since we're dealing with an absolute value, we have two options for inequalities
- The absolute value inequality is '|x-5|>3'
- Taking away the absolute value symbols, we get 'x-5>3'
- Flipping the inequality symbol and changing the sign on the right, we get 'x-5<-3'
- Our compound inequality is 'x-5>3 OR x-5<-3'
-

- The absolute value inequalities are '|x-5|<3' and '|x-5|>3'
- Our compound inequalities are 'x-5<3 AND x-5>-3' and 'x-5>3 OR x-5<-3'
-

- The absolute value inequalities are '|x-5|<3' and '|x-5|>3'
- Our compound inequalities are 'x-5<3 AND x-5>-3' and 'x-5>3 OR x-5<-3'
-

- Our first compound inequality is 'x-5<3 AND x-5>-3'
-

- Our first compound inequality is 'x-5<3 AND x-5>-3'
-

- Our first compound inequality is 'x-5<3 AND x-5>-3'
- Remember that we use open circles for inequalities with '<' or '>'
- Notice the arrow we're drawing points in the same direction as the inequality symbol
-

- Our first compound inequality is 'x-5<3 AND x-5>-3'
- Remember that we use open circles for inequalities with '<' or '>'
- Notice the arrow we're drawing points in the same direction as the inequality symbol
-

- Our first compound inequality is 'x-5<3 AND x-5>-3'
- Remember that we use open circles for inequalities with '<' or '>'
- Notice the arrow we're drawing points in the same direction as the inequality symbol
-

- Our first compound inequality is 'x-5<3 AND x-5>-3'
- The absolute value inequality is '|x-5|<3'
-

- Our first compound inequality is 'x-5<3 AND x-5>-3'
- The absolute value inequality is '|x-5|<3'
-

- Our first compound inequality is 'x-5<3 AND x-5>-3'
- The absolute value inequality is '|x-5|<3'
- So our graph and absolute value inequality agree!
-

- Our graph and absolute value inequality agree!
-

- Our second compound inequality is 'x-5>3 AND x-5<-3'
-

- Our second compound inequality is 'x-5>3 AND x-5<-3'
- Remember that we use open circles for inequalities with '<' or '>'
- Notice the arrow we're drawing points in the same direction as the inequality symbol
-

- Our second compound inequality is 'x-5>3 AND x-5<-3'
- Remember that we use open circles for inequalities with '<' or '>'
- Notice the arrow we're drawing points in the same direction as the inequality symbol
-

- Our second compound inequality is 'x-5>3 AND x-5<-3'
- Remember that we use open circles for inequalities with '<' or '>'
- Notice the arrow we're drawing points in the same direction as the inequality symbol
-

- Our second compound inequality is 'x-5>3 AND x-5<-3'
- Remember that we use open circles for inequalities with '<' or '>'
- Notice the arrow we're drawing points in the same direction as the inequality symbol
- Our graph and absolute value inequality agree!
-

- "The absolute value of x minus 5 will always be GREATER THAN 3" can be written as '|x-5|>3'
- Our graph and absolute value inequality agree!
-

-

- For absolute value inequalities:
- '<' means you will have an AND compound inequality
- '>' means you will have an OR compound inequality
- The '<' symbol means LESS THAN
- The '>' symbol means GREATER THAN