www.VirtualNerd.com

How Do You Solve an Inequality Where You're Multiplying Negative Fractions?

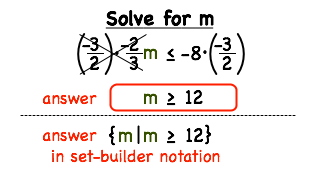
Solve the inequality for m: (-2/3)m ≤ -8

Summary

  1. The '≤' symbol means LESS THAN OR EQUAL TO
  2. Multiplying '-2/3' by its reciprocal, -3/2, will give us 'm'
  3. Remember, we have to multiply both sides by -3/2
  4. (-3/2)•(-2/3) = 1
  5. -8•(-3/2) = 12
  6. Multiplying two negative numbers produces a positive number
  7. The '≥' symbol means GREATER THAN OR EQUAL TO
  8. The inequality symbol flips when we multiply or divide by a negative number

Notes

    1. 'm' is just the variable we are trying to solve for
    1. Solving for 'm' means we want it by itself
    1. This property says that what we do to one side of the inequality, we must do to the other side
    2. It also reminds us that multiplying by a negative number will cause the inequality sign to flip
    1. In order to get rid of the '-2/3' on the left, we need to perform the opposite operation that is being done
    2. Multiplying a number by its reciprocal is performing the opposite operation!
    1. This means we just swap the numerator and the denominator
    1. -3/2 is the reciprocal of '-2/3'
    1. Remember, what we do to one side of the inequality, we must do to the other side!
    1. On the left-hand side, we have '(-3/2)•(-2/3)m'
    1. We multiply -3/2 by '-2/3':
    2. (-3/2)•(-2/3) = 1
    3. Multiplying two negative numbers together produces a positive number
    1. Remember, we had to multiply the right side by -3/2, as well!
    2. -8•(-3/2) = 12
    1. The multiplication property of inequality says that multiplying by a negative will flip the inequality sign
    2. We multiplied by -3/2, a negative number!
    1. The multiplication property of inequality says that multiplying by a negative will flip the inequality sign
    2. We multiplied by -3/2, a negative number!
    3. Preserving an inequality just means making sure the inequality still reads true
    1. 'm' is just the variable we are trying to solve for
    2. Our final answer is 'm≥12'
    3. The '≥' symbol means GREATER THAN OR EQUAL TO
    1. Our answer was 'm≥12'
    1. Opening up our set just means we need to draw a left curly brace, '{'
    2. Curly braces tell us we are dealing with a set
    3. The vertical line, '|', means we're about to define what numbers 'm' represents in the set
    1. Our answer was 'm≥12'
    2. This goes after the '{m |'
    1. Closing our set means we need to draw a right curly brace, '}'
    2. Curly braces tell us we are dealing with a set
    3. Our answer in set-builder notation is: '{m | m≥12}'