www.VirtualNerd.com

How Do You Solve an Inequality with Negative Numbers Using Multiplication?

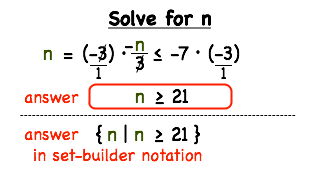
Solve the inequality for n:
(-n/3) ≤ -7

Summary

  1. We multiply by -3 to undo the division by '3' on the left side, leaving us with 'n' alone
  2. Since we multiplied the left side by -3, we have to multiply the right side by -3, which gives us '-7 •(-3)', or 21
  3. Recognize that -3 is the same as -3/1
  4. Multiplying or dividing two negatives makes a positive
  5. The inequality symbol flips when we multiply or divide by a negative number

Notes

    1. 'n' is a variable that we want to solve for
    1. We're trying to get 'n' by itself on the left side
    2. 'n' is a variable that we want to solve for
    1. Multiplication is the opposite of division
    1. Multiplication is the opposite of division
    2. Multiplying by 3 will cancel out the division by '3'
    1. This property states that whatever we multiply one side of the inequality by, we must multiply the other side by
    1. Multiplying by -3 will cancel out the division by '3' and the negative sign on the left side
    2. Remember that multiplying or dividing two negatives makes a positive
    1. Multiplying by -3 will cancel out the division by '3' and the negative sign on the left side
    2. Multiplying by -3 will give us '-7•(-3)'
    3. Remember that multiplying or dividing two negatives makes a positive
    1. Recognizing that -3 is the same as -3/1 will make multiplication of fractions on the left side of the inequality easier
    1. Remember that multiplying or dividing two negatives makes a positive
    2. (-3/1)•(-n/3) = n/1 = n
    1. Remember that multiplying or dividing two negatives makes a positive
    2. (-3/1)•(-n/3) = n/1 = n
    1. Remember, we had to multiply both sides by -3
    2. -7•(-3) = 21
    3. Multiplying or dividing two negatives makes a positive
    1. We need to ask ourselves if we multiplied by a negative number
    2. Remember that "product" means the same as "multiplication"
    3. We did multiply by a negative number, -3!
    1. Preserving an inequality is just making sure the inequality still reads true
    1. The inequality 'n≥21' is our answer
    2. Notice that the inequality symbol flipped since we multiplied by a negative number
    1. The inequality 'n≥21' is our answer
    1. Opening up a set just means we need to draw a left curly brace, '{'
    2. Curly braces tell us we're dealing with a set
    3. Here, 'n' is representing the set of numbers defined by the inequality we got for our answer
    1. So after '{n |', we insert our answer
    2. This gives us '{n | n≥21'
    1. To close a set, just draw a right curly brace, '}'
    2. Our answer in set-builder notation is '{n | n≥21}'