
www.VirtualNerd.com
How Do You Use a Proportion to Find What Percent a Part is of a Whole?
68 is what percent of 85?
Summary
- '%' stands for percent
- In math, 'is' means 'equals'
- Since we don't know what the percent is, we'll call that 'x'
- In math, 'of' means multiplication
- In a percent proportion we put the percent over 100
- Then we set that equal to the part over the whole
- Here our part is 68 and our whole is 85, so we have 68/85
- We can cross multiply to solve for 'x'
- 68 is 80% of 85
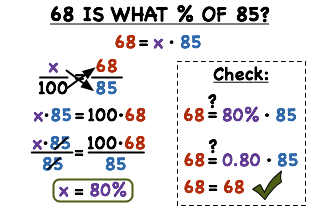
Notes
-

- First we want to rewrite our problem using math symbols
-

- '68 is what percent of 85?'
- In math, 'is' means 'equals'
- 'What percent' is our unknown: 'x'
- 'of' means we multiply
- The equation ends up being 68 = x • 85
-

- Using a percent proportion is one way we can solve for 'x'
-

- In our problem, our 'x' variable represents the percent
-

- We can identify each piece in the percent proportion from our equation
-

- 'x' is the variable representing the percent
-

-

- 68 is a percent of 85, which means it's part of 85
-

- Since the 68 is coming out of 85, 85 is the 'whole'
-

-

- Remember, 'x' is the variable representing the percent
- 85 is the 'whole' in our percent proportion
-
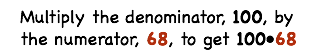
- 68 is the 'part' in our percent proportion
-

- Now we can just simplify to find 'x', our percent!
-

- 'x' is the variable representing the percent
-

- 100•68 is 6800
- When we divide this by 85, we get 80
-

- 'x' is the variable representing the percent
- So 80 is the value of our percent
- But we need to make sure we put the percent symbol, %, after the 80 to show that it's a percent!
-

- We can plug our answer back into the original equation to make sure it's correct
-

- 'x' is the variable representing the percent
- '%' stands for percent
-

- We can't multiply percents, so we need to change 80% to a decimal first
- Just move the decimal point to the left two places and drop the percent symbol
-

- Since we got the same thing on both sides, our answer must be correct!