
www.VirtualNerd.com
How Do You Use the Formula for Simple Interest?
Denzel opened a savings account that made 9% annual interest. After 18 months, she had made $243 in interest. How much money did she put into her savings account?
Summary
- I, p, r, and t are variables in the formula for simple interest
- I represents the interest earned
- p represents the amount of money originally invested
- r represents the interest rate
- t represents time in years
- I, the amount of interest, is 243
- p is what we're solving for, the amount invested
- r, the interest rate, is 9%, which is 0.09 as a decimal
- t, time, is 18 months, which is 1.5 years
- Plug the values into the formula and solve for p
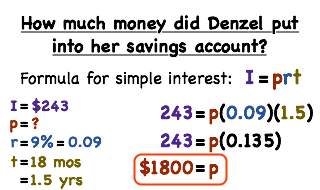
Notes
-

-

-
- The formula for simple interest states that interest is equal to the amount of money invested times the interest rate times time
- We can use this formula to figure out how much money Denzel invested
-

-

- I is the amount of interest
- p is the amount of money invested
- r is the interest rate
- t is time in years
-

- The formula for simple interest is I=prt
- I, p, r, and t are the variables in the formula
-

-

- I is a variable that represents interest earned
-

- I is a variable that represents interest earned
- I=243
-

- p is a variable that represents the amount of money invested
-

- This is our unknown value
- So we will need to use our formula to solve for p
-

- r is a variable that represents the rate of interest
-

- r is a variable that represents the rate of interest
- Our problem gives us an interest rate of 9%
-

- When we are working with formulas, we can't use percents as percents
- But we can convert any percent to a decimal, which we CAN use
-

- To convert a percent to a decimal, take the number of the percent and move the decimal two places to the left
- Since 9 is a whole number, its decimal comes right after the number
- So moving the decimal two places to the left gives us 0.09
- This is a value we can use for r, the interest rate
-

- t is a variable that represents time in years
-

- t is a variable that represents time in years
- Notice that our problem gives time in months
- Since the formula needs time in years, we'll have to convert 18 months to years
-

- t is a variable that represents time in years
- Notice that our problem gives time in months
- Since the formula needs time in years, we'll have to convert 18 months to years
-

- t is a variable that represents time in years
- Divide 18 months by 12 months in a year to get 1.5 years
- So we can use 1.5 for t
-

-

- I, the interest earned, is 243
- p, the amount Denzel invested, is our unknown
- r, the interest rate (as a decimal), is 0.09
- t, time (in years), is 1.5
-

- Now we simplify and solve for p, the amount of money invested
- We can simplify the right hand side by multiplying 0.09 by 1.5
- So we get 0.135 times p on the right
-
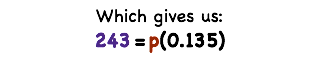
- p is the variable we're solving for, the amount of money invested
-

- p is the variable we're solving for, the amount of money invested
-

- Division is the opposite of multiplication
- So to undo the multiplication and get p by itself, we need to divide both sides by 0.135
-

-

- p, our unknown, is now by itself on the right hand side
- We found the left hand side to be 1800, so this is equal to p
- So we have solved for p, the amount of money invested: p=1800
-
