
www.VirtualNerd.com
How Do You Solve Two Equations with Two Variables?
Solve for a and b given:
a = 23 - 2b
3a + 3b = 48
Summary
- 'a' and 'b' are variables
- Plug (23-2b) in for 'a' in the second equation
- Distribute the 3, and combine the 'b' terms together
- Solve for 'b' by subtracting 69 from both sides and divide by -3
- b=7 and a=9
- Plug 7 in for 'b' to find 'a'
- Check our answers by plugging them into the second equation
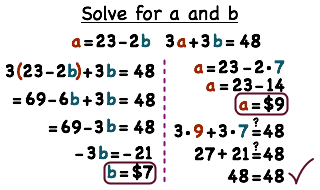
Notes
-

- 'a' is a variable
-

- The second equation is '3a+3b=48'
-

- 'a' and 'b' are variables
- The right hand side of the first equation is: 23-2b
- This is equal to 'a'
-

-

- 'b' is a variable
- To distribute the 3, multiply it by 23 and then -2b
-

- To distribute the 3, multiply it by 23
- 3 times 23 is 69
-

- 'b' is a variable
- To distribute the 3, multiply it by 23 and then -2b
- 3 times -2b is -6b
-

-

- 'b' is a variable
-

- 'b' is a variable
- Negative 6b plus 3b is equal to negative 3b
-

-

- The positive and negative 69 cancel on the left hand side
-

- The positive and negative 69 cancel on the left hand side
- 48 minus 69 is negative 21
-

- The -3's cancel each other out on the left hand side
- -21 divided by -3 is equal to 7
-

-

- 'a' is a variable
-

- 'b' is a variable
- Anywhere you see a 'b' put a 7
-

- 2 • 7 = 14
-
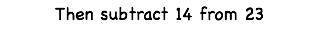
- 23 - 14 = ?
-

- 23 - 14 = 9
-

-

-

- 3 • 9 = ?
- 3 • 7 = ?
-

- 3 • 9 = 27
- 3 • 7 = ?
-

- 3 • 9 = 27
- 3 • 7 = 21
-

- 27 + 21 = 48
-

- Plugging 7 in for 'b' and 9 in for 'a' into the second equation came out with a true statement: 48 = 48
- This means that our answers are correct!