
www.VirtualNerd.com
How Do You Solve a Word Problem Where You Multiply Fractions and Work Backwards?
Emilio has been eating the same sub for an entire week. He loves the sub so much that he doesn't want to finish it off, so each day he leaves one-fourth of the sandwich for the next day. If, on the fifth day, the sandwich was five sixty-fourths of a foot, how long was it when Emilio bought it?
Summary
- Since it's kind of hard to figure out where to start, starting with a simpler problem will help
- The length on the day before is 4 times the current length
- Making a table will help us work backwards to figure out the length of Emilio's sub
- The final length, '5/64 ft', of the sub is given to us
- So the sub was originally 20ft long, wow!

Notes
-

-

- Since it's kind of hard to figure out where to start, starting with a simpler problem will help
-

- Remember, we're looking at a simpler problem before moving onto the real problem
-

- Remember, we're looking at a simpler problem before moving onto the real problem
- Emilio always leaves 1/4 of the sandwich for the next day
-

- Remember, we're looking at a simpler problem before moving onto the real problem
- Emilio always leaves 1/4 of the sandwich for the next day
-

- Remember, we're looking at a simpler problem before moving onto the real problem
- Emilio always leaves 1/4 of the sandwich for the next day
-

- Dividing by 4 is the same as multiplying by 1/4
- Remember, we're looking at a simpler problem before moving onto the real problem
- Emilio always leaves 1/4 of the sandwich for the next day
-

-
4ft
• (1/4) = 1ft - So one-fourth of the sub on the first day is 1 foot
- Remember, we're looking at a simpler problem before moving onto the real problem
- Emilio always leaves 1/4 of the sandwich for the next day
-
4ft
-

-
1
• (1/4) = 1/4 - Dividing by 4 is the same as multiplying by 1/4
- Remember, we're looking at a simpler problem before moving onto the real problem
- Emilio always leaves 1/4 of the sandwich for the next day
-
1
-

-
1ft
• (1/4) = 1/4ft - Dividing by 4 is the same as multiplying by 1/4
- Remember, we're looking at a simpler problem before moving onto the real problem
- Emilio always leaves 1/4 of the sandwich for the next day
-
1ft
-

- So the trick is to multiply the current length by 4 to get the sub's length for the day before
-

-
(1/4ft)
• 4 = 1ft - So the trick is to multiply the current length by 4 to get the sub's length for the day before
- Remember, we're looking at a simpler problem before moving onto the real problem
-
(1/4ft)
-

-
(1ft)
• 4 = 4ft - So the trick is to multiply the current length by 4 to get the sub's length for the day before
- Remember, we're looking at a simpler problem before moving onto the real problem
-
(1ft)
-

- Now we can use our trick and apply it to the real problem
- We're given the sub's length on Day 5
-

- Making a table will help us work backwards to figure out the length of Emilio's sub on Day 1
-

- There are 5 days total
-

- We want to find the length of the sub on Day 1
-

- We want to find the length of the sub on Day 1
- We are given the length of the sub on day 5
-

- Here is where we use our trick of multiplying the current day by 4 to get the previous day's sub length
- We want to find the length of the sub on Day 1
- We are given the length of the sub on day 5
-

- Here is where we use our trick of multiplying the current day by 4 to get the previous day's sub length
- We want to find the length of the sub on Day 1
- We are given the length of the sub on day 5
-

- We are given the length of the sub on day 5, it's 5/64ft
-

- The length of the sub on Day 5 was 5/64ft
- So the length of the sub on Day 4 was 5/16ft
-

- The length of the sub on Day 4 was 5/16ft
- So the length of the sub on Day 3 was 5/4ft
-

- The length of the sub on Day 3 was 5/4ft
- So the length of the sub on Day 2 was 5ft
-
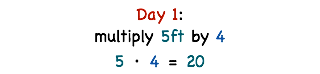
- The length of the sub on Day 2 was 5ft
- So the length of the sub on Day 1 was 20ft
-

- That's a big sandwich!