
www.VirtualNerd.com
How Do You Solve an Equation with Variables on Both Sides?
Solve 15 + 6x = 45 + 8x for x
Summary
- 'x' is our variable
- We have x's on both sides of the equation
- Subtract 6x from both sides to get all the x's on one side
- Subtract 45 from both sides to get the x term by itself
- Divide by 2 on both sides to solve for x
- We get x=-15
- Plug -15 in for x in the original equation
- Since -75=-75 is a true statement, our answer is correct
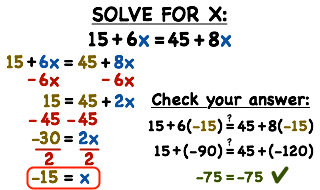
Notes
-

- 'x' is a variable
-

-

-

- 'x' is a variable
-

-

- 'x' is our variable
-

- 'x' is our variable
- The opposite of addition is subtraction
- So we need to subtract 6x from both sides of the equation
-

- 'x' is our variable
- We have 15+6x-6x on the left hand side
- The 6x's cancel out, leaving us with 15
- Then we have 45+8x-6x on the right hand side
- Subtract 8x-6x and we're left with 45+2x on the right hand side
-

-

- 15-45 gives us -30 on the left hand side
- 45-45+2x leaves us with 2x on the right hand side
-

- 'x' is our variable
- Now we have x by itself on one side of the equation
-

- 'x' is our variable
-

- 'x' is our variable
-

- Division is the opposite of multiplication
- So divide -30 by 2 and 2x by 2
-

- 'x' is our variable
- -30/2=-15
- 2x/2=x
-

-

- -15 is the value we got for our variable, x
- So we can plug -15 in wherever we had an x in our original equation
-

- -15 is the value we got for our variable, x
- So we can plug -15 in wherever we had an x in our original equation
-

- If we get a true statement, then our answer is correct
-

- -15 was the value we plugged in for x
-

- 15+(-90)=-75
- 45+(-120)=-75
- Since -75=-75, we get a true statement
-

- So -15, the value we found for x, is the correct answer!