
www.VirtualNerd.com
How Do You Turn a More Complicated Equation into Words?
Turn the following mathematical expression into a story. In other words make up a story that describes the mathematical expression!
2(a+2b)+(1/2)b2+2(a+3b) = m
Summary
- 'a', 'b', and 'm' are variables
- We'll have to figure out what the mathematical symbols, like + and 2, mean in English
- We got the phrase "two times" because we are multiplying 2 by something
- We're using the phrase "the sum of" because of the ( + )
- 'a' is equal to the number of Amit's hours
- 'b' is equal to the number of Betty's hours
- We're writing "squared" because of the 2
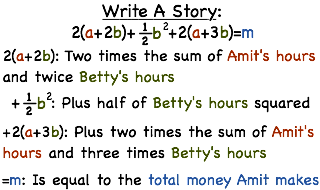
Notes
-

- The mathematical equation is 2(a+2b)+(1/2)b2+2(a+3b) = m
- Where 'a', 'b', and 'm' are variables
-
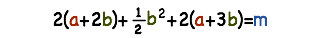
- 'a', 'b', and 'm' are variables
-

- The mathematical equation is 2(a+2b)+(1/2)b2+2(a+3b) = m
- Where 'a', 'b', and 'm' are variables
-

-

- 'a', 'b', and 'm' are variables
- Variables are symbols in mathematics that represent unknown numbers
- Since we're making the story for this equation, we can make the variables represent anything we want
-

- Our variables are 'a', 'b', and 'm'
- Variables are symbols in mathematics that represent unknown numbers
- Since we're making the story for this equation, we can make the variables represent anything we want
-

-

- Since 'a' is a variable, and we are creating the story, 'a' can mean ANYTHING we want it to mean
-

- Remember, 'a' is a variable that we are creating a story for, and the story can be anything we want having to do with numbers
-

- Remember, 'a' is a variable that we are creating a story for, and the story can be anything we want having to do with numbers
-

- Remember, 'a' is a variable that we are creating a story for, and the story can be anything we want having to do with numbers
-

- Remember, 'a' is a variable that we are creating a story for, and the story can be anything we want having to do with numbers
-

- Since 'b' is a variable, and we are creating the story, 'b' can mean ANYTHING we want it to mean
-

- Remember, 'b' is a variable that we are creating a story for, and the story can be anything we want having to do with numbers
-

- Remember, 'b' is a variable that we are creating a story for, and the story can be anything we want having to do with numbers
-

- 'b' is a variable that we are creating a story for, and the story can be anything we want having to do with numbers
- We're letting 'b' have something to do with Betty
-

- Remember, 'b' is a variable that we are creating a story for, and the story can be anything we want having to do with numbers
-

- Remember, 'b' is a variable that we are creating a story for, and the story can be anything we want having to do with numbers
-

- Since 'm' is a variable, and we are creating the story, 'm' can mean almost anything
- We just need to make sure it makes sense with the meanings we've chosen for the other variables
-

- Since 'm' is a variable, and we are creating the story, 'm' can mean almost anything
- We just need to make sure it makes sense with the meanings we've chosen for the other variables
-

- Since 'm' is a variable, and we are creating the story, 'm' can mean almost anything
- We just need to make sure it makes sense with the meanings we've chosen for the other variables
-

- Since 'm' is a variable, and we are creating the story, 'm' can mean almost anything
- We just need to make sure it makes sense with the meanings we've chosen for the other variables
- Using 'm' to represent money makes sense because the amount people get paid is often related to the number of hours they work
-

- Since we're writing the story, the variables could represent anything we want, not just the things we picked today
-

- We'll have to take a look at what the mathematical symbols, like + and 2, mean in English
-

- We'll have to take a look at what the mathematical symbols, like + and 2, mean in English
-

- We'll have to take a look at what the mathematical symbols, like + and 2, mean in English
- We defined 'a' to be the total amount of Amit's hours
- We defined 'b' to be the total amount of Betty's hours
-

- We'll have to take a look at what the mathematical symbols, like + and 2, mean in English
- We defined 'a' to be the total amount of Amit's hours
- We defined 'b' to be the total amount of Betty's hours
-

- We'll have to take a look at what the mathematical symbols, like + and 2, mean in English
- We got the phrase "two times" because we are multiplying 2 by something
-

- We got the phrase "two times" because we are multiplying 2 by something
-

- We got the phrase "two times" because we are multiplying 2 by the quantity (a+2b)
- 'a' and 'b' are the variables we defined earlier
-

- We got the phrase "two times" because we are multiplying 2 by the quantity (a+2b)
- 'a' and 'b' are the variables we defined earlier
- We're using the phrase "the sum of" because of the ( + )
- When you have something inside parentheses like ( + ), you have to do the + sign first, which is why we use "the sum of"
- "Sum" means addition
-

- We're using the phrase "the sum of" because of the ( + )
-

- We're using the phrase "the sum of" because of the ( + )
-

- 'a' and 'b' are the variables we defined before
- 'a' is equal to the number of Amit's hours
- 'b' is equal to the number of Betty's hours
-

- 'a' is a variable we defined before
-

- 'b' is a variable we defined before
-

- 'b' is a variable we defined before
- 'b' is equal to the number of Betty's hours
-

- The expression 2(a+2b) translates to "Two times the sum of Amit's hours and twice Betty's hours"
- 'a' and 'b' are the variables we defined before
- 'a' is equal to the number of Amit's hours
- 'b' is equal to the number of Betty's hours
-

- Remember, we're trying to translate this expression into words
-

-

-

- We're writing "plus" because of the addition sign, +
-

-

- We're writing "half" because of the 1/2
-

- We're writing "half" because of the 1/2
-
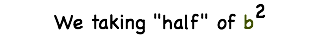
- We're writing "half" because of the 1/2
- 'b' is a variable we defined before
- 'b' is equal to the number of Betty's hours
-

- 'b' is a variable we defined before
- 'b' is equal to the number of Betty's hours
-

- Remember, we started with (1/2)b2
- 'b' is a variable we defined before
- 'b' is equal to the number of Betty's hours
-

- Remember, we started with (1/2)b2
- 'b' is a variable we defined before
- 'b' is equal to the number of Betty's hours
-

- Remember, we started with (1/2)b2
- The exponent 2 means that we are "squaring" 'b'
- 'b' is a variable we defined before
- 'b' is equal to the number of Betty's hours
-

- Remember, we started with (1/2)b2
- 'b' is a variable we defined before
- 'b' is equal to the number of Betty's hours
-

- 'a' and 'b' are the variables we defined before
- 'a' is equal to the number of Amit's hours
- 'b' is equal to the number of Betty's hours
-

-

- 'a' and 'b' are the variables we defined before
- 'a' is equal to the number of Amit's hours
- 'b' is equal to the number of Betty's hours
-

- The expression 2(a+2b) translates to "Two times the sum of Amit's hours and twice Betty's hours"
- 'a' and 'b' are the variables we defined before
- 'a' is equal to the number of Amit's hours
- 'b' is equal to the number of Betty's hours
-

- The first term is so similar to this one, we can use it to help us write the third term
- The expression 2(a+2b) translates to "Two times the sum of Amit's hours and twice Betty's hours"
- 'a' and 'b' are the variables we defined before
- 'a' is equal to the number of Amit's hours
- 'b' is equal to the number of Betty's hours
-

- The word "sum" is used to show addition
-

- 'a' and 'b' are the variables we defined before
- 'a' is equal to the number of Amit's hours
- 'b' is equal to the number of Betty's hours
-

- 'a' is a variable we defined before
- 'a' is equal to the number of Amit's hours
-

- 'b' is a variable we defined before
- 'b' is equal to the number of Betty's hours
-

- 'b' is a variable we defined before
- 'b' is equal to the number of Betty's hours
-

- Remember, we started with 2(a+3b)
- 'b' is a variable we defined before
- 'b' is equal to the number of Betty's hours
-

- 'm' is a variable we defined before
- 'm' is equal to the total amount of money Amit makes
-

-

- 'm' is a variable we defined before
- 'm' is equal to the total amount of money Amit makes
-

- 'm' is a variable we defined before
- 'm' is equal to the total amount of money Amit makes
-

- We're writing down "the total money Amit makes"
-

- Our equation was "2(a+2b)+(1/2)b2+2(a+3b) = m"
-

-

-

- The equation we translated was "2(a+2b)+(1/2)b2+2(a+3b) = m"
- 'a' is equal to the number of Amit's hours
- 'b' is equal to the number of Betty's hours
- 'm' is equal to the total amount of money Amit makes
-

- The equation we translated was "2(a+2b)+(1/2)b2+2(a+3b) = m"
- 'a' is equal to the number of Amit's hours
- 'b' is equal to the number of Betty's hours
- 'm' is equal to the total amount of money Amit makes
-

- You don't have to define the variables 'a', 'b', and 'm' in the same way we did
-

- You don't have to define the variables 'a', 'b', and 'm' in the same way we did
-

- You don't have to define the variables 'a', 'b', and 'm' in the same way we did