
www.VirtualNerd.com
How Do Different Categories of Numbers Compare To Each Other?
How do different categories of numbers compare to each other?
Summary
- Natural numbers are the counting numbers: 1, 2, 3, 4, ...
- Whole numbers are all the natural numbers including 0: 0, 1, 2, 3, ...
- Integers are the whole numbers plus negative counterparts: ..., -2, -1, 0, 1, 2, ....
- Rational numbers are numbers that you can write as fractions: 1/2, 1/3, -4/9, 1.75, 0.1111
- Irrational numbers are non-repeating, never-ending decimals, like
π and√ 2 - Real numbers are all numbers found on a number line
Notes
-

-

- These are the numbers 1, 2, 3, 4, and so on
-

- So instead of starting at 1, we start at 0
- Whole numbers are 0, 1, 2, 3, and so on
-

- So we have 0, 1, 2, 3 and so on
- But we also have -1, -2, -3 and so on with negatives as well
-

- Rational numbers are numbers that can be written as fractions
- So any number you can rewrite as a/b, where b is not 0, is a rational number
- 1.75 can be rewritten as 7/4, so it is a rational number
- 0.1111... can be rewritten as 1/9, so it is also rational
-

- Irrational numbers are non-repeating, never-ending decimals
- They CANNOT be rewritten as fractions
-

-

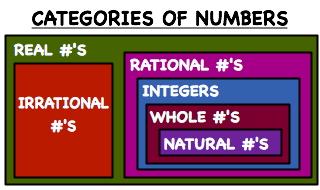
- This will help us understand how these categories relate to each other
-

- Remember, these are the counting numbers: 1, 2, 3, and so on
-

- So these are the numbers 0, 1, 2, 3, and so on
- All natural numbers are also whole numbers
- So the box for natural numbers is completely contained in the box for whole numbers
-

- Integers are the numbers 0, 1, 2, 3, and so on, but also include -1, -2, -3 and so on with the negatives
- This means that all whole numbers and natural numbers are also integers
-

- Rational numbers are numbers that can be rewritten as fractions
- You can always rewrite an integer as itself over 1, so all integers are rational numbers
- This means all natural and whole numbers are rational numbers as well
-

- Numbers are either irrational or rational, but they cannot be both!
-
Remember, these are never-ending, non-repeating decimals, like
π and√ 2 - All of the numbers in the other categories so far were rational numbers, which means they are NOT irrational
-
- Real numbers include ALL rational and ALL irrational numbers
-

- So natural numbers are also whole numbers, integers, and rational numbers
- Whole numbers are also integers and rational numbers
- Integers are also rational numbers
- And all the numbers in the diagram are real numbers
-

- For example, not all whole numbers are natural numbers, since 0 is a whole number but not a natural number