www.VirtualNerd.com

How Do You Subtract Radicals with Unlike Radicands?

Subtract these radicals: √12 - √50 - √27 - √72

Summary

  1. Like radicals have the same radicand -- the number underneath the square root symbol
  2. We can simplify each square root by factoring out perfect squares
  3. Rewriting the perfect squares as something squared makes it easier to take the square root
  4. The Product Property of Square Roots lets you break up a square root into multiple square roots being multiplied together
  5. The square and square root are opposite operations, so they cancel each other out

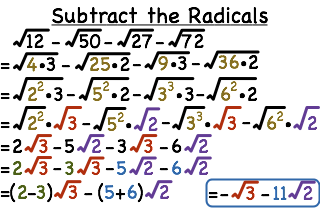
Notes

    1. We can only subtract like radicals
    2. Like radicals have the same radicand, or number underneath the square root symbol
    3. The symbol above 12, 50, 27, and 72 is a square root symbol
    4. A perfect square is a value that can be written as something squared
    1. A perfect square is a value that can be written as something squared
    1. A perfect square is a value that can be written as something squared
    2. The radicand is just the number under the square root symbol
    3. We can simplify the number under each square root by factoring out perfect squares
    1. 4 is a factor of 12 and it's a perfect square: it can be written as 22
    2. 25 is a factor of 50 and it's a perfect square: it can be written as 52
    3. 9 is a perfect square factor of 27
    4. 36 is a perfect square factor of 72
    1. Remember, a perfect square is a value that can be written as something squared
    2. Rewriting the perfect squares like this will make it easier to take the square root later
    1. Rewriting the perfect squares like this makes it easier to take the square root
    1. This property lets you break up a square root into multiple square roots being multiplied together
    1. This property lets you break up a square root into multiple square roots being multiplied together
    2. So for example we can rewrite √(22•3) as √(22)•√(3)
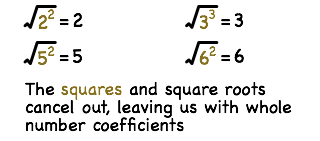
    1. The opposite of squaring is taking the square root
    2. Those operations cancel each other out!
    1. We now have some like radicals, so we can group them together and combine them
    1. Like radicals have the same radicand, the same number underneath the square root symbol
    2. We have two terms with a √(3) and two terms with a √(2)
    3. So we can group them next to each other and combine them, just like we do with variables
    1. Subtracting like radicals is just like subtracting like terms
    1. Subtracting like radicals is just like subtracting like terms
    2. Treat the like radicals as if they were like variables
    1. Neither 3 nor 2 have any perfect square factors, so they are as simplified as they can be