
www.VirtualNerd.com
How Do You Find the Length of a Leg of a Right Triangle?
Find the length of the missing side of the given triangle.
Summary
- The triangle's hypotenuse has a length of 20, and one leg has a length of 8
- The small box in the corner of the triangle represents a 90-degree, or right angle
- Since we have a right triangle, we can use the Pythagorean Theorem to solve for the missing length
- 'a', 'b', and 'c' represent the three sides of the triangle, where 'a' and 'b' are the legs and 'c' is the hypotenuse
- The symbols over the b2 and 336 are square roots
- Taking the square root of a squared number will get rid of the square
- The
≈ symbol means 'is approximately equal to'
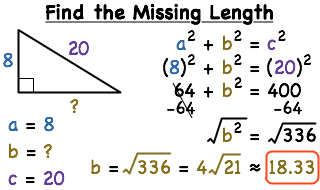
Notes
-

-

- 'a' and 'b' are the lengths of the legs: the two shorter sides of the right triangle
- 'c' is the length of the hypotenuse: the longest side of the right triangle
- Since we have a right triangle, we can use the Pythagorean Theorem to solve for the missing length
-

- Since we have a right triangle, we want to identify 'a', 'b', and 'c' and plug them into the Pythagorean Theorem
- 'a' and 'b' are the lengths of the legs: the two shorter sides of the right triangle
- 'c' is the length of the hypotenuse: the longest side of the right triangle
-

- The legs are the two shorter sides that come together to form the right angle
- It doesn't matter which leg we pick to be 'a' and which to be 'b'
-

- The hypotenuse is always across from the right angle in a right triangle
-

- We can plug 8 in for 'a' and 20 in for 'c' into the Pythagorean Theorem
- So we get 82 + b2 = 202
-

- Squaring 8 gives us 64 and squaring 20 gives us 400
- Subtracting 64 from both sides of 64 + b2 = 400 will get b2 by itself on the left side: b2 = 336
-

- Taking the square root of a squared number gets rid of the squared part
- The symbols over the b2 and 336 are square roots
- So the square root of b2 is just 'b'
-

-
Since 336 = 16•21,
√ (336) =√ (16•21) -
The Product Property of Square Roots lets us break
√ (16•21) into√ (16)•√ (21) -
Then we can simplify
√ (16) to 4 and rewrite√ (336) as 4√ (21) -
The
√ symbols represent square roots
-
Since 336 = 16•21,
-

- 4 times the square root of 21 is the most exact answer we can get for the length of 'b'
-
The
≈ symbol means 'is approximately equal to'