
www.VirtualNerd.com
How Do You Simplify Radicals Using the Product Property?
Write the √ 60 in its simplest form.
Summary
- The symbol above the 60 is a square root sign
- Writing out the prime factorization of 60 makes it easier to simplify the radical
- A prime number is a number whose only factors are 1 and itself
- Break up the problem into multiple radicals to make it easier to simplify
- Squaring and taking the square root are opposite operations, so they cancel each other out
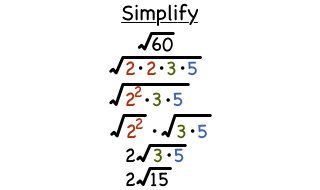
Notes
-

- Writing out the prime factorization of 60 will make it easier to simplify the radical
-

- A prime number is a number whose only factors are 1 and itself
-
2
• 2• 3• 5 is the prime factorization of 60
-

- Rewriting multiple factors using exponents makes it easier to see what can be taken out of the radical
-

-

- This property lets us break up a radical into the product of multiple radicals
-

- This property lets us break up a radical into the product of multiple radicals
-
So we can break
√ (22•3•5) into√ (22)•√ (3•5) - Breaking the radical up like this will make it easier to simplify
-

-

- A perfect square can be written as something squared
- Squaring and taking the square root are opposite operations
- They cancel each other out!
- Since neither 3 nor 5 are perfect squares, we can just multiply them back together to simplify
-

- The number under the radical, 15, doesn't have any perfect square factors
- That means our expression is in simplest form!