www.VirtualNerd.com

How was the Quadratic Formula Derived?

How was the Quadratic Formula derived?

Summary

  1. ax2+bx+c=0 is the general form of a quadratic equation
  2. To derive the Quadratic Formula, we will solve for 'x' by completing the square
  3. '±' means 'plus or minus' and indicates that the square root can be positive or negative

Notes

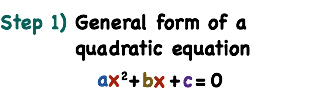
    1. ax2+bx+c=0 is the general form of a quadratic equation
    2. To derive the Quadratic Formula, we want to solve this equation for x
    3. This means we need to get x by itself on one side
    4. We can do this by completing the square
    1. We'll start by moving 'c' to the other side of the equals sign
    1. When we subtract 'c' from both sides, the two 'c's cancel on the left
    2. We're left with '-c' on the right
    1. In order to complete the square, we need the coefficient in front of x2 to be 1
    2. Since anything divided by itself is 1, if we divide both sides by 'a' we can turn that first coefficient into a 1
    1. Since anything divided by itself is 1, if we divide both sides by 'a' we can turn that first coefficient into a 1
    2. Make sure you divide by the same thing on both sides to preserve the equality!
    3. When we divide 'ax2' by 'a' the 'a's cancel, leaving us with 'x2'
    4. Dividing 'bx' by 'a' gives us (b/a)•x
    1. To complete the square, we need to turn the left hand side into a perfect square trinomial
    2. Then we will easily be able to factor
    3. To find the term we need to add to make a perfect square trinomial, we need to look at the coefficient in front of 'x'
    1. To find the value we need to add, we need to divide (b/a) by 2 and then square it
    2. Multiplying by 1/2 is the same as dividing by 2
    3. When we take a quotient to a power, we can take both the top and the bottom to that power
    1. We want to add the term we just found, b2/(4a2), to both sides of the equation
    2. This will give us our perfect square trinomial on the left!
    3. Remember, we need to add it to the right as well to maintain the equality
    1. To factor a perfect square trinomial, we can use the formula a2+2ab+b2=(a+b)2
    1. To factor a perfect square trinomial, we can use the formula a2+2ab+b2=(a+b)2
    2. Here we can use 'x2' as 'a2' and 'b2/(4a2)' as 'b2'
    3. Take the square root of both terms to find 'a' and 'b' for our formula
    4. The square root of 'x2' is 'x', so we can use that for 'a'
    5. The square root of 'b2/(4a2)' is 'b/(4a)', so we can use that for 'b'
    1. We want to find a common denominator so we can add the two fractions
    1. The least common denominator between these two fractions is '4a2'
    2. So we want to multiply (-c/a) by (4a)/(4a), which will give us a denominator of '4a2'
    3. Remember, anything divided by itself is just 1, so we're really just multiplying (-c/a) by 1
    4. We don't need to do anything to 'b2/(4a2)' because it already has the least common denominator
    1. In order to get 'x' by itself, we need to get rid of the square
    2. To do that, we can take the square root of both sides
    3. Remember, when you square either a positive or a negative number you end up with a positive
    4. So when you take the square root of something squared, you don't know whether it's going to be positive or negative
    5. So we put a plus or minus sign in front of the square root to account for both possibilities
    1. We don't want to have a fraction under a square root, so let's see if we can simplify any more
    1. The Quotient Property of Square Roots says we can break up the square root between the numerator and denominator of the fraction
    2. On the bottom, ±√(4a2) simplifies to '2a'
    1. Now all we have to do to get 'x' by itself is subtract 'b/(2a)' from both sides
    1. The 'b/(2a)'s cancel out on the left
    2. This leaves us with 'x' by itself on the left
    3. Remember, we need to subtract 'b/(2a)' on the right too!
    1. The two fractions on the right hand side have the same denominator
    2. So we can just subtract the numerators to simplify
    1. The two fractions on the right hand side have the same denominator
    2. So we can just subtract the numerators to simplify
    3. We want to put the '-b' before the square root, so we know that it's not a part of the square root
    4. Make sure you remember to keep the minus sign in front of the 'b' when you move it to the front!