
www.VirtualNerd.com
How Do You Graph the Parent Quadratic Function y=x2?
Graph y = x2.
Summary
- y = x2 is a quadratic function, which means its graph is a parabola
- Quadratic functions have the form y = ax2 + bx + c
- Since there is no coefficient in front of the x2, that means we have an invisible 1
- So our value for 'a' is 1
- Since we don't have an 'x' term or a constant, our values for 'b' and 'c' are 0
- The axis of symmetry is at x = 0, which is the y-axis
- The vertex is (0,0), which is the origin

Notes
-
- The axis of symmetry is the vertical line that cuts the parabola into two symmetrical halves
-

- The graph of a quadratic function is a parabola
-

- The axis of symmetry is the vertical line that cuts the parabola into two symmetrical halves
- Quadratic functions have the form y = ax2 + bx + c
- In the quadratic y = x2, a = 1 and b = 0
- So the axis of symmetry is at x = 0, which is the y-axis
-

- The vertex is where a quadratic function intersects the axis of symmetry
-

- The axis of symmetry is the vertical line that cuts the parabola into two symmetrical halves
- The axis of symmetry is at x = 0
-

- Since the vertex is on the axis of symmetry, which is the line x = 0, it has the same x-value
- The vertex is also on the parabola, so we can plug that x-value into our function to find the y-value for the vertex
-

- When we plug 0 in for 'x' into y = x2, we get y = 02, which is just 0
- So that means our y-value is 0 as well!
-

- Use a table to find points that are on the graph of y = x2
-

- The vertex is at the origin: the point (0,0)
- We want to choose some values for 'x' on either side of the vertex, so we can see what's happening on both sides of the parabola
- We're going to choose -2, -1, 0, 1, and 2 as the x-values
- Then we'll plug those values into y = x2 to find their corresponding y-values
-
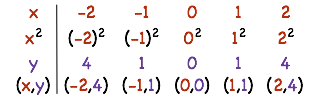
- We're choosing -2, -1, 0, 1, and 2 as the x-values
- Remember, the vertex is at the point (0,0), where x = 0
- Make sure to put parentheses around the negative numbers when you square them, so that you square the negative too!
- Simplify each square to find the values for 'y'
- Put the x's and y's together to make ordered pairs we can use to graph the parabola!
-

-

- The ordered pairs were:
- (-2,4), (-1,1), (0,0), (1,1), and (2,4)
-

- The parent function is the simplest form of a particular type of function
- In order to be a quadratic, a function needs to have an x2 term
- That's all the function y = x2 has -- you can't get any simpler than that and still be a quadratic!
- So y = x2 is the parent quadratic function